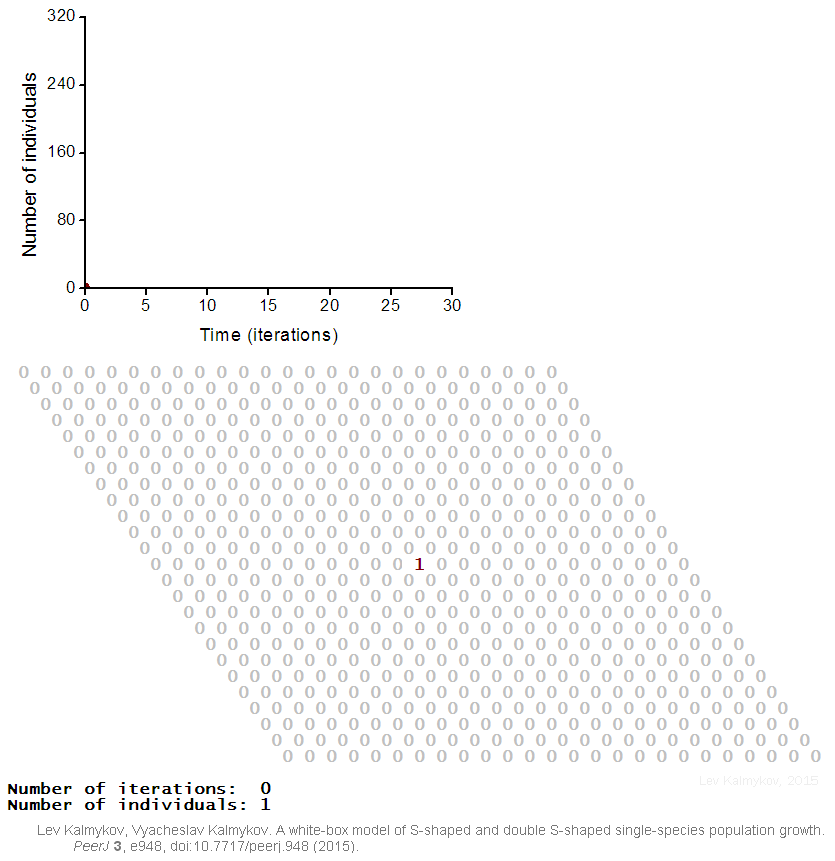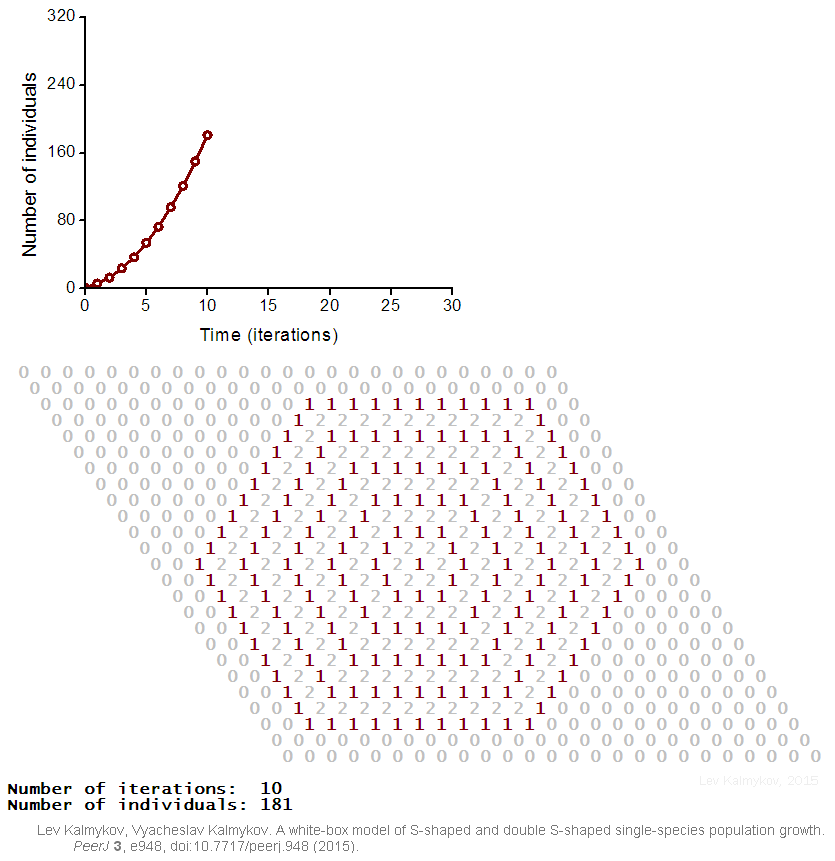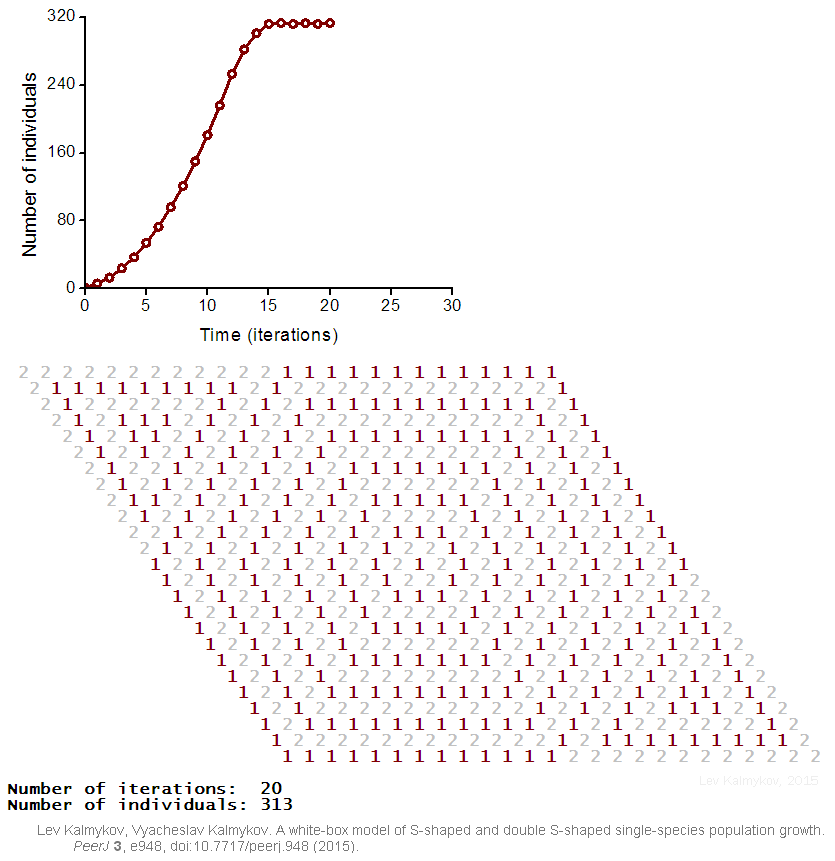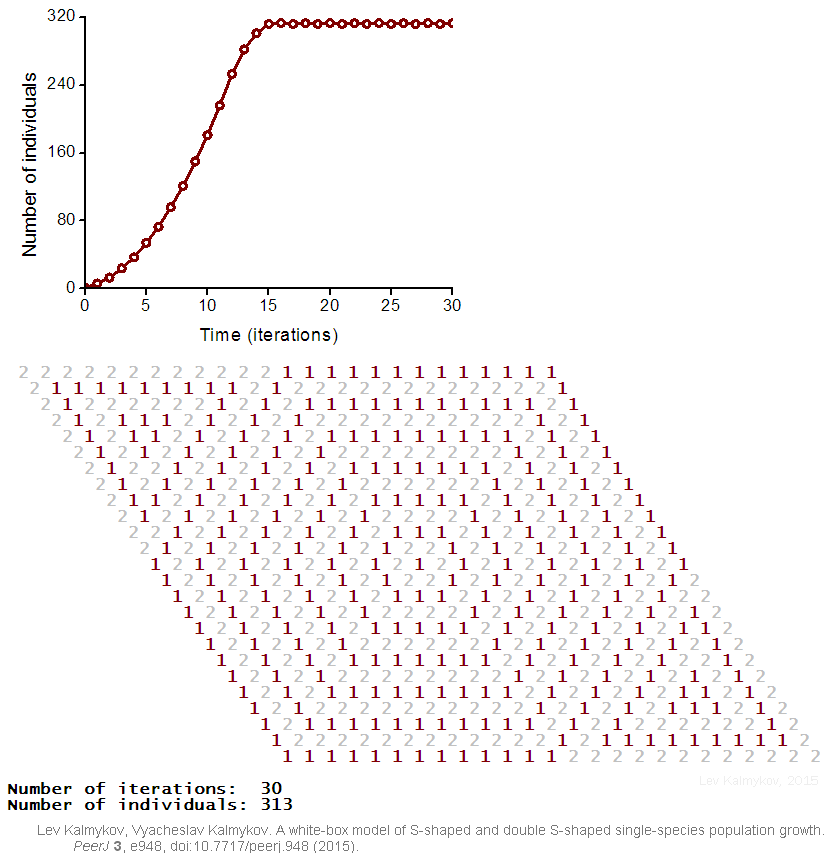
|
Population Modeling
A population model is a type of mathematical model that is applied to the study of population dynamics. Rationale Models allow a better understanding of how complex interactions and processes work. Modeling of dynamic interactions in nature can provide a manageable way of understanding how numbers change over time or in relation to each other. Many patterns can be noticed by using population modeling as a tool. Ecological population modeling is concerned with the changes in parameters such as population size and age distribution within a population. This might be due to interactions with the environment, individuals of their own species, or other species. Population models are used to determine maximum harvest for agriculturists, to understand the dynamics of biological invasions, and for environmental conservation. Population models are also used to understand the spread of parasites, viruses, and disease. Another way populations models are useful are when species become enda ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Mathematical Model
A mathematical model is a description of a system using mathematical concepts and language. The process of developing a mathematical model is termed mathematical modeling. Mathematical models are used in the natural sciences (such as physics, biology, earth science, chemistry) and engineering disciplines (such as computer science, electrical engineering), as well as in non-physical systems such as the social sciences (such as economics, psychology, sociology, political science). The use of mathematical models to solve problems in business or military operations is a large part of the field of operations research. Mathematical models are also used in music, linguistics, and philosophy (for example, intensively in analytic philosophy). A model may help to explain a system and to study the effects of different components, and to make predictions about behavior. Elements of a mathematical model Mathematical models can take many forms, including dynamical systems, statisti ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Moment Closure
In probability theory, moment closure is an approximation method used to estimate moments of a stochastic process. Introduction Typically, differential equations describing the ''i-''th moment will depend on the ''(i + 1)''-st moment. To use moment closure, a level is chosen past which all cumulants are set to zero. This leaves a resulting closed system of equations which can be solved for the moments. The approximation is particularly useful in models with a very large state space, such as stochastic population models. History The moment closure approximation was first used by Goodman and Whittle who set all third and higher-order cumulants to be zero, approximating the population distribution with a normal distribution In statistics, a normal distribution or Gaussian distribution is a type of continuous probability distribution for a real-valued random variable. The general form of its probability density function is : f(x) = \frac e^ The parameter \mu ... ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Population Ecology
Population ecology is a sub-field of ecology that deals with the dynamics of species populations and how these populations interact with the environment, such as birth and death rates, and by immigration and emigration. The discipline is important in conservation biology, especially in the development of population viability analysis which makes it possible to predict the long-term probability of a species persisting in a given patch of habitat. Although population ecology is a subfield of biology, it provides interesting problems for mathematicians and statisticians who work in population dynamics. History In the 1940s ecology was divided into autecology—the study of individual species in relation to the environment—and synecology—the study of groups of species in relation to the environment. The term autecology (from Ancient Greek: αὐτο, ''aúto'', "self"; οίκος, ''oíkos'', "household"; and λόγος, ''lógos'', "knowledge"), refers to roughly the same fie ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Population Dynamics Of Fisheries
A fishery is an area with an associated fish or aquatic population which is harvested for its commercial or recreational value. Fisheries can be wild or farmed. Population dynamics describes the ways in which a given population grows and shrinks over time, as controlled by birth, death, and migration. It is the basis for understanding changing fishery patterns and issues such as habitat destruction, predation and optimal harvesting rates. The population dynamics of fisheries is used by fisheries scientists to determine sustainable yields. The basic accounting relation for population dynamics is the BIDE (Birth, Immigration, Death, Emigration) model, shown as: : ''N''1 = ''N''0 + ''B'' − ''D'' + ''I'' − ''E'' where ''N''1 is the number of individuals at time 1, ''N''0 is the number of individuals at time 0, ''B'' is the number of individuals born, ''D'' the number that died, ''I'' the number that immigrated, and ''E'' the number that emigrated between time 0 and time ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Population Dynamics
Population dynamics is the type of mathematics used to model and study the size and age composition of populations as dynamical systems. History Population dynamics has traditionally been the dominant branch of mathematical biology, which has a history of more than 220 years,Malthus, Thomas Robert. An Essay on the Principle of Population: Library of Economics although over the last century the scope of mathematical biology has greatly expanded. The beginning of population dynamics is widely regarded as the work of Malthus, formulated as the Malthusian growth model. According to Malthus, assuming that the conditions (the environment) remain constant ('' ceteris paribus''), a population will grow (or decline) exponentially. This principle provided the basis for the subsequent predictive theories, such as the demographic studies such as the work of Benjamin Gompertz and Pierre François Verhulst in the early 19th century, who refined and adjusted the Malthusian demographic model. ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Logical Deterministic Individual-based Cellular Automata Model Of Interspecific Competition For A Single Limited Resource
Logic is the study of correct reasoning. It includes both formal and informal logic. Formal logic is the science of deductively valid inferences or of logical truths. It is a formal science investigating how conclusions follow from premises in a topic-neutral way. When used as a countable noun, the term "a logic" refers to a logical formal system that articulates a proof system. Formal logic contrasts with informal logic, which is associated with informal fallacies, critical thinking, and argumentation theory. While there is no general agreement on how formal and informal logic are to be distinguished, one prominent approach associates their difference with whether the studied arguments are expressed in formal or informal languages. Logic plays a central role in multiple fields, such as philosophy, mathematics, computer science, and linguistics. Logic studies arguments, which consist of a set of premises together with a conclusion. Premises and conclusions are usually under ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Logical Deterministic Individual-based Cellular Automata Model Of Single Species Population Growth
Logic is the study of correct reasoning. It includes both formal and informal logic. Formal logic is the science of deductively valid inferences or of logical truths. It is a formal science investigating how conclusions follow from premises in a topic-neutral way. When used as a countable noun, the term "a logic" refers to a logical formal system that articulates a proof system. Formal logic contrasts with informal logic, which is associated with informal fallacies, critical thinking, and argumentation theory. While there is no general agreement on how formal and informal logic are to be distinguished, one prominent approach associates their difference with whether the studied arguments are expressed in formal or informal languages. Logic plays a central role in multiple fields, such as philosophy, mathematics, computer science, and linguistics. Logic studies arguments, which consist of a set of premises together with a conclusion. Premises and conclusions are usually under ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Species–area Relationship
The species-area relationship or species-area curve describes the relationship between the area of a habitat, or of part of a habitat, and the number of species found within that area. Larger areas tend to contain larger numbers of species, and empirically, the relative numbers seem to follow systematic mathematical relationships.Preston, F.W. 1962. The canonical distribution of commonness and rarity: Part I. Ecology 43:185–215 and 410–432. The species-area relationship is usually constructed for a single type of organism, such as all vascular plants or all species of a specific trophic level within a particular site. It is rarely if ever, constructed for all types of organisms if simply because of the prodigious data requirements. It is related but not identical to the species discovery curve. Ecologists have proposed a wide range of factors determining the slope and elevation of the species-area relationship.Rosenzweig, M.L. 1995. Species Diversity in Space and Time. Cambrid ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Island Biogeography
Insular biogeography or island biogeography is a field within biogeography that examines the factors that affect the species richness and diversification of isolated natural communities. The theory was originally developed to explain the pattern of the species–area relationship occurring in oceanic islands. Under either name it is now used in reference to any ecosystem (present or past) that is isolated due to being surrounded by unlike ecosystems, and has been extended to mountain peaks, seamounts, oases, fragmented forests, and even natural habitats isolated by human land development. The field was started in the 1960s by the ecologists Robert H. MacArthur and E. O. Wilson, who coined the term ''island biogeography'' in their inaugural contribution to Princeton's Monograph in Population Biology series, which attempted to predict the number of species that would exist on a newly created island. Definitions For biogeographical purposes, an insular environment or "island" is ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Competitive Lotka–Volterra Equations
The competitive Lotka–Volterra equations are a simple model of the population dynamics of species competing for some common resource. They can be further generalised to the Generalized Lotka–Volterra equation to include trophic interactions. Overview The form is similar to the Lotka–Volterra equations for predation in that the equation for each species has one term for self-interaction and one term for the interaction with other species. In the equations for predation, the base population model is exponential. For the competition equations, the logistic equation is the basis. The logistic population model, when used by ecologists often takes the following form: = rx\left(1-\right). Here is the size of the population at a given time, is inherent per-capita growth rate, and is the carrying capacity. Two species Given two populations, and , with logistic dynamics, the Lotka–Volterra formulation adds an additional term to account for the species' interactions. Th ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Logistic Growth
A logistic function or logistic curve is a common S-shaped curve (sigmoid curve) with equation f(x) = \frac, where For values of x in the domain of real numbers from -\infty to +\infty, the S-curve shown on the right is obtained, with the graph of f approaching L as x approaches +\infty and approaching zero as x approaches -\infty. The logistic function finds applications in a range of fields, including biology (especially ecology Ecology () is the study of the relationships between living organisms, including humans, and their physical environment. Ecology considers organisms at the individual, population, community, ecosystem, and biosphere level. Ecology overlaps wi ...), biomathematics, chemistry, demography, economics, geoscience, mathematical psychology, probability, sociology, political science, linguistics, statistics, and artificial neural networks. A generalization of the logistic function is the hyperbolastic functions, hyperbolastic function of type I. ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
.png "Click for more on -> Island Biogeography")
