|
Plane Of Reference
In celestial mechanics, the plane of reference (or reference plane) is the plane used to define orbital elements (positions). The two main orbital elements that are measured with respect to the plane of reference are the inclination and the longitude of the ascending node. Depending on the type of body being described, there are four different kinds of reference planes that are typically used: *The ecliptic or invariable plane for planets, asteroids, comets, etc. within the Solar System, as these bodies generally have orbits that lie close to the ecliptic. *The equatorial plane of the orbited body for satellites orbiting with small semi-major axes *The local Laplace plane for satellites orbiting with intermediate-to-large semi-major axes *The plane tangent to celestial sphere for extrasolar objects On the plane of reference, a zero-point must be defined from which the angles of longitude are measured. This is usually defined as the point on the celestial sphere where the plane ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Celestial Mechanics
Celestial mechanics is the branch of astronomy that deals with the motions of objects in outer space. Historically, celestial mechanics applies principles of physics (classical mechanics) to astronomical objects, such as stars and planets, to produce ephemeris data. History Modern analytic celestial mechanics started with Isaac Newton's Principia of 1687. The name "celestial mechanics" is more recent than that. Newton wrote that the field should be called "rational mechanics." The term "dynamics" came in a little later with Gottfried Leibniz, and over a century after Newton, Pierre-Simon Laplace introduced the term "celestial mechanics." Prior to Kepler there was little connection between exact, quantitative prediction of planetary positions, using geometrical or arithmetical techniques, and contemporary discussions of the physical causes of the planets' motion. Johannes Kepler Johannes Kepler (1571–1630) was the first to closely integrate the predictive geom ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
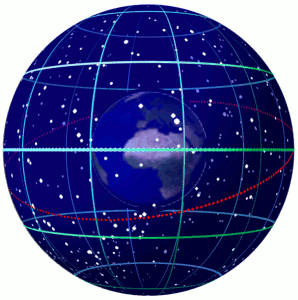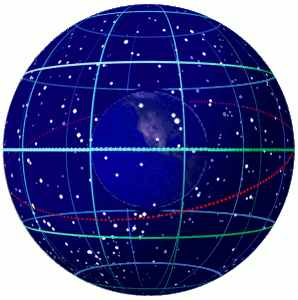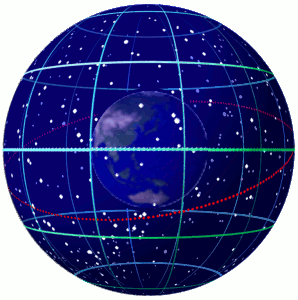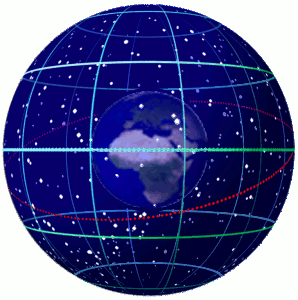
Celestial Sphere
In astronomy and navigation, the celestial sphere is an abstract sphere that has an arbitrarily large radius and is concentric to Earth. All objects in the sky can be conceived as being projected upon the inner surface of the celestial sphere, which may be centered on Earth or the observer. If centered on the observer, half of the sphere would resemble a hemispherical screen over the observing location. The celestial sphere is a conceptual tool used in spherical astronomy to specify the position of an object in the sky without consideration of its linear distance from the observer. The celestial equator divides the celestial sphere into northern and southern hemispheres. Introduction Because astronomical objects are at such remote distances, casual observation of the sky offers no information on their actual distances. All celestial objects seem equally far away, as if fixed onto the inside of a sphere with a large but unknown radius, which appears to rotate westward o ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Plane (geometry)
In mathematics, a plane is a Euclidean (flat), two-dimensional surface that extends indefinitely. A plane is the two-dimensional analogue of a point (zero dimensions), a line (one dimension) and three-dimensional space. Planes can arise as subspaces of some higher-dimensional space, as with one of a room's walls, infinitely extended, or they may enjoy an independent existence in their own right, as in the setting of two-dimensional Euclidean geometry. Sometimes the word ''plane'' is used more generally to describe a two-dimensional surface, for example the hyperbolic plane and elliptic plane. When working exclusively in two-dimensional Euclidean space, the definite article is used, so ''the'' plane refers to the whole space. Many fundamental tasks in mathematics, geometry, trigonometry, graph theory, and graphing are performed in a two-dimensional space, often in the plane. Euclidean geometry Euclid set forth the first great landmark of mathematical thought, an axiomatic ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Fundamental Plane (spherical Coordinates)
The fundamental plane in a spherical coordinate system is a plane of reference that divides the sphere into two hemispheres. The geocentric latitude of a point is then the angle between the fundamental plane and the line joining the point to the centre of the sphere. For a geographic coordinate system of the Earth, the fundamental plane is the Equator. Astronomical coordinate systems have varying fundamental planes: * The horizontal coordinate system uses the observer's horizon. * The Besselian coordinate system uses Earth's terminator (day/night boundary). This is a Cartesian coordinate system (''x'', ''y'', ''z''). * The equatorial coordinate system uses the celestial equator. * The ecliptic coordinate system uses the ecliptic. * The galactic coordinate system uses the Milky Way's galactic equator. See also *Plane of reference In celestial mechanics, the plane of reference (or reference plane) is the plane used to define orbital elements (positions). The two main orbital ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Equinox
A solar equinox is a moment in time when the Sun crosses the Earth's equator, which is to say, appears directly above the equator, rather than north or south of the equator. On the day of the equinox, the Sun appears to rise "due east" and set "due west". This occurs twice each year, around 20 March and 23 September. More precisely, an equinox is traditionally defined as the time when the plane of Earth's equator passes through the geometric center of the Sun's disk. Equivalently, this is the moment when Earth's rotation axis is directly perpendicular to the Sun-Earth line, tilting neither toward nor away from the Sun. In modern times, since the Moon (and to a lesser extent the planets) causes Earth's orbit to vary slightly from a perfect ellipse, the equinox is officially defined by the Sun's more regular ecliptic longitude rather than by its declination. The instants of the equinoxes are currently defined to be when the apparent geocentric longitude of the Sun is 0° a ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hour Circle
In astronomy, the hour circle, which together with declination and distance (from the planet's centre of mass) determines the location of any celestial object, is the great circle through the object and the two celestial poles. As such, it is a higher concept than the meridian as defined in astronomy, which takes account of the terrain and depth to the centre of Earth at a ground observer's location. The hour circles, specifically, are perfect circles perpendicular (at right angles) to the celestial equator. By contrast, the declination of an object viewed on the celestial sphere is the angle of that object to/from the celestial equator (thus ranging from +90° to −90°). The location of stars, planets, and other similarly distant objects is usually expressed in the following parameters, one for each of the three spatial dimensions: their declination, right ascension (epoch-fixed hour angle), and distance. These are as located at the vernal equinox for the epoch (e.g. J ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Longitude
Longitude (, ) is a geographic coordinate that specifies the east–west position of a point on the surface of the Earth, or another celestial body. It is an angular measurement, usually expressed in degrees and denoted by the Greek letter lambda (λ). Meridians are semicircular lines running from pole to pole that connect points with the same longitude. The prime meridian defines 0° longitude; by convention the International Reference Meridian for the Earth passes near the Royal Observatory in Greenwich, England on the island of Great Britain. Positive longitudes are east of the prime meridian, and negative ones are west. Because of the Earth's rotation, there is a close connection between longitude and time measurement. Scientifically precise local time varies with longitude: a difference of 15° longitude corresponds to a one-hour difference in local time, due to the differing position in relation to the Sun. Comparing local time to an absolute measure of time allows ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Laplace Plane
The Laplace plane or Laplacian plane of a planetary satellite, named after its discoverer Pierre-Simon Laplace (1749–1827), is a mean or reference plane about whose axis the instantaneous orbital plane of that satellite precesses. Laplace's name is sometimes applied to the invariable plane, which is the plane perpendicular to a system's mean angular momentum vector, but the two should not be confused. They are equivalent only in the case where all perturbers and resonances are far from the precessing body. Definition The axis of this Laplace plane is coplanar with, and between, (a) the polar axis of the parent planet's spin, and (b) the orbital axis of the parent planet's orbit around the Sun. The Laplace plane arises because the equatorial oblateness of the parent planet tends to cause the orbit of the satellite to precess around the polar axis of the parent planet's equatorial plane, while the solar perturbations tend to cause the orbit of the satellite to precess around the ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Orbital Elements
Orbital elements are the parameters required to uniquely identify a specific orbit. In celestial mechanics these elements are considered in two-body systems using a Kepler orbit. There are many different ways to mathematically describe the same orbit, but certain schemes, each consisting of a set of six parameters, are commonly used in astronomy and orbital mechanics. A real orbit and its elements change over time due to gravitational perturbations by other objects and the effects of general relativity. A Kepler orbit is an idealized, mathematical approximation of the orbit at a particular time. Keplerian elements The traditional orbital elements are the six Keplerian elements, after Johannes Kepler and his laws of planetary motion. When viewed from an inertial frame, two orbiting bodies trace out distinct trajectories. Each of these trajectories has its focus at the common center of mass. When viewed from a non-inertial frame centered on one of the bodies, only the traj ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Semi-major Axis
In geometry, the major axis of an ellipse is its longest diameter: a line segment that runs through the center and both foci, with ends at the two most widely separated points of the perimeter. The semi-major axis (major semiaxis) is the longest semidiameter or one half of the major axis, and thus runs from the centre, through a focus, and to the perimeter. The semi-minor axis (minor semiaxis) of an ellipse or hyperbola is a line segment that is at right angles with the semi-major axis and has one end at the center of the conic section. For the special case of a circle, the lengths of the semi-axes are both equal to the radius of the circle. The length of the semi-major axis of an ellipse is related to the semi-minor axis's length through the eccentricity and the semi-latus rectum \ell, as follows: The semi-major axis of a hyperbola is, depending on the convention, plus or minus one half of the distance between the two branches. Thus it is the distance from the center ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Equatorial Plane
The celestial equator is the great circle of the imaginary celestial sphere on the same plane as the equator of Earth. This plane of reference bases the equatorial coordinate system. In other words, the celestial equator is an abstract projection of the terrestrial equator into outer space. Due to Earth's axial tilt, the celestial equator is currently inclined by about 23.44° with respect to the ecliptic (the plane of Earth's orbit), but has varied from about 22.0° to 24.5° over the past 5 million years due to perturbation from other planets. An observer standing on Earth's equator visualizes the celestial equator as a semicircle passing through the zenith, the point directly overhead. As the observer moves north (or south), the celestial equator tilts towards the opposite horizon. The celestial equator is defined to be infinitely distant (since it is on the celestial sphere); thus, the ends of the semicircle always intersect the horizon due east and due west, regardless ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
