|
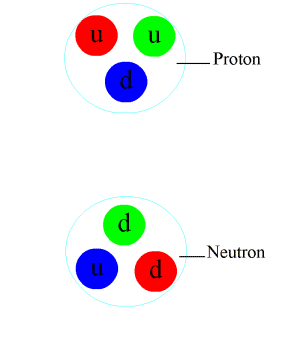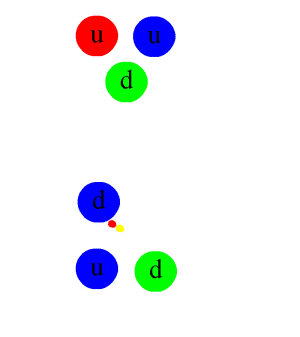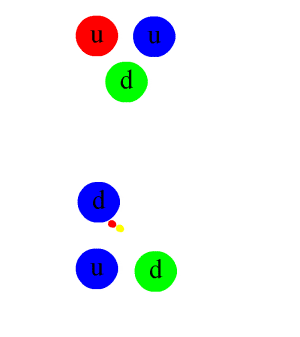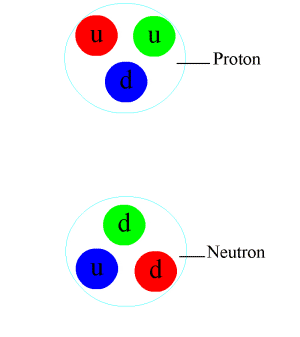
OZI Rule
The OZI rule is a consequence of quantum chromodynamics (QCD) that explains why certain decay modes appear less frequently than otherwise might be expected. It was independently proposed by Susumu Okubo, George Zweig and Jugoro Iizuka in the 1960s. It states that any strongly occurring process will be suppressed if, through only the removal of internal gluon lines, its Feynman diagram can be separated into two disconnected diagrams: one containing all of the initial-state particles and one containing all of the final-state particles. An example of such a suppressed decay is the Phi meson into pions: It would be expected that this decay mode would dominate over other decay modes such as which have much lower values. In actuality, it is seen that φ decays to kaons 84% of the time, suggesting the decay path to pions is suppressed. An explanation of the OZI rule can be seen from the decrease of the coupling constant in QCD with increasing energy (or momentum transfer). For t ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Phi Meson
In particle physics, the phi meson or meson is a vector meson formed of a strange quark and a strange antiquark. It was the meson's unusual propensity to decay into and that led to the discovery of the OZI rule. It has a mass of and a mean lifetime of . Properties The most common decay modes of the meson are at , at , and various indistinguishable combinations of s and pions at . In all cases, it decays via the strong force. The pion channel would naïvely be the dominant decay channel because the collective mass of the pions is smaller than that of the kaons, making it energetically favorable; however, it is suppressed by the OZI rule. The quark composition of the meson can be thought of as a mix between , , and states, but it is very nearly a pure state. This can be shown by deconstructing the wave function of the into its component parts. We see that the and mesons are mixtures of the SU(3) wave functions as follows. : \phi = \psi_8 \cos\theta - \psi_1 \sin\ ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Kaon
KAON (Karlsruhe ontology) is an ontology infrastructure developed by the University of Karlsruhe and the Research Center for Information Technologies in Karlsruhe. Its first incarnation was developed in 2002 and supported an enhanced version of RDF ontologies. Several tools like the graphical ontology editor OIModeler or the KAON Server were based on KAON. There are ontology learning companion tools which take non-annotated natural language text as input: TextToOnto (KAON-based) and Text2Onto (KAON2-based). Text2Onto is based on the Probabilistic Ontology Model (POM). In 2005, the first version of KAON2 was released, offering fast reasoning support for OWL ontologies. KAON2 is not backward-compatible with KAON. KAON2 is developed as a joint effort of the Information Process Engineering (IPE) at the Research Center for Information Technologies (FZI), the Institute of Applied Informatics and Formal Description Methods (AIFB) at the University of Karlsruhe, and the Informati ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
D Meson
The D mesons are the lightest particle containing charm quarks. They are often studied to gain knowledge on the weak interaction. The strange D mesons (Ds) were called "F mesons" prior to 1986. Overview The D mesons were discovered in 1976 by the Mark I detector at the Stanford Linear Accelerator Center. Since the D mesons are the lightest mesons containing a single charm quark (or antiquark), they must change the charm (anti)quark into an (anti)quark of another type to decay. Such transitions involve a change of the internal charm quantum number, and can take place only via the weak interaction. In D mesons, the charm quark preferentially changes into a strange quark via an exchange of a W particle, therefore the D meson preferentially decays into kaons () and pions (). List of D mesons ‡ PDG reports the resonance width ~\left(\Gamma\right)~. Here the conversion \; \tau = \frac \; is given instead. – oscillations In 2021 it was ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Charmonium
In particle physics, quarkonium (from quark and -onium, pl. quarkonia) is a flavorless meson whose constituents are a heavy quark and its own antiquark, making it both a neutral particle and its own antiparticle. Light quarks Light quarks ( up, down, and strange) are much less massive than the heavier quarks, and so the physical states actually seen in experiments ( η, η′, and π0 mesons) are quantum mechanical mixtures of the light quark states. The much larger mass differences between the charm and bottom quarks and the lighter quarks results in states that are well defined in terms of a quark–antiquark pair of a given flavor. Heavy quarks Examples of quarkonia are the J/ψ meson (the ground state of charmonium, ) and the meson (bottomonium, ). Because of the high mass of the top quark, toponium ( θ meson) does not exist, since the top quark decays through the electroweak interaction before a bound state can form (a rare example of a weak process proceeding ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Color Charge
Color charge is a property of quarks and gluons that is related to the particles' strong interactions in the theory of quantum chromodynamics (QCD). The "color charge" of quarks and gluons is completely unrelated to the everyday meanings of color and charge. The term ''color'' and the labels red, green, and blue became popular simply because of the loose analogy to the primary colors. Some particles have corresponding antiparticles. A particle with red, green, or blue charge has a corresponding antiparticle in which the color charge must be the anticolor of red, green, and blue, respectively, for the color charge to be conserved in particle–antiparticle creation and annihilation. Particle physicists call these antired, antigreen, and antiblue. All three colors mixed together, or any one of these colors and its complement (or negative), is "colorless" or "white" and has a net color charge of zero. Due to a property of the strong interaction called color confinement, free part ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
1/N Expansion
In quantum field theory and statistical mechanics, the 1/''N'' expansion (also known as the "large ''N''" expansion) is a particular perturbative analysis of quantum field theories with an internal symmetry group such as SO(N) or SU(N). It consists in deriving an expansion for the properties of the theory in powers of 1/N, which is treated as a small parameter. This technique is used in QCD (even though N is only 3 there) with the gauge group SU(3). Another application in particle physics is to the study of AdS/CFT dualities. It is also extensively used in condensed matter physics where it can be used to provide a rigorous basis for mean-field theory. Example Starting with a simple example — the O(N) φ4 — the scalar field φ takes on values in the real vector representation of O(N). Using the index notation for the N " flavors" with the Einstein summation convention and because O(N) is orthogonal, no distinction will be made between covariant and contravari ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Rest Mass
The invariant mass, rest mass, intrinsic mass, proper mass, or in the case of bound systems simply mass, is the portion of the total mass of an object or system of objects that is independent of the overall motion of the system. More precisely, it is a characteristic of the system's total energy and momentum that is the same in all frames of reference related by Lorentz transformations.Lawrence S. LernerPhysics for Scientists and Engineers, Volume 2, page 1073 1997. If a center-of-momentum frame exists for the system, then the invariant mass of a system is equal to its total mass in that "rest frame". In other reference frames, where the system's momentum is nonzero, the total mass (a.k.a. relativistic mass) of the system is greater than the invariant mass, but the invariant mass remains unchanged. Because of mass–energy equivalence, the rest energy of the system is simply the invariant mass times the speed of light squared. Similarly, the total energy of the system is its ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Momentum Transfer
In particle physics, wave mechanics and optics, momentum transfer is the amount of momentum that one particle gives to another particle. It is also called the scattering vector as it describes the transfer of wavevector in wave mechanics. In the simplest example of scattering of two colliding particles with initial momenta \vec_,\vec_, resulting in final momenta \vec_,\vec_, the momentum transfer is given by : \vec q = \vec_ - \vec_ = \vec_ - \vec_ where the last identity expresses momentum conservation. Momentum transfer is an important quantity because \Delta x = \hbar / , q, is a better measure for the typical distance resolution of the reaction than the momenta themselves. Wave mechanics and optics A wave has a momentum p = \hbar k and is a vectorial quantity. The difference of the momentum of the scattered wave to the incident wave is called ''momentum transfer''. The wave number k is the absolute of the wave vector k = p / \hbar and is related to the wavelength k = 2 ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Quantum Chromodynamics
In theoretical physics, quantum chromodynamics (QCD) is the theory of the strong interaction between quarks mediated by gluons. Quarks are fundamental particles that make up composite hadrons such as the proton, neutron and pion. QCD is a type of quantum field theory called a non-abelian gauge theory, with symmetry group SU(3). The QCD analog of electric charge is a property called ''color''. Gluons are the force carriers of the theory, just as photons are for the electromagnetic force in quantum electrodynamics. The theory is an important part of the Standard Model of particle physics. A large body of experimental evidence for QCD has been gathered over the years. QCD exhibits three salient properties: * Color confinement. Due to the force between two color charges remaining constant as they are separated, the energy grows until a quark–antiquark pair is spontaneously produced, turning the initial hadron into a pair of hadrons instead of isolating a color charge. Al ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Running Coupling
In physics, a coupling constant or gauge coupling parameter (or, more simply, a coupling), is a number that determines the strength of the force exerted in an interaction. Originally, the coupling constant related the force acting between two static bodies to the "charges" of the bodies (i.e. the electric charge for electrostatic and the mass for Newtonian gravity) divided by the distance squared, r^2, between the bodies; thus: G in F=G m_1 m_2/r^2 for Newtonian gravity and k_\text in F=k_\textq_1 q_2/r^2 for electrostatic. This description remains valid in modern physics for linear theories with static bodies and massless force carriers. A modern and more general definition uses the Lagrangian \mathcal (or equivalently the Hamiltonian \mathcal) of a system. Usually, \mathcal (or \mathcal) of a system describing an interaction can be separated into a ''kinetic part'' T and an ''interaction part'' V: \mathcal=T-V (or \mathcal=T+V). In field theory, V always contains 3 fields t ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Q Value (nuclear Science)
In nuclear physics and chemistry, the value for a reaction is the amount of energy absorbed or released during the nuclear reaction. The value relates to the enthalpy of a chemical reaction or the energy of radioactive decay products. It can be determined from the masses of reactants and products. values affect reaction rates. In general, the larger the positive value for the reaction, the faster the reaction proceeds, and the more likely the reaction is to "favor" the products. : Q = (\,m_\text - m_\text\,) \times \text where the masses are in atomic mass units. Also both \;m_\text\; and \;m_\text\; are the sums of the reactant and product masses respectively. Definition The conservation of energy, between the initial and final energy of a nuclear process \text E_\text = E_\text \text enables the general definition of based on the mass–energy equivalence. For any radioactive particle decay, the kinetic energy difference will be given by: : Q = K_\text - K_\text = (\ ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Pion
In particle physics, a pion (or a pi meson, denoted with the Greek letter pi: ) is any of three subatomic particles: , , and . Each pion consists of a quark and an antiquark and is therefore a meson. Pions are the lightest mesons and, more generally, the lightest hadrons. They are unstable, with the charged pions and decaying after a mean lifetime of 26.033 nanoseconds ( seconds), and the neutral pion decaying after a much shorter lifetime of 85 attoseconds ( seconds). Charged pions most often decay into muons and muon neutrinos, while neutral pions generally decay into gamma rays. The exchange of virtual pions, along with vector, rho and omega mesons, provides an explanation for the residual strong force between nucleons. Pions are not produced in radioactive decay, but commonly are in high-energy collisions between hadrons. Pions also result from some matter–antimatter annihilation events. All types of pions are also produced in natural pr ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |