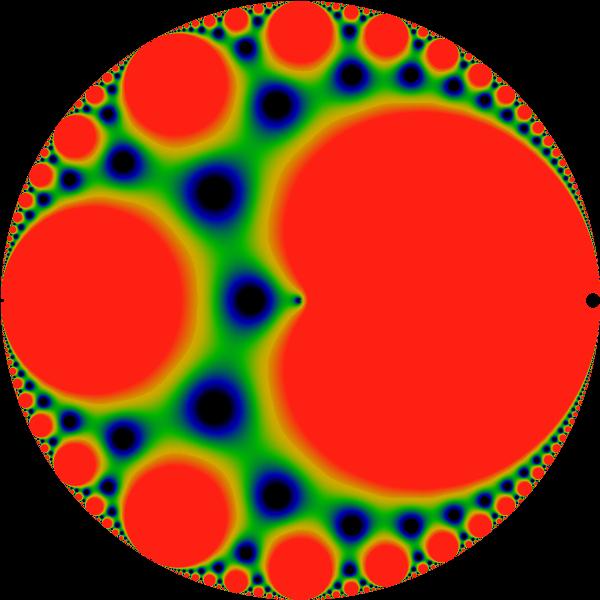
|
Mumford's Compactness Theorem
In mathematics, Mumford's compactness theorem states that the space of compact Riemann surfaces of fixed genus ''g'' > 1 with no closed geodesics of length less than some fixed ''ε'' > 0 in the Poincaré metric is compact. It was proved by as a consequence of a theorem about the compactness of sets of discrete subgroups of semisimple Lie groups generalizing Mahler's compactness theorem In mathematics, Mahler's compactness theorem, proved by , is a foundational result on lattices in Euclidean space, characterising sets of lattices that are 'bounded' in a certain definite sense. Looked at another way, it explains the ways in whic .... References * Riemann surfaces Kleinian groups Compactness theorems {{Riemannian-geometry-stub ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Compact Space
In mathematics, specifically general topology, compactness is a property that seeks to generalize the notion of a closed and bounded subset of Euclidean space by making precise the idea of a space having no "punctures" or "missing endpoints", i.e. that the space not exclude any ''limiting values'' of points. For example, the open interval (0,1) would not be compact because it excludes the limiting values of 0 and 1, whereas the closed interval ,1would be compact. Similarly, the space of rational numbers \mathbb is not compact, because it has infinitely many "punctures" corresponding to the irrational numbers, and the space of real numbers \mathbb is not compact either, because it excludes the two limiting values +\infty and -\infty. However, the ''extended'' real number line ''would'' be compact, since it contains both infinities. There are many ways to make this heuristic notion precise. These ways usually agree in a metric space, but may not be equivalent in other topologic ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Riemann Surface
In mathematics, particularly in complex analysis, a Riemann surface is a connected one-dimensional complex manifold. These surfaces were first studied by and are named after Bernhard Riemann. Riemann surfaces can be thought of as deformed versions of the complex plane: locally near every point they look like patches of the complex plane, but the global topology can be quite different. For example, they can look like a sphere or a torus or several sheets glued together. The main interest in Riemann surfaces is that holomorphic functions may be defined between them. Riemann surfaces are nowadays considered the natural setting for studying the global behavior of these functions, especially multi-valued functions such as the square root and other algebraic functions, or the logarithm. Every Riemann surface is a two-dimensional real analytic manifold (i.e., a surface), but it contains more structure (specifically a complex structure) which is needed for the unambiguous definitio ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Genus (mathematics)
In mathematics, genus (plural genera) has a few different, but closely related, meanings. Intuitively, the genus is the number of "holes" of a surface. A sphere has genus 0, while a torus has genus 1. Topology Orientable surfaces The genus of a connected, orientable surface is an integer representing the maximum number of cuttings along non-intersecting closed simple curves without rendering the resultant manifold disconnected. It is equal to the number of handles on it. Alternatively, it can be defined in terms of the Euler characteristic ''χ'', via the relationship ''χ'' = 2 − 2''g'' for closed surfaces, where ''g'' is the genus. For surfaces with ''b'' boundary components, the equation reads ''χ'' = 2 − 2''g'' − ''b''. In layman's terms, it's the number of "holes" an object has ("holes" interpreted in the sense of doughnut holes; a hollow sphere would be considered as having zero holes in this sense). A torus has 1 such h ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Closed Geodesic
In differential geometry and dynamical systems, a closed geodesic on a Riemannian manifold is a geodesic that returns to its starting point with the same tangent direction. It may be formalized as the projection of a closed orbit of the geodesic, geodesic flow on the tangent space of the manifold. Definition In a Riemannian manifold (''M'',''g''), a closed geodesic is a curve \gamma:\mathbb R\rightarrow M that is a geodesic for the metric ''g'' and is periodic. Closed geodesics can be characterized by means of a variational principle. Denoting by \Lambda M the space of smooth 1-periodic curves on ''M'', closed geodesics of period 1 are precisely the critical point (mathematics), critical points of the energy function E:\Lambda M\rightarrow\mathbb R, defined by : E(\gamma)=\int_0^1 g_(\dot\gamma(t),\dot\gamma(t))\,\mathrmt. If \gamma is a closed geodesic of period ''p'', the reparametrized curve t\mapsto\gamma(pt) is a closed geodesic of period 1, and therefore it is a critical poi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Poincaré Metric
In mathematics, the Poincaré metric, named after Henri Poincaré, is the metric tensor describing a two-dimensional surface of constant negative curvature. It is the natural metric commonly used in a variety of calculations in hyperbolic geometry or Riemann surfaces. There are three equivalent representations commonly used in two-dimensional hyperbolic geometry. One is the Poincaré half-plane model, defining a model of hyperbolic space on the upper half-plane. The Poincaré disk model defines a model for hyperbolic space on the unit disk. The disk and the upper half plane are related by a conformal map, and isometries are given by Möbius transformations. A third representation is on the punctured disk, where relations for ''q''-analogues are sometimes expressed. These various forms are reviewed below. Overview of metrics on Riemann surfaces A metric on the complex plane may be generally expressed in the form :ds^2=\lambda^2(z,\overline)\, dz\,d\overline where λ is ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Semisimple Lie Group
In mathematics, a Lie algebra is semisimple if it is a direct sum of modules, direct sum of simple Lie algebras. (A simple Lie algebra is a non-abelian Lie algebra without any non-zero proper Lie algebra#Subalgebras.2C ideals and homomorphisms, ideals). Throughout the article, unless otherwise stated, a Lie algebra is a finite-dimensional Lie algebra over a field of Characteristic (algebra), characteristic 0. For such a Lie algebra \mathfrak g, if nonzero, the following conditions are equivalent: *\mathfrak g is semisimple; *the Killing form, κ(x,y) = tr(ad(''x'')ad(''y'')), is non-degenerate; *\mathfrak g has no non-zero abelian ideals; *\mathfrak g has no non-zero solvable Lie algebra, solvable ideals; * the Radical of a Lie algebra, radical (maximal solvable ideal) of \mathfrak g is zero. Significance The significance of semisimplicity comes firstly from the Levi decomposition, which states that every finite dimensional Lie algebra is the semidirect product of a solvable i ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Mahler's Compactness Theorem
In mathematics, Mahler's compactness theorem, proved by , is a foundational result on lattices in Euclidean space, characterising sets of lattices that are 'bounded' in a certain definite sense. Looked at another way, it explains the ways in which a lattice could degenerate (''go off to infinity'') in a sequence of lattices. In intuitive terms it says that this is possible in just two ways: becoming ''coarse-grained'' with a fundamental domain that has ever larger volume; or containing shorter and shorter vectors. It is also called his selection theorem, following an older convention used in naming compactness theorems, because they were formulated in terms of sequential compactness (the possibility of selecting a convergent subsequence). Let ''X'' be the space :\mathrm_n(\mathbb)/\mathrm_n(\mathbb) that parametrises lattices in \mathbb^n, with its quotient topology. There is a well-defined function Δ on ''X'', which is the absolute value of the determinant of a matrix †... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Riemann Surfaces
In mathematics, particularly in complex analysis, a Riemann surface is a connected one-dimensional complex manifold. These surfaces were first studied by and are named after Bernhard Riemann. Riemann surfaces can be thought of as deformed versions of the complex plane: locally near every point they look like patches of the complex plane, but the global topology can be quite different. For example, they can look like a sphere or a torus or several sheets glued together. The main interest in Riemann surfaces is that holomorphic functions may be defined between them. Riemann surfaces are nowadays considered the natural setting for studying the global behavior of these functions, especially multi-valued functions such as the square root and other algebraic functions, or the logarithm. Every Riemann surface is a two-dimensional real analytic manifold (i.e., a surface), but it contains more structure (specifically a complex structure) which is needed for the unambiguous definition of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Kleinian Groups
In mathematics, a Kleinian group is a discrete subgroup of the group of orientation-preserving isometries of hyperbolic 3-space . The latter, identifiable with , is the quotient group of the 2 by 2 complex matrices of determinant 1 by their center, which consists of the identity matrix and its product by . has a natural representation as orientation-preserving conformal transformations of the Riemann sphere, and as orientation-preserving conformal transformations of the open unit ball in . The group of Möbius transformations is also related as the non-orientation-preserving isometry group of , . So, a Kleinian group can be regarded as a discrete subgroup acting on one of these spaces. History The theory of general Kleinian groups was founded by and , who named them after Felix Klein. The special case of Schottky groups had been studied a few years earlier, in 1877, by Schottky. Definitions One modern definition of Kleinian group is as a group which acts on the 3-ball B^3 ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
