|
Minkowski's Question Mark Function
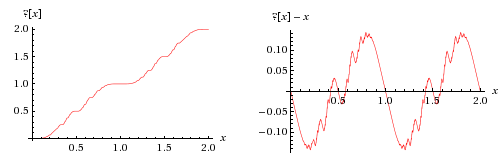
In mathematics, the Minkowski question-mark function, denoted , is a function with unusual fractal properties, defined by Hermann Minkowski in 1904. It maps quadratic irrational numbers to rational numbers on the unit interval, via an expression relating the continued fraction expansions of the quadratics to the binary expansions of the rationals, given by Arnaud Denjoy in 1938. It also maps rational numbers to dyadic rationals, as can be seen by a recursive definition closely related to the Stern–Brocot tree. Definition and intuition One way to define the question-mark function involves the correspondence between two different ways of representing fractional numbers using finite or infinite binary sequences. Most familiarly, a string of 0's and 1's with a single point mark ".", like "11.001001000011111..." can be interpreted as the binary representation of a number. In this case this number is 2+1+\frac18+\frac1+\cdots=\pi. There is a different way of interpreting the sam ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Minkowski Question Mark
In mathematics, the Minkowski question-mark function, denoted , is a Function (mathematics), function with unusual fractal properties, defined by Hermann Minkowski in 1904. It maps quadratic irrational numbers to rational numbers on the unit interval, via an expression relating the continued fraction expansions of the quadratics to the binary expansions of the rationals, given by Arnaud Denjoy in 1938. It also maps rational numbers to dyadic rationals, as can be seen by a recursive definition closely related to the Stern–Brocot tree. Definition and intuition One way to define the question-mark function involves the correspondence between two different ways of representing fractional numbers using finite or infinite binary sequences. Most familiarly, a string of 0's and 1's with a single point mark ".", like "11.001001000011111..." can be interpreted as the binary representation of a number. In this case this number is 2+1+\frac18+\frac1+\cdots=\pi. There is a different way of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Real Number
In mathematics, a real number is a number that can be used to measurement, measure a ''continuous'' one-dimensional quantity such as a distance, time, duration or temperature. Here, ''continuous'' means that values can have arbitrarily small variations. Every real number can be almost uniquely represented by an infinite decimal expansion. The real numbers are fundamental in calculus (and more generally in all mathematics), in particular by their role in the classical definitions of limit (mathematics), limits, continuous function, continuity and derivatives. The set of real numbers is mathematical notation, denoted or \mathbb and is sometimes called "the reals". The adjective ''real'' in this context was introduced in the 17th century by René Descartes to distinguish real numbers, associated with physical reality, from imaginary numbers (such as the square roots of ), which seemed like a theoretical contrivance unrelated to physical reality. The real numbers subset, include t ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Periodic Orbit
In mathematics, in the study of iterated functions and dynamical systems, a periodic point of a function is a point which the system returns to after a certain number of function iterations or a certain amount of time. Iterated functions Given a mapping ''f'' from a set ''X'' into itself, :f: X \to X, a point ''x'' in ''X'' is called periodic point if there exists an ''n'' so that :\ f_n(x) = x where f_n is the ''n''th iterate of ''f''. The smallest positive integer ''n'' satisfying the above is called the ''prime period'' or ''least period'' of the point ''x''. If every point in ''X'' is a periodic point with the same period ''n'', then ''f'' is called ''periodic'' with period ''n'' (this is not to be confused with the notion of a periodic function). If there exist distinct ''n'' and ''m'' such that :f_n(x) = f_m(x) then ''x'' is called a preperiodic point. All periodic points are preperiodic. If ''f'' is a diffeomorphism of a differentiable manifold, so that the derivative ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Modular Group
In mathematics, the modular group is the projective special linear group of matrices with integer coefficients and determinant 1. The matrices and are identified. The modular group acts on the upper-half of the complex plane by fractional linear transformations, and the name "modular group" comes from the relation to moduli spaces and not from modular arithmetic. Definition The modular group is the group of linear fractional transformations of the upper half of the complex plane, which have the form :z\mapsto\frac, where , , , are integers, and . The group operation is function composition. This group of transformations is isomorphic to the projective special linear group , which is the quotient of the 2-dimensional special linear group over the integers by its center . In other words, consists of all matrices :\begin a & b \\ c & d \end where , , , are integers, , and pairs of matrices and are considered to be identical. The group operation is the us ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Linear Fractional Transformation
In mathematics, a linear fractional transformation is, roughly speaking, a transformation of the form :z \mapsto \frac , which has an inverse. The precise definition depends on the nature of , and . In other words, a linear fractional transformation is a ''transformation'' that is represented by a ''fraction'' whose numerator and denominator are ''linear''. In the most basic setting, , and are complex numbers (in which case the transformation is also called a Möbius transformation), or more generally elements of a field. The invertibility condition is then . Over a field, a linear fractional transformation is the restriction to the field of a projective transformation or homography of the projective line. When are integer (or, more generally, belong to an integral domain), is supposed to be a rational number (or to belong to the field of fractions of the integral domain. In this case, the invertibility condition is that must be a unit of the domain (that is or in the ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
De Rham Curve
In mathematics, a de Rham curve is a certain type of fractal curve named in honor of Georges de Rham. The Cantor function, Cesàro curve, Minkowski's question mark function, the Lévy C curve, the blancmange curve, and Koch curve are all special cases of the general de Rham curve. Construction Consider some complete metric space (M,d) (generally \mathbb2 with the usual euclidean distance), and a pair of contracting maps on M: :d_0:\ M \to M :d_1:\ M \to M. By the Banach fixed-point theorem, these have fixed points p_0 and p_1 respectively. Let ''x'' be a real number in the interval ,1/math>, having binary expansion :x = \sum_^\infty \frac, where each b_k is 0 or 1. Consider the map :c_x:\ M \to M defined by :c_x = d_ \circ d_ \circ \cdots \circ d_ \circ \cdots, where \circ denotes function composition. It can be shown that each c_x will map the common basin of attraction of d_0 and d_1 to a single point p_x in M. The collection of points p_x, parameterized by ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Period-doubling Monoid
In mathematics, a de Rham curve is a certain type of fractal curve named in honor of Georges de Rham. The Cantor function, Cesàro curve, Minkowski's question mark function, the Lévy C curve, the blancmange curve, and Koch curve are all special cases of the general de Rham curve. Construction Consider some complete metric space (M,d) (generally \mathbb2 with the usual euclidean distance), and a pair of contracting maps on M: :d_0:\ M \to M :d_1:\ M \to M. By the Banach fixed-point theorem, these have fixed points p_0 and p_1 respectively. Let ''x'' be a real number in the interval ,1/math>, having binary expansion :x = \sum_^\infty \frac, where each b_k is 0 or 1. Consider the map :c_x:\ M \to M defined by :c_x = d_ \circ d_ \circ \cdots \circ d_ \circ \cdots, where \circ denotes function composition. It can be shown that each c_x will map the common basin of attraction of d_0 and d_1 to a single point p_x in M. The collection of points p_x, parameterized by a sing ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Self-similarity
__NOTOC__ In mathematics, a self-similar object is exactly or approximately similar to a part of itself (i.e., the whole has the same shape as one or more of the parts). Many objects in the real world, such as coastlines, are statistically self-similar: parts of them show the same statistical properties at many scales. Self-similarity is a typical property of fractals. Scale invariance is an exact form of self-similarity where at any magnification there is a smaller piece of the object that is similar to the whole. For instance, a side of the Koch snowflake is both symmetrical and scale-invariant; it can be continually magnified 3x without changing shape. The non-trivial similarity evident in fractals is distinguished by their fine structure, or detail on arbitrarily small scales. As a counterexample, whereas any portion of a straight line may resemble the whole, further detail is not revealed. A time developing phenomenon is said to exhibit self-similarity if the numerica ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Function Graph
In mathematics, the graph of a function f is the set of ordered pairs (x, y), where f(x) = y. In the common case where x and f(x) are real numbers, these pairs are Cartesian coordinates of points in two-dimensional space and thus form a subset of this plane. In the case of functions of two variables, that is functions whose domain consists of pairs (x, y), the graph usually refers to the set of ordered triples (x, y, z) where f(x,y) = z, instead of the pairs ((x, y), z) as in the definition above. This set is a subset of three-dimensional space; for a continuous real-valued function of two real variables, it is a surface. In science, engineering, technology, finance, and other areas, graphs are tools used for many purposes. In the simplest case one variable is plotted as a function of another, typically using rectangular axes; see ''Plot (graphics)'' for details. A graph of a function is a special case of a relation. In the modern foundations of mathematics, and, typicall ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Point Reflection
In geometry, a point reflection (point inversion, central inversion, or inversion through a point) is a type of isometry of Euclidean space. An object that is invariant under a point reflection is said to possess point symmetry; if it is invariant under point reflection through its center, it is said to possess central symmetry or to be centrally symmetric. Point reflection can be classified as an affine transformation. Namely, it is an isometric involutive affine transformation, which has exactly one fixed point, which is the point of inversion. It is equivalent to a homothetic transformation with scale factor equal to −1. The point of inversion is also called homothetic center. Terminology The term ''reflection'' is loose, and considered by some an abuse of language, with ''inversion'' preferred; however, ''point reflection'' is widely used. Such maps are involutions, meaning that they have order 2 – they are their own inverse: applying them twice yields the identi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Monoid
In abstract algebra, a branch of mathematics, a monoid is a set equipped with an associative binary operation and an identity element. For example, the nonnegative integers with addition form a monoid, the identity element being 0. Monoids are semigroups with identity. Such algebraic structures occur in several branches of mathematics. The functions from a set into itself form a monoid with respect to function composition. More generally, in category theory, the morphisms of an object to itself form a monoid, and, conversely, a monoid may be viewed as a category with a single object. In computer science and computer programming, the set of strings built from a given set of characters is a free monoid. Transition monoids and syntactic monoids are used in describing finite-state machines. Trace monoids and history monoids provide a foundation for process calculi and concurrent computing. In theoretical computer science, the study of monoids is fundamental for ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Ternary Numeral System
A ternary numeral system (also called base 3 or trinary) has three as its base. Analogous to a bit, a ternary digit is a trit (trinary digit). One trit is equivalent to log2 3 (about 1.58496) bits of information. Although ''ternary'' most often refers to a system in which the three digits are all non–negative numbers; specifically , , and , the adjective also lends its name to the balanced ternary system; comprising the digits −1, 0 and +1, used in comparison logic and ternary computers. Comparison to other bases Representations of integer numbers in ternary do not get uncomfortably lengthy as quickly as in binary. For example, decimal 365 or senary 1405 corresponds to binary 101101101 (nine digits) and to ternary 111112 (six digits). However, they are still far less compact than the corresponding representations in bases such as decimalsee below for a compact way to codify ternary using nonary (base 9) and septemvigesimal (base 27). As for rational num ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |