|
Major General's Song
"I Am the Very Model of a Modern Major-General" (often referred to as the "Major-General's Song" or "Modern Major-General's Song") is a patter song from Gilbert and Sullivan's 1879 comic opera ''The Pirates of Penzance''. It has been called the most famous Gilbert and Sullivan patter song. Sung by Major-general (United Kingdom), Major-General Stanley at his first entrance, towards the end of Act I, the character introduces himself by presenting his résumé and admitting to a few shortcomings. The song satirises the idea of the "modern" educated British Army officer of the latter 19th century. The song is replete with historical and cultural references, in which the Major-General describes his impressive and well-rounded education in non-military matters, but he says that his military knowledge has "only been brought down to the beginning of the century". The stage directions in the libretto state that at the end of each verse the Major-General is "bothered for a rhyme". Interpo ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
The Fifteen Decisive Battles Of The World
''The Fifteen Decisive Battles of the World: from Marathon to Waterloo'' is a book written by Sir Edward Shepherd Creasy and published in 1851. This book tells the story of the fifteen military engagements which, according to the author, had a significant impact on world history. Chapters Each chapter of the book describes a different battle. The fifteen chapters are: # The Battle of Marathon, 490 BC #* Excerpt: "Two thousand three hundred and forty years ago, a council of Athenian Officers was summoned on the slope of one of the mountains that look over the plain of Marathon, on the eastern coast of Attica. The immediate subject of their meeting was to consider whether they should give battle to an enemy that lay encamped on the shore beneath them; but on the result of their deliberations depended, not merely the fate of two armies, but the whole future progress of human civilization." # Defeat of the Athenians at Syracuse, 413 BC #* Known as the Battle of Syracus ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Caradoc
Caradoc Vreichvras (; Modern cy, Caradog Freichfras, ) was a semi-legendary ancestor to the kings of Gwent. He may have lived during the 5th or 6th century. He is remembered in the Matter of Britain as a Knight of the Round Table, under the names King Carados and Carados Briefbras (French for "Carados Shortarm"). Identification and historicity Though the name "Caradoc" and its various forms were by no means uncommon during the Middle Ages, it is probable some of the Caradocs referred to in Welsh genealogies and hagiographies such the ''Life of St. Tatheus'' are the same person. Due to the name's prevalence considerable confusion exists about Caradoc's identity, both historical and literary. He may have become confused with the British hero Caratacus (the Latin form of Caradoc), Cerdic of Wessex and any number of British history's later Caradocs. His parentage varies from text to text; he is called the son of Llŷr Marini (possibly implying Llŷr) several times in the '' Mabin ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
King Arthur
King Arthur ( cy, Brenin Arthur, kw, Arthur Gernow, br, Roue Arzhur) is a legendary king of Britain, and a central figure in the medieval literary tradition known as the Matter of Britain. In the earliest traditions, Arthur appears as a leader of the post-Roman Britons in battles against Saxon invaders of Britain in the late 5th and early 6th centuries. He appears in two early medieval historical sources, the ''Annales Cambriae'' and the ''Historia Brittonum'', but these date to 300 years after he is supposed to have lived, and most historians who study the period do not consider him a historical figure.Tom Shippey, "So Much Smoke", ''review'' of , ''London Review of Books'', 40:24:23 (20 December 2018) His name also occurs in early Welsh poetic sources such as ''Y Gododdin''. The character developed through Welsh mythology, appearing either as a great warrior defending Britain from human and supernatural enemies or as a magical figure of folklore, sometimes associated wi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Animalcule
Animalcule ('little animal', from Latin ''animal'' + the diminutive suffix ''-culum'') is an old term for microscopic organisms that included bacteria, protozoans, and very small animals. The word was invented by 17th-century Dutch scientist Antonie van Leeuwenhoek to refer to the microorganisms he observed in rainwater. Some better-known types of animalcule include: * '' Actinophrys'', and other heliozoa, termed sun animalcules. * ''Amoeba'', termed ''Proteus'' animalcules. * ''Noctiluca scintillans'', commonly termed the sea sparkles. * ''Paramecium'', termed slipper animalcules. * ''Rotifers'', termed wheel animalcules. * ''Stentor'', termed trumpet animalcules. * ''Vorticella'', and other peritrichs, termed bell animalcules. The concept seems to have been proposed at least as early as about 30 BC, as evidenced by this translation from Marcus Varro's ''Rerum Rusticarum Libri Tres'': Note also if there be any swampy ground, both for the reasons given above, and because certa ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Binomial Nomenclature
In taxonomy, binomial nomenclature ("two-term naming system"), also called nomenclature ("two-name naming system") or binary nomenclature, is a formal system of naming species of living things by giving each a name composed of two parts, both of which use Latin grammatical forms, although they can be based on words from other languages. Such a name is called a binomial name (which may be shortened to just "binomial"), a binomen, name or a scientific name; more informally it is also historically called a Latin name. The first part of the name – the '' generic name'' – identifies the genus to which the species belongs, whereas the second part – the specific name or specific epithet – distinguishes the species within the genus. For example, modern humans belong to the genus ''Homo'' and within this genus to the species ''Homo sapiens''. ''Tyrannosaurus rex'' is likely the most widely known binomial. The ''formal'' introduction of this system of naming species is credit ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Differential Calculus
In mathematics, differential calculus is a subfield of calculus that studies the rates at which quantities change. It is one of the two traditional divisions of calculus, the other being integral calculus—the study of the area beneath a curve. The primary objects of study in differential calculus are the derivative of a function, related notions such as the differential, and their applications. The derivative of a function at a chosen input value describes the rate of change of the function near that input value. The process of finding a derivative is called differentiation. Geometrically, the derivative at a point is the slope of the tangent line to the graph of the function at that point, provided that the derivative exists and is defined at that point. For a real-valued function of a single real variable, the derivative of a function at a point generally determines the best linear approximation to the function at that point. Differential calculus and integral calculus are ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Integral Calculus
In mathematics, an integral assigns numbers to Function (mathematics), functions in a way that describes Displacement (geometry), displacement, area, volume, and other concepts that arise by combining infinitesimal data. The process of finding integrals is called integration. Along with Derivative, differentiation, integration is a fundamental, essential operation of calculus,Integral calculus is a very well established mathematical discipline for which there are many sources. See and , for example. and serves as a tool to solve problems in mathematics and physics involving the area of an arbitrary shape, the length of a curve, and the volume of a solid, among others. The integrals enumerated here are those termed definite integrals, which can be interpreted as the signed area of the region in the plane that is bounded by the Graph of a function, graph of a given function between two points in the real line. Conventionally, areas above the horizontal axis of the plane are posi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
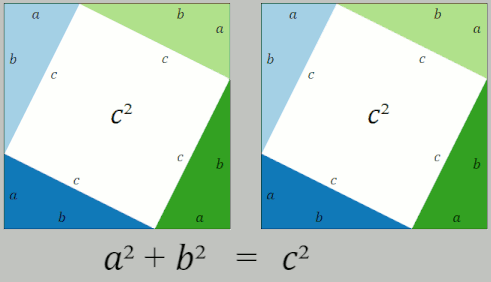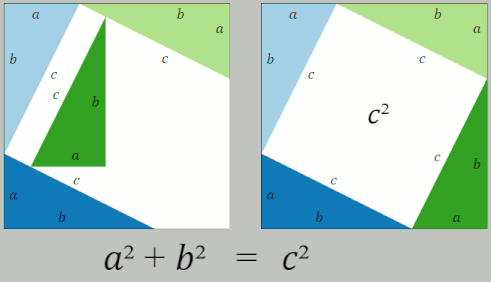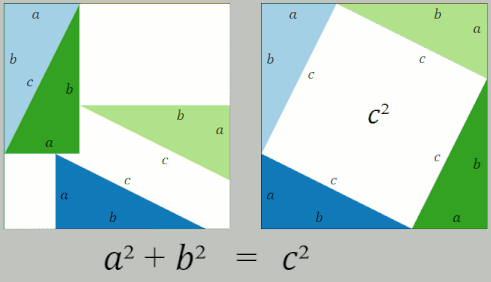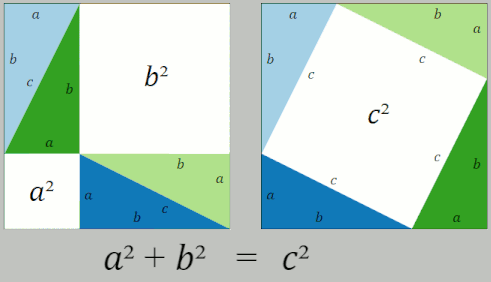
Pythagorean Theorem
In mathematics, the Pythagorean theorem or Pythagoras' theorem is a fundamental relation in Euclidean geometry between the three sides of a right triangle. It states that the area of the square whose side is the hypotenuse (the side opposite the right angle) is equal to the sum of the areas of the squares on the other two sides. This theorem can be written as an equation relating the lengths of the sides ''a'', ''b'' and the hypotenuse ''c'', often called the Pythagorean equation: :a^2 + b^2 = c^2 , The theorem is named for the Greek philosopher Pythagoras, born around 570 BC. The theorem has been proven numerous times by many different methods – possibly the most for any mathematical theorem. The proofs are diverse, including both geometric proofs and algebraic proofs, with some dating back thousands of years. When Euclidean space is represented by a Cartesian coordinate system in analytic geometry, Euclidean distance satisfies the Pythagorean relation: the squared dist ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Binomial Theorem
In elementary algebra, the binomial theorem (or binomial expansion) describes the algebraic expansion of powers of a binomial. According to the theorem, it is possible to expand the polynomial into a sum involving terms of the form , where the exponents and are nonnegative integers with , and the coefficient of each term is a specific positive integer depending on and . For example, for , (x+y)^4 = x^4 + 4 x^3y + 6 x^2 y^2 + 4 x y^3 + y^4. The coefficient in the term of is known as the binomial coefficient \tbinom or \tbinom (the two have the same value). These coefficients for varying and can be arranged to form Pascal's triangle. These numbers also occur in combinatorics, where \tbinom gives the number of different combinations of elements that can be chosen from an -element set. Therefore \tbinom is often pronounced as " choose ". History Special cases of the binomial theorem were known since at least the 4th century BC when Greek mathematician Euclid ment ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Quadratic Equation
In algebra, a quadratic equation () is any equation that can be rearranged in standard form as ax^2 + bx + c = 0\,, where represents an unknown (mathematics), unknown value, and , , and represent known numbers, where . (If and then the equation is linear equation, linear, not quadratic.) The numbers , , and are the ''coefficients'' of the equation and may be distinguished by respectively calling them, the ''quadratic coefficient'', the ''linear coefficient'' and the ''constant'' or ''free term''. The values of that satisfy the equation are called ''solution (mathematics), solutions'' of the equation, and ''zero of a function, roots'' or ''zero of a function, zeros'' of the Expression (mathematics), expression on its left-hand side. A quadratic equation has at most two solutions. If there is only one solution, one says that it is a double root. If all the coefficients are real numbers, there are either two real solutions, or a single real double root, or two complex number, c ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Linear Equation
In mathematics, a linear equation is an equation that may be put in the form a_1x_1+\ldots+a_nx_n+b=0, where x_1,\ldots,x_n are the variables (or unknowns), and b,a_1,\ldots,a_n are the coefficients, which are often real numbers. The coefficients may be considered as parameters of the equation, and may be arbitrary expressions, provided they do not contain any of the variables. To yield a meaningful equation, the coefficients a_1, \ldots, a_n are required to not all be zero. Alternatively, a linear equation can be obtained by equating to zero a linear polynomial over some field, from which the coefficients are taken. The solutions of such an equation are the values that, when substituted for the unknowns, make the equality true. In the case of just one variable, there is exactly one solution (provided that a_1\ne 0). Often, the term ''linear equation'' refers implicitly to this particular case, in which the variable is sensibly called the ''unknown''. In the case of two vari ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |