|
Magic Circle (mathematics)
Magic circles were invented by the Song dynasty (960–1279) Chinese mathematician Yang Hui (c. 1238–1298). It is the arrangement of natural numbers on circles where the sum of the numbers on each circle and the sum of numbers on diameters are identical. One of his magic circles was constructed from the natural numbers from 1 to 33 arranged on four concentric circles, with 9 at the center. Yang Hui magic circles Yang Hui's magic circle series was published in his ''Xugu Zhaiqi Suanfa''《續古摘奇算法》(Sequel to Excerpts of Mathematical Wonders) of 1275. His magic circle series includes: magic 5 circles in square, 6 circles in ring, magic eight circle in square magic concentric circles, magic 9 circles in square. Yang Hui magic concentric circle Yang Hui's magic concentric circle has the following properties *The sum of the numbers on four diameters = 147, ** 28 + 5 + 11 + 25 + 9 + 7 + 19& ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Yang Hui Magic Circle
Yang may refer to: * Yang, in yin and yang, one half of the two symbolic polarities in Chinese philosophy * Korean yang, former unit of currency of Korea from 1892 to 1902 * YANG, a data modeling language for the NETCONF network configuration protocol Geography * Yang County, in Shaanxi, China * Yangzhou (ancient China), also known as Yang Prefecture * Yang (state), ancient Chinese state * Yang, Iran, a village in Razavi Khorasan Province * Yang River (other) People * Yang, one of the names for the Karen people in the Thai language *Yang di-Pertuan Agong, the constitutional monarch of Malaysia * Yang (surname), Chinese surname * Yang (Korean surname) Fictional characters * Cristina Yang, on the TV show ''Grey's Anatomy'' * Yang, from the show ''Yin Yang Yo!'' * Yang, Experiment 502 in '' Lilo and Stitch: The Series'' * Yang Fang Leiden, from ''Final Fantasy IV'' * Yang Lee, in the ''Street Fighter III'' series of videogames * Mr. Yang, the Yin Yang serial killer in ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Yanghui Magic Circle 2
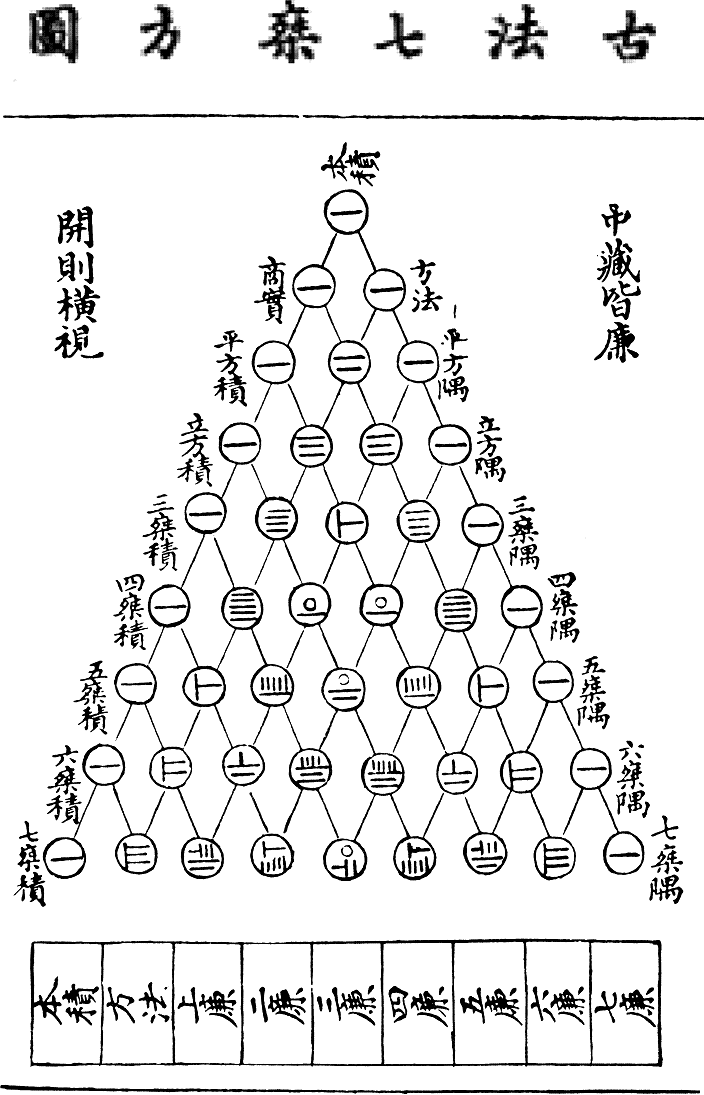
Yang Hui (, ca. 1238–1298), courtesy name Qianguang (), was a Chinese mathematician and writer during the Song dynasty. Originally, from Qiantang (modern Hangzhou, Zhejiang), Yang worked on magic squares, magic circles and the binomial theorem, and is best known for his contribution of presenting Yang Hui's Triangle. This triangle was the same as Pascal's Triangle, discovered by Yang's predecessor Jia Xian. Yang was also a contemporary to the other famous mathematician Qin Jiushao. Written work The earliest extant Chinese illustration of 'Pascal's triangle' is from Yang's book ''Xiangjie Jiuzhang Suanfa'' ()Fragments of this book was retained in the Yongle Encyclopedia vol 16344, in British Museum Library of 1261 AD, in which Yang acknowledged that his method of finding square roots and cubic roots using "Yang Hui's Triangle" was invented by mathematician Jia XianNeedham, Volume 3, 134-137. who expounded it around 1100 AD, about 500 years before Pascal. In his book (now lost ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Wu Wenjun
Wu Wenjun ( zh, s=吴文俊; 12 May 1919 – 7 May 2017), also commonly known as Wu Wen-tsün, was a Chinese mathematician, historian, and writer. He was an academician at the Chinese Academy of Sciences (CAS), best known for the Wu's method of characteristic set. Biography Wu's ancestral hometown was Jiashan, Zhejiang. He was born in Shanghai and graduated from Shanghai Jiao Tong University in 1940. In 1945, Wu taught several months at Hangchow University (later merged into Zhejiang University) in Hangzhou. In 1947, he went to France for further study at the University of Strasbourg. In 1949, he received his PhD, for his thesis ''Sur les classes caractéristiques des structures fibrées sphériques'', written under the direction of Charles Ehresmann. Afterwards, he did some work in Paris with René Thom and discovered the Wu class and Wu formula in algebraic topology. In 1951 he was appointed to a post at Peking University. However, Wu may have been among a wave of reca ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Most-perfect Magic Square
A most-perfect magic square of order ''n'' is a magic square containing the numbers 1 to ''n''2 with two additional properties: # Each 2 × 2 subsquare sums to 2''s'', where ''s'' = ''n''2 + 1. # All pairs of integers distant ''n''/2 along a (major) diagonal sum to ''s''. __TOC__ Examples Two 12 × 12 most-perfect magic squares can be obtained adding 1 to each element of: 1 2 3 4 5 6 7 8 9 10 11 12'' ,'' 64 92 81 94 48 77 67 63 50 61 83 78 ,'' 31 99 14 97 47 114 28 128 45 130 12 113 ,'' 24 132 41 134 8 117 27 103 10 101 43 118 ,'' 23 107 6 105 39 122 20 136 37 138 4 121 ,'' 16 140 33 142 0 125 19 111 2 109 35 126 ,'' 75 55 58 53 91 70 72 84 89 86 56 69 ,'' 76 80 93 82 60 65 79 51 62 49 95 66 ,'' 115 15 98 13 131 30 ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Magic Circle Derived From Magic Square
Magic or Magick most commonly refers to: * Magic (supernatural), beliefs and actions employed to influence supernatural beings and forces * Ceremonial magic, encompasses a wide variety of rituals of magic * Magical thinking, the belief that unrelated events are causally connected, particularly as a result of supernatural effects * Magic (illusion), the art of appearing to perform supernatural feats Magic(k) may also refer to: Art and entertainment Film and television * ''Magic'' (1917 film), a silent Hungarian drama * ''Magic'' (1978 film), an American horror film * ''Magic'' (soap opera), 2013 Indonesian soap opera * Magic (TV channel), a British music television station Literature * Magic in fiction, the genre of fiction that uses supernatural elements as a theme * ''Magic'' (Chesterton play), 1913 * ''Magic'' (short story collection), 1996 short story collection by Isaac Asimov * ''Magic'' (novel), 1976 novel by William Goldman * ''The Magic Comic'', a 1939–1 ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Sphere
A sphere () is a Geometry, geometrical object that is a solid geometry, three-dimensional analogue to a two-dimensional circle. A sphere is the Locus (mathematics), set of points that are all at the same distance from a given point in three-dimensional space.. That given point is the centre (geometry), centre of the sphere, and is the sphere's radius. The earliest known mentions of spheres appear in the work of the Greek mathematics, ancient Greek mathematicians. The sphere is a fundamental object in many fields of mathematics. Spheres and nearly-spherical shapes also appear in nature and industry. Bubble (physics), Bubbles such as soap bubbles take a spherical shape in equilibrium. spherical Earth, The Earth is often approximated as a sphere in geography, and the celestial sphere is an important concept in astronomy. Manufactured items including pressure vessels and most curved mirrors and lenses are based on spheres. Spheres rolling, roll smoothly in any direction, so mos ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Andrews Magic Sphere
Andrews may refer to: Places Australia *Andrews, Queensland *Andrews, South Australia United States *Andrews, Florida (other), various places *Andrews, Indiana * Andrews, Nebraska *Andrews, North Carolina * Andrews, Oregon *Andrews, South Carolina *Andrews, Texas *Andrews County, Texas *Andrews Air Force Base near Washington, D.C., home of Air Force One *Andrews University (Michigan) Philippines *Andrews Avenue, a major thoroughfare in Metro Manila, Philippines Other *Andrews (surname) *''Andrews v Law Society of British Columbia'', a 1989 Supreme Court of Canada case on constitutional equality guarantees *''Joseph Andrews'', a novel by Henry Fielding *''An Apology for the Life of Mrs. Shamela Andrews'', a parody novel *Andrews, a bus company in Sheffield, South Yorkshire, England, that merged with Yorkshire Traction *Andrews Osborne Academy, a private school in Willoughby, Ohio *Henry Cranke Andrews (fl. 1794 – 1830), English botanist (standard author abbreviation An ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Suanfa Tongzong
''Suanfa tongzong'' ( zh, 算法統宗) is a mathematical text written by sixteenth century Chinese mathematician Cheng Dawei (1533–1606) and published in the year 1592. The book contains 595 problems divided into 17 chapters. The book is essentially general arithmetic for the abacus. The book was the main source available to scholars concerning mathematics as it developed in China's tradition. Six years after the publication of Suanfa Tongzong, Cheng Dawei published another book titled ''Suanfa Zuanyao'' (''A Compendium of calculating Methods''). About 90% of the content of the new book came from the contents of four chapters of the first book with some rearrangement. It is said that when Suanfa Tongzong was first published, it sold so many copies that the cost of paper went up and the lucrative sales resulted in unscrupulous people beginning to print pirated copies of the book with many errors. It was this that forced the author to print an abridged version. Some features ''Sua ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Magic Square
In recreational mathematics, a square array of numbers, usually positive integers, is called a magic square if the sums of the numbers in each row, each column, and both main diagonals are the same. The 'order' of the magic square is the number of integers along one side (''n''), and the constant sum is called the ' magic constant'. If the array includes just the positive integers 1,2,...,n^2, the magic square is said to be 'normal'. Some authors take magic square to mean normal magic square. Magic squares that include repeated entries do not fall under this definition and are referred to as 'trivial'. Some well-known examples, including the Sagrada Família magic square and the Parker square are trivial in this sense. When all the rows and columns but not both diagonals sum to the magic constant this gives a ''semimagic square (sometimes called orthomagic square). The mathematical study of magic squares typically deals with their construction, classification, and enumeration. ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Ding Yidong Magic Circle
Ding may refer to: Bronze and ceramics * Ding (vessel), a bronze or ceramic cauldron used in ancient and early imperial China * Ding ware, ceramics produced in Dingzhou in medieval China People * Ding (surname) (丁), a Chinese surname and list of people with the name * Duke Ding of Jin (died 475 BC), ruler of Jin * Duke Ding of Qi, tenth century ruler of Qi * Empress Dowager Ding (died 402), empress dowager of the state of Later Yan * King Ding of Zhou, king of the Zhou Dynasty in ancient China from 606 to 586 BC * Ding Darling (1876–1962), American cartoonist who signed his work "Ding" Arts and entertainment * "Ding" (song), by Seeed * Ding, the nickname of Domingo Chavez, a recurring character in Tom Clancy's novels and video games * ''Ding'', a webcomic by Scott Kurtz * D!NG, a spinoff web channel from Vsauce Places * Dingzhou, formerly Ding County and Ding Prefecture, China * Ding railway station, Haryana, India Other uses * (ding) or Gnus, a news reader * Ding l ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
YangHui Magic Circle 1
Yang Hui (, ca. 1238–1298), courtesy name Qianguang (), was a Chinese mathematician and writer during the Song dynasty. Originally, from Qiantang (modern Hangzhou, Zhejiang), Yang worked on magic squares, magic circles and the binomial theorem, and is best known for his contribution of presenting Yang Hui's Triangle. This triangle was the same as Pascal's Triangle, discovered by Yang's predecessor Jia Xian. Yang was also a contemporary to the other famous mathematician Qin Jiushao. Written work The earliest extant Chinese illustration of 'Pascal's triangle' is from Yang's book ''Xiangjie Jiuzhang Suanfa'' ()Fragments of this book was retained in the Yongle Encyclopedia vol 16344, in British Museum Library of 1261 AD, in which Yang acknowledged that his method of finding square roots and cubic roots using "Yang Hui's Triangle" was invented by mathematician Jia XianNeedham, Volume 3, 134-137. who expounded it around 1100 AD, about 500 years before Pascal. In his book (now lost ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Song Dynasty
The Song dynasty (; ; 960–1279) was an imperial dynasty of China that began in 960 and lasted until 1279. The dynasty was founded by Emperor Taizu of Song following his usurpation of the throne of the Later Zhou. The Song conquered the rest of the Ten Kingdoms, ending the Five Dynasties and Ten Kingdoms period. The Song often came into conflict with the contemporaneous Liao, Western Xia and Jin dynasties in northern China. After retreating to southern China, the Song was eventually conquered by the Mongol-led Yuan dynasty. The dynasty is divided into two periods: Northern Song and Southern Song. During the Northern Song (; 960–1127), the capital was in the northern city of Bianjing (now Kaifeng) and the dynasty controlled most of what is now Eastern China. The Southern Song (; 1127–1279) refers to the period after the Song lost control of its northern half to the Jurchen-led Jin dynasty in the Jin–Song Wars. At that time, the Song court retreated south o ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |