|
List Of Things Named After Karl Schwarzschild
{{Short description, none Things named after the astronomy and relativity scientist Karl Schwarzschild (1873–1916) include: * Institutions: ** Karl Schwarzschild Medal ** Karl Schwarzschild Observatory * Astronomical features: ** Lunar crater Schwarzschild ** Asteroid 837 Schwarzschilda * Technical terms: ** Schwarzschild constant ** Schwarzschild effect in photography, also known as reciprocity failure, and important for calibrating astronomical measurement *** Schwarzschild law, empirical equation relating to Schwarzschild effect ** Schwarzschild criterion, in astronomy ** Schwarzschild coordinates ** Schwarzschild's equation for radiative transfer ** Relativity terms: *** Schwarzschild metric (closely related to ''Schwarzschild solution'', ''Schwarzschild geometry'', ''Schwarzschild black hole'', and ''Schwarzschild vacuum'') ****de Sitter–Schwarzschild metric ****Distorted Schwarzschild metric **** Schwarzschild geodesics *** Schwarzschild fluid solution *** Schwarzsch ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
General Relativity
General relativity, also known as the general theory of relativity and Einstein's theory of gravity, is the geometric theory of gravitation published by Albert Einstein in 1915 and is the current description of gravitation in modern physics. General relativity generalizes special relativity and refines Newton's law of universal gravitation, providing a unified description of gravity as a geometric property of space and time or four-dimensional spacetime. In particular, the ' is directly related to the energy and momentum of whatever matter and radiation are present. The relation is specified by the Einstein field equations, a system of second order partial differential equations. Newton's law of universal gravitation, which describes classical gravity, can be seen as a prediction of general relativity for the almost flat spacetime geometry around stationary mass distributions. Some predictions of general relativity, however, are beyond Newton's law of universal gravitat ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Schwarzschild's Equation For Radiative Transfer
In the study of heat transfer, Schwarzschild's equation Those without access to a text discussing Schwarzschild's equation can find a discussion abstracted from these pages at: https://scienceofdoom.com/2011/02/07/understanding-atmospheric-radiation-and-the-“greenhouse”-effect-–-part-six-the-equations/. is used to calculate radiative transfer ( energy transfer via electromagnetic radiation) through a medium in local thermodynamic equilibrium that both absorbs and emits radiation. The incremental change in spectral intensity, (, /sr/m2/μm at a given wavelength as radiation travels an incremental distance () through a non-scattering medium is given by: dI _\lambda = n\sigma_\lambda B_\lambda(T) \, ds - n\sigma_\lambda I_\lambda \, ds = n \sigma_\lambda _\lambda(T) - I_\lambda\, ds where * is the density of absorbing/emitting molecules, * is their absorption cross-section at wavelength , * is the Planck function for temperature and wavelength , * is the spectral intens ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
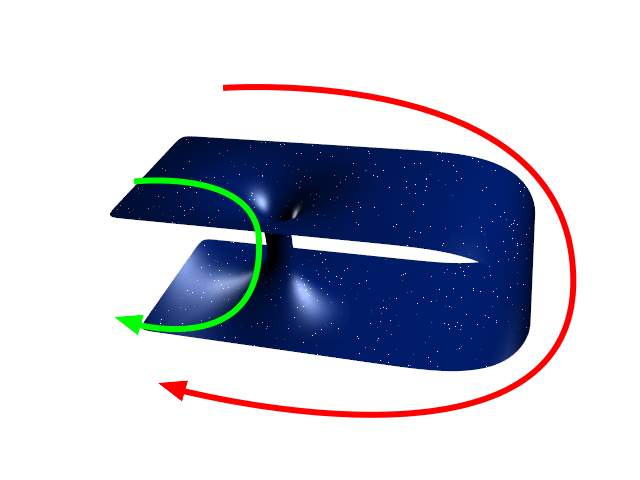Wormhole
A wormhole (Einstein-Rosen bridge) is a hypothetical structure connecting disparate points in spacetime, and is based on a special Solutions of the Einstein field equations, solution of the Einstein field equations. A wormhole can be visualized as a tunnel with two ends at separate points in spacetime (i.e., different locations, different points in time, or both). Wormholes are consistent with the General relativity, general theory of relativity, but whether wormholes actually exist remains to be seen. Many scientists postulate that wormholes are merely projections of a Four-dimensional space, fourth spatial dimension, analogous to how a two-dimensional (2D) being could experience only part of a three-dimensional (3D) object. Theoretically, a wormhole might connect extremely long distances such as a billion light years, or short distances such as a few meters, or different points in time, or even multiverse, different universes. In 1995, Matt Visser suggested there may be ma ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Schwarzschild Radius
The Schwarzschild radius or the gravitational radius is a physical parameter in the Schwarzschild solution to Einstein's field equations that corresponds to the radius defining the event horizon of a Schwarzschild black hole. It is a characteristic radius associated with any quantity of mass. The Schwarzschild radius was named after the German astronomer Karl Schwarzschild, who calculated this exact solution for the theory of general relativity in 1916. The Schwarzschild radius is given as r_\text = \frac , where ''G'' is the gravitational constant, ''M'' is the object mass, and ''c'' is the speed of light. History In 1916, Karl Schwarzschild obtained the exact solution to Einstein's field equations for the gravitational field outside a non-rotating, spherically symmetric body with mass M (see Schwarzschild metric). The solution contained terms of the form 1-/r and \frac , which become singular at r = 0 and r=r_\text respectively. The r_\text has come to be known as the ''Schwa ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Kugelblitz (astrophysics)
A kugelblitz is a theoretical astrophysical object predicted by general relativity. It is a concentration of heat, light or radiation so intense that its energy forms an event horizon and becomes self-trapped. In other words, if enough radiation is aimed into a region of space, the concentration of energy can warp spacetime so much that it creates a black hole. This would be a black hole whose original mass–energy was in the form of radiant energy rather than matter , however as soon as it forms, it is indistinguishable from an ordinary Black Hole, meaning there is currently no way to trace the origins of a Black Hole. John Archibald Wheeler's 1955 ''Physical Review'' paper entitled " geons" refers to the kugelblitz phenomena and explores the idea of creating such particles (or toy models of particles) from spacetime curvature. The kugelblitz phenomenon has been considered a possible basis for interstellar engines (drives) for future black hole starships. See also * Beke ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Schwarzschild Fluid Solution
In Einstein's theory of general relativity, the interior Schwarzschild metric (also interior Schwarzschild solution or Schwarzschild fluid solution) is an exact solution for the gravitational field in the interior of a non-rotating spherical body which consists of an incompressible fluid (implying that density is constant throughout the body) and has zero pressure at the surface. This is a static solution, meaning that it does not change over time. It was discovered by Karl Schwarzschild in 1916, who earlier had found the exterior Schwarzschild metric. Mathematics The interior Schwarzschild metric is framed in a spherical coordinate system with the body's centre located at the origin, plus the time coordinate. Its line element is : c^2 ^ = -\frac \left( 3 \sqrt-\sqrt \right)^2 c^2 dt^2 + \left( 1-\frac \right)^ dr^2 + r^2 \left(d\theta^2 + \sin^2\theta \, d\varphi^2\right), where * \tau is the proper time (time measured by a clock moving along the same world line with the te ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
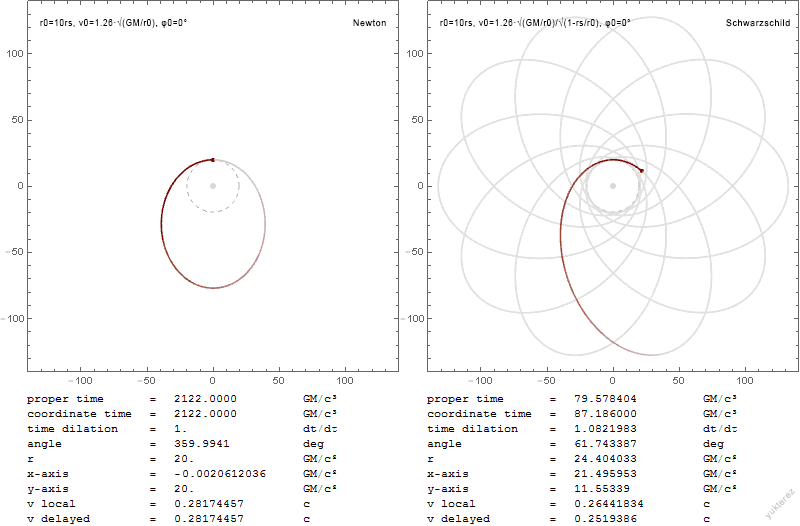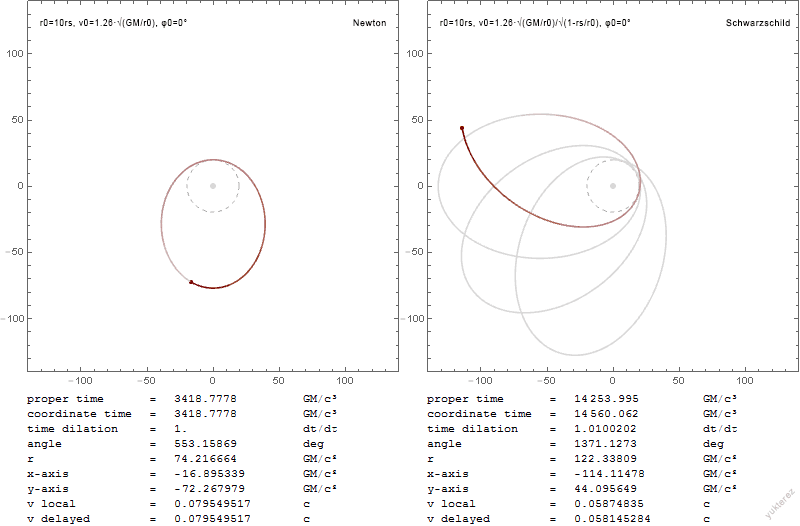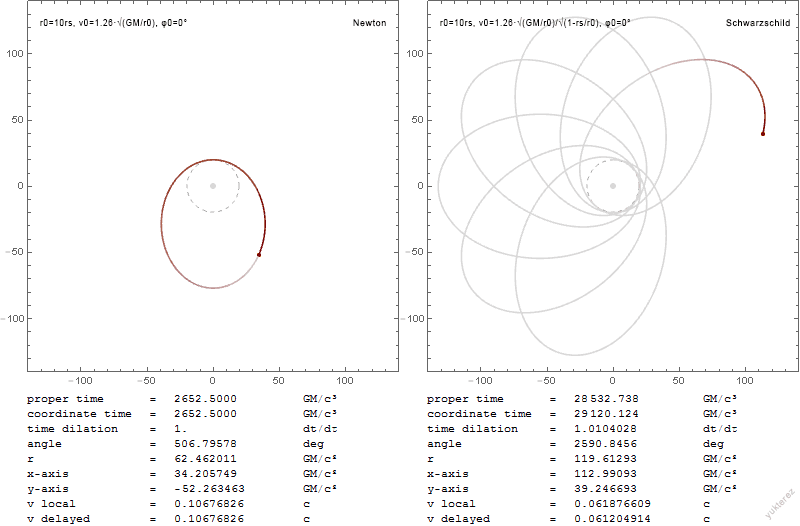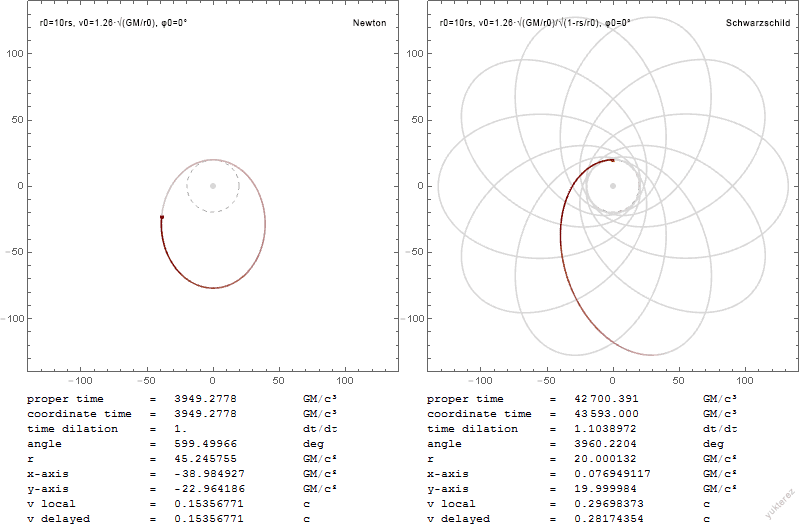Schwarzschild Geodesics
In general relativity, Schwarzschild geodesics describe the motion of test particles in the gravitational field of a central fixed mass M, that is, motion in the Schwarzschild metric. Schwarzschild geodesics have been pivotal in the validation of Einstein's theory of general relativity. For example, they provide accurate predictions of the anomalous precession of the planets in the Solar System and of the deflection of light by gravity. Schwarzschild geodesics pertain only to the motion of particles of masses so small they contribute little to the gravitational field. However, they are highly accurate in many astrophysical scenarios provided that m is many-fold smaller than the central mass M, e.g., for planets orbiting their sun. Schwarzschild geodesics are also a good approximation to the relative motion of two bodies of arbitrary mass, provided that the Schwarzschild mass M is set equal to the sum of the two individual masses m_1 and m_2. This is important in predicting the mo ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Distorted Schwarzschild Metric
In physics, the distorted Schwarzschild metric is the metric of a standard/isolated Schwarzschild spacetime exposed in external fields. In numerical simulation, the Schwarzschild metric can be distorted by almost arbitrary kinds of external energy–momentum distribution. However, in exact analysis, the mature method to distort the standard Schwarzschild metric is restricted to the framework of Weyl metrics. Standard Schwarzschild as a vacuum Weyl metric All static axisymmetric solutions of the Einstein–Maxwell equations can be written in the form of Weyl's metric,Jeremy Bransom Griffiths, Jiri Podolsky. ''Exact Space-Times in Einstein's General Relativity''. Cambridge: Cambridge University Press, 2009. Chapter 10. (1)\quad ds^2=-e^dt^2+e^(d\rho^2+dz^2)+e^\rho^2 d\phi^2\,, From the Weyl perspective, the metric potentials generating the standard Schwarzschild solution are given byR Gautreau, R B Hoffman, A Armenti. ''Static multiparticle systems in general relativity''. IL NU ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
De Sitter–Schwarzschild Metric
In general relativity, the de Sitter–Schwarzschild solution describes a black hole in a causal patch of de Sitter space. Unlike a flat-space black hole, there is a largest possible de Sitter black hole, which is the Nariai spacetime. The Nariai limit has no singularities, the cosmological and black hole horizons have the same area, and they can be mapped to each other by a discrete reflection symmetry in any causal patch. Introduction In general relativity, space-times can have black hole event horizons and also cosmological horizons. The de Sitter–Schwarzschild solution is the simplest solution which has both. Metric The metric of any spherically symmetric solution in Schwarzschild form is: :: ds^2 = - f(r) dt^2 + + r^2(d\theta^2 + \sin^2\theta \,d\phi^2) \, The vacuum Einstein equations give a ''linear'' equation for ''ƒ''(''r''), which has as solutions: :: f(r)=1-2a/r \, :: f(r)= 1 - b r^2 \, The first is a zero stress energy solution describing a black hole ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Schwarzschild Metric
In Einstein's theory of general relativity, the Schwarzschild metric (also known as the Schwarzschild solution) is an exact solution to the Einstein field equations that describes the gravitational field outside a spherical mass, on the assumption that the electric charge of the mass, angular momentum of the mass, and universal cosmological constant are all zero. The solution is a useful approximation for describing slowly rotating astronomical objects such as many stars and planets, including Earth and the Sun. It was found by Karl Schwarzschild in 1916, and around the same time independently by Johannes Droste, who published his more complete and modern-looking discussion four months after Schwarzschild. According to Birkhoff's theorem, the Schwarzschild metric is the most general spherically symmetric vacuum solution of the Einstein field equations. A Schwarzschild black hole or static black hole is a black hole that has neither electric charge nor angular momentum. A Schwar ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Schwarzschild Coordinates
In the theory of Lorentzian manifolds, spherically symmetric spacetimes admit a family of ''nested round spheres''. In such a spacetime, a particularly important kind of coordinate chart is the Schwarzschild chart, a kind of polar spherical coordinate chart on a static and spherically symmetric spacetime, which is ''adapted'' to these nested round spheres. The defining characteristic of Schwarzschild chart is that the radial coordinate possesses a natural geometric interpretation in terms of the surface area and Gaussian curvature of each sphere. However, radial distances and angles are not accurately represented. These charts have many applications in metric theories of gravitation such as general relativity. They are most often used in static spherically symmetric spacetimes. In the case of general relativity, Birkhoff's theorem states that every ''isolated'' spherically symmetric vacuum or electrovacuum solution of the Einstein field equation is static, but this is certainl ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Karl Schwarzschild
Karl Schwarzschild (; 9 October 1873 – 11 May 1916) was a German physicist and astronomer. Schwarzschild provided the first exact solution to the Einstein field equations of general relativity, for the limited case of a single spherical non-rotating mass, which he accomplished in 1915, the same year that Einstein first introduced general relativity. The Schwarzschild solution, which makes use of Schwarzschild coordinates and the Schwarzschild metric, leads to a derivation of the Schwarzschild radius, which is the size of the event horizon of a non-rotating black hole. Schwarzschild accomplished this while serving in the German army during World War I. He died the following year from the autoimmune disease pemphigus, which he developed while at the Russian front. Various forms of the disease particularly affect people of Ashkenazi Jewish origin. Asteroid 837 Schwarzschilda is named in his honour, as is the large crater ''Schwarzschild'', on the far side of the Moon. Life ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
