|
List Of Things Named After Johann Lambert
This article is a list of things named in the memory of the 18th century Swiss scientist Johann Heinrich Lambert: Optics * Beer–Lambert law **Beer–Lambert–Bouguer law, see above * lambert (unit) ** Foot-lambert * Lambert's cosine law * Lambertian reflectance Mathematics * Lambert's theorem on the parabola * Lambert azimuthal equal-area projection * Lambert conformal conic projection * Lambert cylindrical equal-area projection * Lambert mean * Lambert problem * Lambert quadrilateral * Lambert series * Lambert's trinomial equation * Lambert W function * Lambertian function (inverse of the Gudermannian function) Other * 187 Lamberta, asteroid * Lambert (lunar crater). In the MARE IMBRIUM, Diameter: 30.1209 km * Lambert (Martian crater). In the Sinus Sabaeus quadrangle of Mars, located at 20.2°S latitude and 334.7°W longitude. It is 92.0 km in diameter References {{reflist, refs= {{cite book , last1=Schmadel , first=Lutz D. , title=Dictionary of Minor Plan ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
|
 |
Johann Heinrich Lambert
Johann Heinrich Lambert (, ''Jean-Henri Lambert'' in French; 26 or 28 August 1728 – 25 September 1777) was a polymath from the Republic of Mulhouse, generally referred to as either Swiss or French, who made important contributions to the subjects of mathematics, physics (particularly optics), philosophy, astronomy and map projections. Biography Lambert was born in 1728 into a Huguenot family in the city of Mulhouse (now in Alsace, France), at that time a city-state allied to Switzerland. Some sources give 26 August as his birth date and others 28 August. Leaving school at 12, he continued to study in his free time while undertaking a series of jobs. These included assistant to his father (a tailor), a clerk at a nearby iron works, a private tutor, secretary to the editor of ''Basler Zeitung'' and, at the age of 20, private tutor to the sons of Count Salis in Chur. Travelling Europe with his charges (1756–1758) allowed him to meet established mathematicians in the German ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
|
Lambert's Problem
In celestial mechanics, Lambert's problem is concerned with the determination of an orbit from two position vectors and the time of flight, posed in the 18th century by Johann Heinrich Lambert and formally solved with mathematical proof by Joseph-Louis Lagrange. It has important applications in the areas of rendezvous, targeting, guidance, and preliminary orbit determination. Suppose a body under the influence of a central gravitational force is observed to travel from point ''P''1 on its conic trajectory, to a point ''P''2 in a time ''T''. The time of flight is related to other variables by Lambert's theorem, which states: :''The transfer time of a body moving between two points on a conic trajectory is a function only of the sum of the distances of the two points from the origin of the force, the linear distance between the points, and the semimajor axis of the conic.'' Stated another way, Lambert's problem is the boundary value problem for the differential equation \ddot = -\m ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
|
|
Lambert (lunar Crater)
Lambert is a lunar impact crater on the southern half of the Mare Imbrium basin. It was named after Swiss polymath Johann Heinrich Lambert. It lies to the east and somewhat south of the slightly larger crater Timocharis. To the south is the smaller Pytheas, and some distance to the west-southwest is Euler. The crater is relatively easy to locate due to its isolated position on the mare. It has an outer rampart, terraced inner walls, and a rough interior that has a comparable albedo to its surroundings. Instead of a central peak, a small craterler lies at the midpoint of the interior. Just to the south of Lambert's ramparts is the lava-covered rim of Lambert R, a crater that is almost completely covered by the mare. The diameter of this ghost crater is larger than Lambert, but it is difficult to spot except when the Sun is at a very low angle, casting long shadows. Satellite craters By convention these features are identified on lunar maps by placing the letter on the side of t ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
|
|
187 Lamberta
Lamberta (minor planet designation: 187 Lamberta) is a main-belt asteroid that was discovered by Corsican-born French astronomer Jérôme Eugène Coggia on April 11, 1878, and named after the astronomer Johann Heinrich Lambert. It was the second of Coggia's five asteroid discoveries. The spectrum matches a classification of a C-type asteroid, which may mean it has a composition of primitive carbonaceous materials. It is a dark object as indicated by the low albedo Albedo (; ) is the measure of the diffuse reflection of sunlight, solar radiation out of the total solar radiation and measured on a scale from 0, corresponding to a black body that absorbs all incident radiation, to 1, corresponding to a body ... and has an estimated size of about 131 km. References External links * * Background asteroids Lamberta Lamberta C-type asteroids (Tholen) Ch-type asteroids (SMASS) 18780411 {{C-beltasteroid-stub ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
|
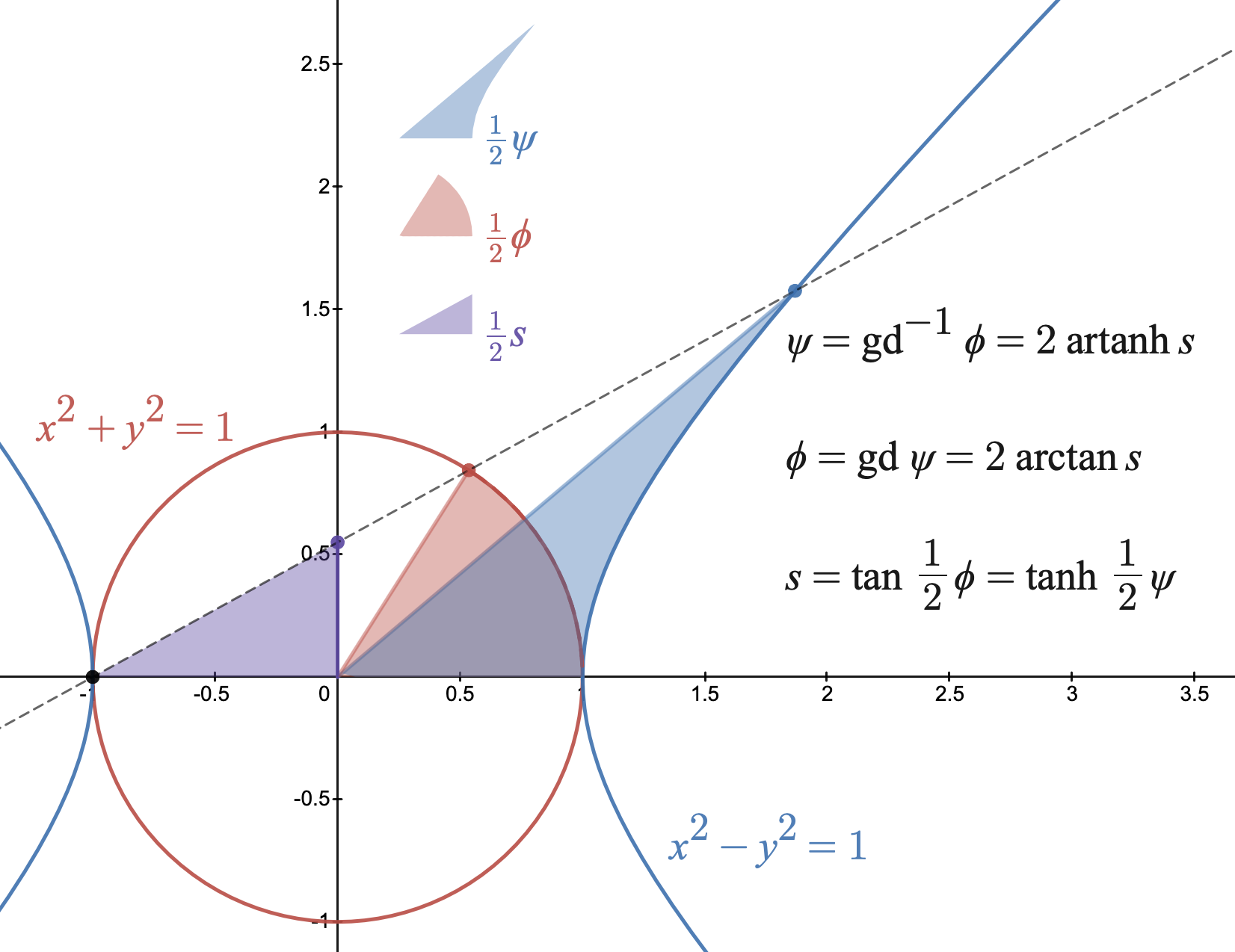 |
Gudermannian Function
In mathematics, the Gudermannian function relates a hyperbolic angle measure \psi to a circular angle measure \phi called the ''gudermannian'' of \psi and denoted \operatorname\psi. The Gudermannian function reveals a close relationship between the circular functions and hyperbolic functions. It was introduced in the 1760s by Johann Heinrich Lambert, and later named for Christoph Gudermann who also described the relationship between circular and hyperbolic functions in 1830. The gudermannian is sometimes called the hyperbolic amplitude as a limiting case of the Jacobi elliptic amplitude \operatorname(\psi, m) when parameter m=1. The real Gudermannian function is typically defined for -\infty < \psi < \infty to be the integral of the hyperbolic secant The real inverse Gudermannian function can be defined for as the [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
 |
Lambert W Function
In mathematics, the Lambert function, also called the omega function or product logarithm, is a multivalued function, namely the branches of the converse relation of the function , where is any complex number and is the exponential function. For each integer there is one branch, denoted by , which is a complex-valued function of one complex argument. is known as the principal branch. These functions have the following property: if and are any complex numbers, then :w e^ = z holds if and only if :w=W_k(z) \ \ \text k. When dealing with real numbers only, the two branches and suffice: for real numbers and the equation :y e^ = x can be solved for only if ; we get if and the two values and if . The Lambert relation cannot be expressed in terms of elementary functions. It is useful in combinatorics, for instance, in the enumeration of trees. It can be used to solve various equations involving exponentials (e.g. the maxima of the Planck, Bose–Einstein, ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
|
Trinomial Equation
In elementary algebra, a trinomial is a polynomial consisting of three terms or monomials. Examples of trinomial expressions # 3x + 5y + 8z with x, y, z variables # 3t + 9s^2 + 3y^3 with t, s, y variables # 3ts + 9t + 5s with t, s variables # ax^2+bx+c, the quadratic expression in standard form with a,b,c variables. # A x^a y^b z^c + B t + C s with x, y, z, t, s variables, a, b, c nonnegative integers and A, B, C any constants. # Px^a + Qx^b + Rx^c where x is variable and constants a, b, c are nonnegative integers and P, Q, R any constants. Trinomial equation A trinomial equation is a polynomial equation involving three terms. An example is the equation x = q + x^m studied by Johann Heinrich Lambert in the 18th century. Some notable trinomials * The quadratic trinomial in standard form (as from above): ax^2+bx+c See also *Trinomial expansion *Monomial *Binomial * Multinomial * Simple expression *Compound expression In mathematics, an expression or mathematical expr ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
|
 |
Lambert Series
In mathematics, a Lambert series, named for Johann Heinrich Lambert, is a series taking the form :S(q)=\sum_^\infty a_n \frac . It can be resumed formally by expanding the denominator: :S(q)=\sum_^\infty a_n \sum_^\infty q^ = \sum_^\infty b_m q^m where the coefficients of the new series are given by the Dirichlet convolution of ''a''''n'' with the constant function 1(''n'') = 1: :b_m = (a*1)(m) = \sum_ a_n. \, This series may be inverted by means of the Möbius inversion formula, and is an example of a Möbius transform. Examples Since this last sum is a typical number-theoretic sum, almost any natural multiplicative function will be exactly summable when used in a Lambert series. Thus, for example, one has :\sum_^\infty q^n \sigma_0(n) = \sum_^\infty \frac where \sigma_0(n)=d(n) is the number of positive divisors of the number ''n''. For the higher order sum-of-divisor functions, one has :\sum_^\infty q^n \sigma_\alpha(n) = \sum_^\infty \frac ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
|
Lambert Quadrilateral
In geometry, a Lambert quadrilateral (also known as Ibn al-Haytham–Lambert quadrilateral), is a quadrilateral in which three of its angles are right angles. Historically, the fourth angle of a Lambert quadrilateral was of considerable interest since if it could be shown to be a right angle, then the Euclidean parallel postulate could be proved as a theorem. It is now known that the type of the fourth angle depends upon the geometry in which the quadrilateral exists. In hyperbolic geometry the fourth angle is acute, in Euclidean geometry it is a right angle and in elliptic geometry it is an obtuse angle. A Lambert quadrilateral can be constructed from a Saccheri quadrilateral by joining the midpoints of the base and summit of the Saccheri quadrilateral. This line segment is perpendicular to both the base and summit and so either half of the Saccheri quadrilateral is a Lambert quadrilateral. Lambert quadrilateral in hyperbolic geometry In hyperbolic geometry a Lambert quadril ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
|
|
Lambert Mean
Lambert may refer to People *Lambert (name), a given name and surname * Lambert, Bishop of Ostia (c. 1036–1130), became Pope Honorius II *Lambert, Margrave of Tuscany (fl. 929–931), also count and duke of Lucca *Lambert (pianist), stage-name of German pianist and composer Paul Lambert Places United States *Lambert, Mississippi, a town *Lambert, Missouri, a village * St. Louis Lambert International Airport, St. Louis, Missouri *Lambert, Montana, a rural town in Montana *Lambert, Oklahoma, a town * Lambert Township, Red Lake County, Minnesota * Lambert Castle, a mansion in Paterson, New Jersey *Lambert Creek, San Mateo County, California Elsewhere *Lambert Gravitational Centre, the geographical centre of Australia *Lambert (lunar crater), named after Johann Heinrich Lambert * Lambert (Martian crater), named after Johann Heinrich Lambert Transportation *Lambert (automobile), a defunct American automobile brand * Lambert (cyclecar), British three-wheeled cyclecar *''Lambert'', ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
|
|
Beer–Lambert Law
The Beer–Lambert law, also known as Beer's law, the Lambert–Beer law, or the Beer–Lambert–Bouguer law relates the attenuation of light to the properties of the material through which the light is travelling. The law is commonly applied to chemical analysis measurements and used in understanding attenuation in physical optics, for photons, neutrons, or rarefied gases. In mathematical physics, this law arises as a solution of the BGK equation. History The law was discovered by Pierre Bouguer before 1729, while looking at red wine, during a brief vacation in Alentejo, Portugal. It is often attributed to Johann Heinrich Lambert, who cited Bouguer's ''Essai d'optique sur la gradation de la lumière'' (Claude Jombert, Paris, 1729) – and even quoted from it – in his '' Photometria'' in 1760. Lambert's law stated that the loss of light intensity when it propagates in a medium is directly proportional to intensity and path length. Much later, the German scientist August ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
|
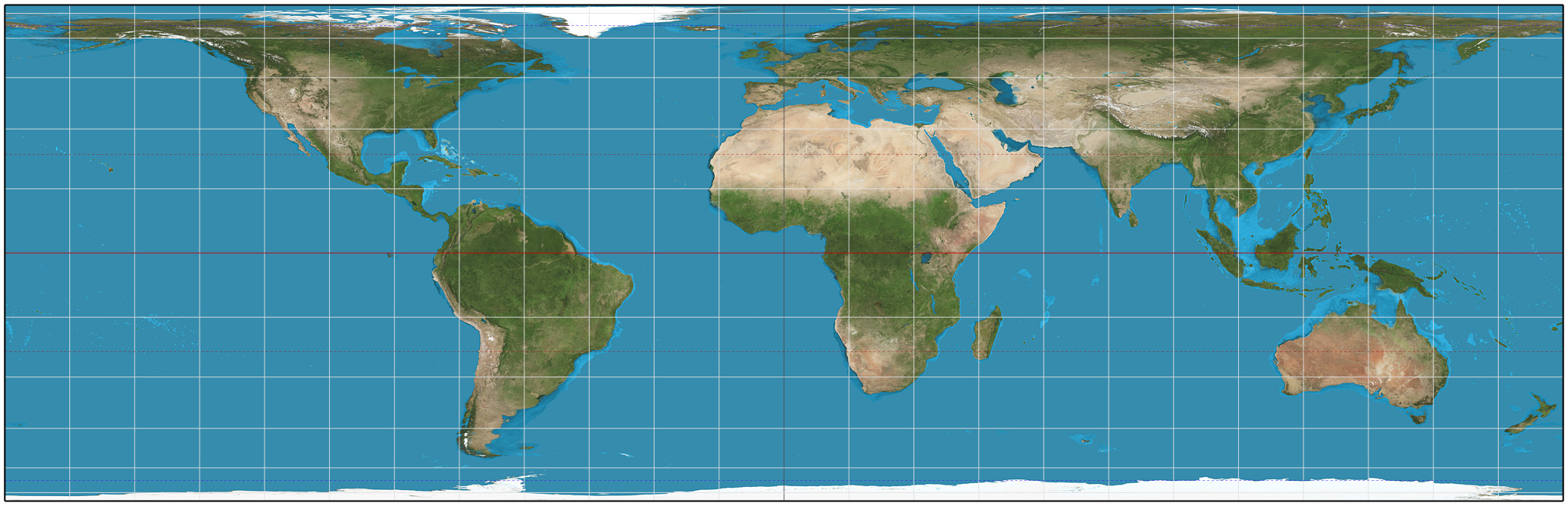 |
Lambert Cylindrical Equal-area Projection
In cartography, the Lambert cylindrical equal-area projection, or Lambert cylindrical projection, is a cylindrical equal-area projection. This projection is undistorted along the equator, which is its standard parallel, but distortion increases rapidly towards the poles. Like any cylindrical projection, it stretches parallels increasingly away from the equator. The poles accrue infinite distortion, becoming lines instead of points. History The projection was invented by the Swiss mathematician Johann Heinrich Lambert and described in his 1772 treatise, ''Beiträge zum Gebrauche der Mathematik und deren Anwendung'', part III, section 6: ''Anmerkungen und Zusätze zur Entwerfung der Land- und Himmelscharten'', translated as, ''Notes and Comments on the Composition of Terrestrial and Celestial Maps''. Lambert's projection is the basis for the cylindrical equal-area projection family. Lambert chose the equator as the parallel of no distortion. By multiplying the projection's heigh ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |