|
Laguerre–Forsyth Invariant
In projective geometry, the Laguerre–Forsyth invariant is a cubic differential that is an invariant of a projective plane curve. It is named for Edmond Laguerre and Andrew Forsyth, the latter of whom analyzed the invariant in an influential book on ordinary differential equations. Suppose that p:\mathbf P^1\to\mathbf P^2 is a three-times continuously differentiable immersion of the projective line into the projective plane, with homogeneous coordinates given by p(t)=(x_1(t),x_2(t),x_3(t)) then associated to ''p'' is the third-order ordinary differential equation :\left, \begin x&x'&x''&x\\ x_1&x_1'&x_1''&x_1\\ x_2&x_2'&x_2''&x_2\\ x_3&x_3'&x_3''&x_3\\ \end\ = 0. Generically, this equation can be put into the form :x+Ax''+Bx'+Cx = 0 where A,B,C are rational functions of the components of ''p'' and its derivatives. After a change of variables of the form t\to f(t), x\to g(t)^x, this equation can be further reduced to an equation without first or second derivative terms :x + Rx = 0 ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Projective Geometry
In mathematics, projective geometry is the study of geometric properties that are invariant with respect to projective transformations. This means that, compared to elementary Euclidean geometry, projective geometry has a different setting, projective space, and a selective set of basic geometric concepts. The basic intuitions are that projective space has more points than Euclidean space, for a given dimension, and that geometric transformations are permitted that transform the extra points (called "points at infinity") to Euclidean points, and vice-versa. Properties meaningful for projective geometry are respected by this new idea of transformation, which is more radical in its effects than can be expressed by a transformation matrix and translations (the affine transformations). The first issue for geometers is what kind of geometry is adequate for a novel situation. It is not possible to refer to angles in projective geometry as it is in Euclidean geometry, because angle is ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Edmond Laguerre
Edmond Nicolas Laguerre (9 April 1834, Bar-le-Duc – 14 August 1886, Bar-le-Duc) was a French mathematician and a member of the Académie des sciences (1885). His main works were in the areas of geometry and complex analysis. He also investigated orthogonal polynomials (see Laguerre polynomials). Laguerre's method is a root-finding algorithm tailored to polynomials. He laid the foundations of a geometry of oriented spheres (Laguerre geometry and Laguerre plane), including the Laguerre transformation or transformation by reciprocal directions. Works Selection * * * * Théorie des équations numériques', Paris: Gauthier-Villars. 1884 on Google Books * * Oeuvres de Laguerrepubl. sous les auspices de l'Académie des sciences par MM. Charles Hermite, Henri Poincaré, et Eugène Rouché.'' (Paris, 1898-1905) (reprint: New York : Chelsea publ., 1972 ) Extensive lists More than 80 articleson Nundam.org.p See also * Isotropic line * ''q''-Laguerre polynomials * Big ''q ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Andrew Forsyth
Andrew Russell Forsyth, FRS, FRSE (18 June 1858, Glasgow – 2 June 1942, South Kensington) was a British mathematician. Life Forsyth was born in Glasgow on 18 June 1858, the son of John Forsyth, a marine engineer, and his wife Christina Glen. Forsyth studied at Liverpool College and was tutored by Richard Pendlebury before entering Trinity College, Cambridge, graduating senior wrangler in 1881. He was elected a fellow of Trinity and then appointed to the chair of mathematics at the University of Liverpool at the age of 24. He returned to Cambridge as a lecturer in 1884 and became Sadleirian Professor of Pure Mathematics in 1895. Forsyth was forced to resign his chair in 1910 as a result of a scandal caused by his affair with Marion Amelia Boys, ''née'' Pollock, the wife of physicist C. V. Boys. Boys was granted a divorce on the grounds of Marion's adultery with Forsyth. Marion and Andrew Forsyth were later married. Forsyth became professor at the Imperial College ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Ordinary Differential Equation
In mathematics, an ordinary differential equation (ODE) is a differential equation whose unknown(s) consists of one (or more) function(s) of one variable and involves the derivatives of those functions. The term ''ordinary'' is used in contrast with the term partial differential equation which may be with respect to ''more than'' one independent variable. Differential equations A linear differential equation is a differential equation that is defined by a linear polynomial in the unknown function and its derivatives, that is an equation of the form :a_0(x)y +a_1(x)y' + a_2(x)y'' +\cdots +a_n(x)y^+b(x)=0, where , ..., and are arbitrary differentiable functions that do not need to be linear, and are the successive derivatives of the unknown function of the variable . Among ordinary differential equations, linear differential equations play a prominent role for several reasons. Most elementary and special functions that are encountered in physics and applied mathematics are ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Projective Line
In mathematics, a projective line is, roughly speaking, the extension of a usual line by a point called a ''point at infinity''. The statement and the proof of many theorems of geometry are simplified by the resultant elimination of special cases; for example, two distinct projective lines in a projective plane meet in exactly one point (there is no "parallel" case). There are many equivalent ways to formally define a projective line; one of the most common is to define a projective line over a field ''K'', commonly denoted P1(''K''), as the set of one-dimensional subspaces of a two-dimensional ''K''-vector space. This definition is a special instance of the general definition of a projective space. The projective line over the reals is a manifold; see real projective line for details. Homogeneous coordinates An arbitrary point in the projective line P1(''K'') may be represented by an equivalence class of ''homogeneous coordinates'', which take the form of a pair : _1 : x_2/mat ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Projective Plane
In mathematics, a projective plane is a geometric structure that extends the concept of a plane. In the ordinary Euclidean plane, two lines typically intersect in a single point, but there are some pairs of lines (namely, parallel lines) that do not intersect. A projective plane can be thought of as an ordinary plane equipped with additional "points at infinity" where parallel lines intersect. Thus ''any'' two distinct lines in a projective plane intersect at exactly one point. Renaissance artists, in developing the techniques of drawing in perspective, laid the groundwork for this mathematical topic. The archetypical example is the real projective plane, also known as the extended Euclidean plane. This example, in slightly different guises, is important in algebraic geometry, topology and projective geometry where it may be denoted variously by , RP2, or P2(R), among other notations. There are many other projective planes, both infinite, such as the complex projective plane, ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Homogeneous Coordinates
In mathematics, homogeneous coordinates or projective coordinates, introduced by August Ferdinand Möbius in his 1827 work , are a system of coordinates used in projective geometry, just as Cartesian coordinates are used in Euclidean geometry. They have the advantage that the coordinates of points, including points at infinity, can be represented using finite coordinates. Formulas involving homogeneous coordinates are often simpler and more symmetric than their Cartesian counterparts. Homogeneous coordinates have a range of applications, including computer graphics and 3D computer vision, where they allow affine transformations and, in general, projective transformations to be easily represented by a matrix. If homogeneous coordinates of a point are multiplied by a non-zero scalar then the resulting coordinates represent the same point. Since homogeneous coordinates are also given to points at infinity, the number of coordinates required to allow this extension is one more than ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
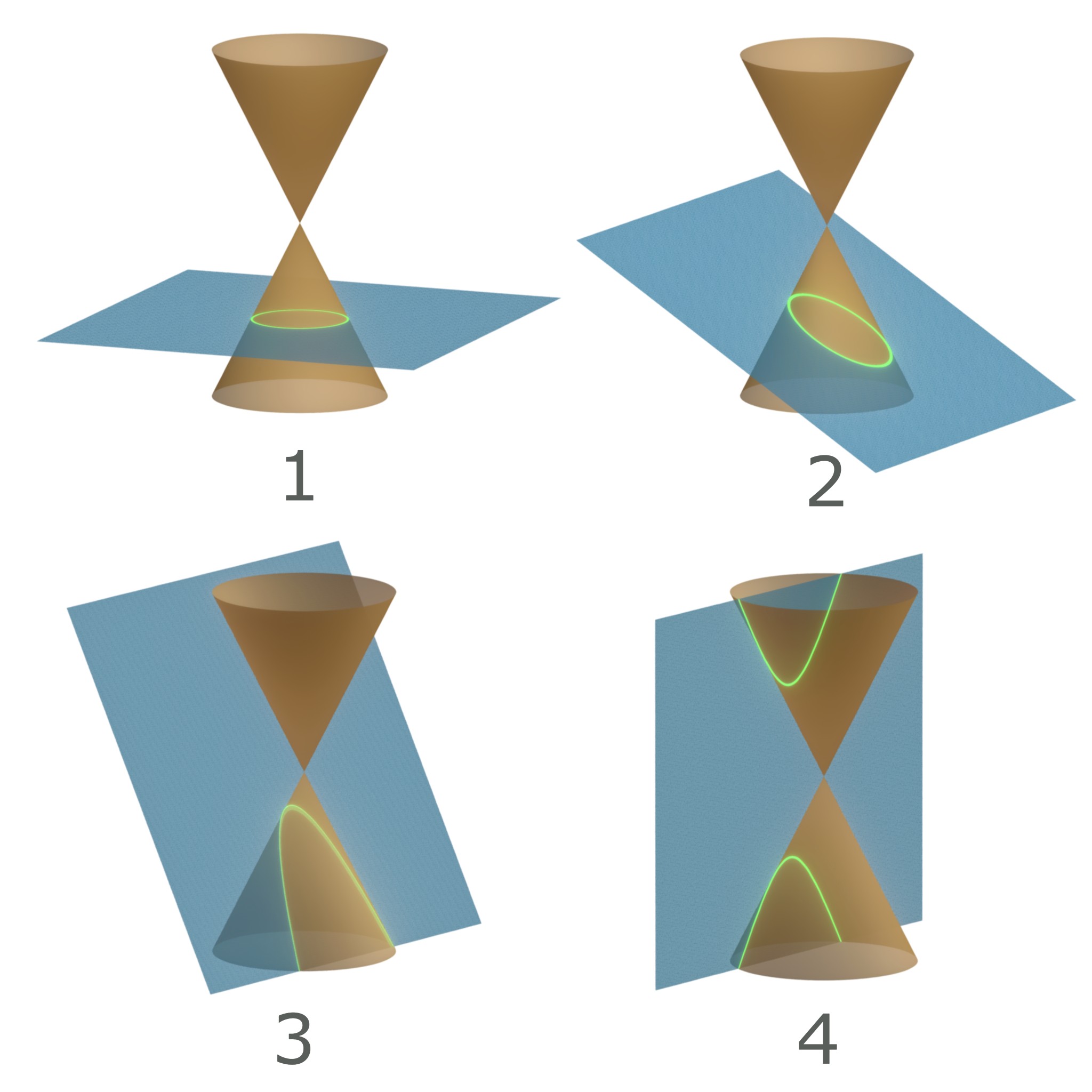
Conic Section
In mathematics, a conic section, quadratic curve or conic is a curve obtained as the intersection of the surface of a cone with a plane. The three types of conic section are the hyperbola, the parabola, and the ellipse; the circle is a special case of the ellipse, though historically it was sometimes called a fourth type. The ancient Greek mathematicians studied conic sections, culminating around 200 BC with Apollonius of Perga's systematic work on their properties. The conic sections in the Euclidean plane have various distinguishing properties, many of which can be used as alternative definitions. One such property defines a non-circular conic to be the set of those points whose distances to some particular point, called a ''focus'', and some particular line, called a ''directrix'', are in a fixed ratio, called the ''eccentricity''. The type of conic is determined by the value of the eccentricity. In analytic geometry, a conic may be defined as a plane algebraic curve of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Herglotz
Gustav Herglotz (2 February 1881 – 22 March 1953) was a German Bohemian physicist best known for his works on the theory of relativity and seismology. Biography Gustav Ferdinand Joseph Wenzel Herglotz was born in Volary num. 28 to a public notary Gustav Herglotz (also a Doctor of Law) and his wife Maria née Wachtel. The family were Sudeten Germans. He studied mathematics and astronomy at the University of Vienna in 1899, and attended lectures by Ludwig Boltzmann. In this time of study, he had a friendship with his colleagues Paul Ehrenfest, Hans Hahn and Heinrich Tietze. In 1900 he went to the LMU Munich and achieved his Doctorate in 1902 under Hugo von Seeliger. Afterwards, he went to the University of Göttingen, where he habilitated under Felix Klein. In 1904 he became Privatdozent for Astronomy and Mathematics there, and in 1907 Professor extraordinarius. In 1908 he became Professor extraordinarius in Vienna, and in 1909 at the University of Leipzig. From 1925 (until becom ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Johann Radon
Johann Karl August Radon (; 16 December 1887 – 25 May 1956) was an Austrian mathematician. His doctoral dissertation was on the calculus of variations (in 1910, at the University of Vienna). Life RadonBrigitte Bukovics: ''Biography of Johann Radon'', in: 75 Years of Radon Transform, S. Gindikin and P. Michor, eds., International Press Incorporated (1994), pp. 13–18, was born in Tetschen, Bohemia, Austria-Hungary, now Děčín, Czech Republic. He received his doctoral degree at the University of Vienna in 1910. He spent the winter semester 1910/11 at the University of Göttingen, then he was an assistant at the German Technical University in Brno, and from 1912 to 1919 at the Technical University of Vienna. In 1913/14, he passed his habilitation at the University of Vienna. Due to his near-sightedness, he was exempt from the draft during wartime. In 1919, he was called to become Professor extraordinarius at the newly founded University of Hamburg; in 1922, he became ''Prof ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |