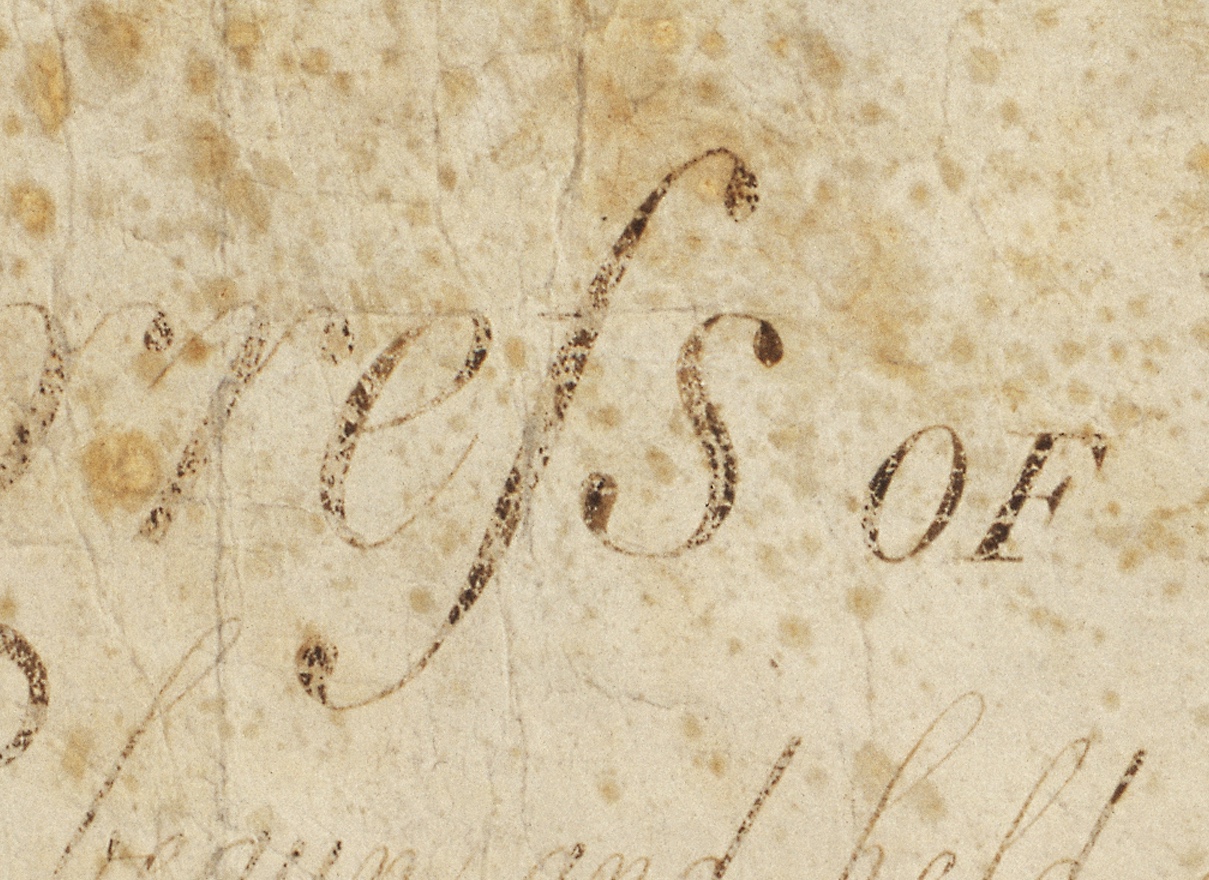|
Leibniz's Notation
In calculus, Leibniz's notation, named in honor of the 17th-century German philosopher and mathematician Gottfried Wilhelm Leibniz, uses the symbols and to represent infinitely small (or infinitesimal) increments of and , respectively, just as and represent finite increments of and , respectively. Consider as a function of a variable , or = . If this is the case, then the derivative of with respect to , which later came to be viewed as the limit :\lim_\frac = \lim_\frac, was, according to Leibniz, the quotient of an infinitesimal increment of by an infinitesimal increment of , or :\frac=f'(x), where the right hand side is Joseph-Louis Lagrange's notation for the derivative of at . The infinitesimal increments are called . Related to this is the integral in which the infinitesimal increments are summed (e.g. to compute lengths, areas and volumes as sums of tiny pieces), for which Leibniz also supplied a closely related notation involving the same differentials, ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Gottfried Wilhelm Leibniz C1700
Gottfried is a masculine German given name. It is derived from the Old High German name , recorded since the 7th century. The name is composed of the elements (conflated from the etyma for 'God' and 'good', and possibly further conflated with ) and ('peace, protection'). The German name was commonly hypocoristically abbreviated as ''Götz'' from the late medieval period. ''Götz'' and variants (including '' Göthe, Göthke'' and ''Göpfert'') also came into use as German surnames. Gottfried is a common Jewish surname as well. Given name The given name ''Gottfried'' became extremely frequent in Germany in the High Middle Ages, to the point of eclipsing most other names in ''God-'' (such as ''Godabert, Gotahard, Godohelm, Godomar, Goduin, Gotrat, Godulf'', etc.) The name was Latinised as ''Godefridus''. Medieval bearers of the name include: *Gotfrid, Duke of Alemannia and Raetia (d. 709) *Godefrid (d. c. 720), son of Drogo of Champagne, Frankish nobleman. *Godfrid Haraldsson ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Differential Forms
In mathematics, differential forms provide a unified approach to define integrands over curves, surfaces, solids, and higher-dimensional manifolds. The modern notion of differential forms was pioneered by Élie Cartan. It has many applications, especially in geometry, topology and physics. For instance, the expression is an example of a -form, and can be integrated over an interval contained in the domain of : :\int_a^b f(x)\,dx. Similarly, the expression is a -form that can be integrated over a surface : :\int_S (f(x,y,z)\,dx\wedge dy + g(x,y,z)\,dz\wedge dx + h(x,y,z)\,dy\wedge dz). The symbol denotes the exterior product, sometimes called the ''wedge product'', of two differential forms. Likewise, a -form represents a volume element that can be integrated over a region of space. In general, a -form is an object that may be integrated over a -dimensional manifold, and is homogeneous of degree in the coordinate differentials dx, dy, \ldots. On an -dimensional manifold, t ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Mathematical Notation
Mathematical notation consists of using symbols for representing operations, unspecified numbers, relations and any other mathematical objects, and assembling them into expressions and formulas. Mathematical notation is widely used in mathematics, science, and engineering for representing complex concepts and properties in a concise, unambiguous and accurate way. For example, Albert Einstein's equation E=mc^2 is the quantitative representation in mathematical notation of the mass–energy equivalence. Mathematical notation was first introduced by François Viète at the end of the 16th century, and largely expanded during the 17th and 18th century by René Descartes, Isaac Newton, Gottfried Wilhelm Leibniz, and overall Leonhard Euler. Symbols The use of many symbols is the basis of mathematical notation. They play a similar role as words in natural languages. They may play different roles in mathematical notation similarly as verbs, adjective and nouns play different roles in ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Dependent And Independent Variables
Dependent and independent variables are variables in mathematical modeling, statistical modeling and experimental sciences. Dependent variables receive this name because, in an experiment, their values are studied under the supposition or demand that they depend, by some law or rule (e.g., by a mathematical function), on the values of other variables. Independent variables, in turn, are not seen as depending on any other variable in the scope of the experiment in question. In this sense, some common independent variables are time, space, density, mass, fluid flow rate, and previous values of some observed value of interest (e.g. human population size) to predict future values (the dependent variable). Of the two, it is always the dependent variable whose variation is being studied, by altering inputs, also known as regressors in a statistical context. In an experiment, any variable that can be attributed a value without attributing a value to any other variable is called an in ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Dimensional Analysis
In engineering and science, dimensional analysis is the analysis of the relationships between different physical quantities by identifying their base quantities (such as length, mass, time, and electric current) and units of measure (such as miles vs. kilometres, or pounds vs. kilograms) and tracking these dimensions as calculations or comparisons are performed. The conversion of units from one dimensional unit to another is often easier within the metric or the SI than in others, due to the regular 10-base in all units. ''Commensurable'' physical quantities are of the same kind and have the same dimension, and can be directly compared to each other, even if they are expressed in differing units of measure, e.g. yards and metres, pounds (mass) and kilograms, seconds and years. ''Incommensurable'' physical quantities are of different kinds and have different dimensions, and can not be directly compared to each other, no matter what units they are expressed in, e.g. metres and ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Separation Of Variables
In mathematics, separation of variables (also known as the Fourier method) is any of several methods for solving ordinary and partial differential equations, in which algebra allows one to rewrite an equation so that each of two variables occurs on a different side of the equation. Ordinary differential equations (ODE) Suppose a differential equation can be written in the form :\frac f(x) = g(x)h(f(x)) which we can write more simply by letting y = f(x): :\frac=g(x)h(y). As long as ''h''(''y'') ≠ 0, we can rearrange terms to obtain: : = g(x) \, dx, so that the two variables ''x'' and ''y'' have been separated. ''dx'' (and ''dy'') can be viewed, at a simple level, as just a convenient notation, which provides a handy mnemonic aid for assisting with manipulations. A formal definition of ''dx'' as a differential (infinitesimal) is somewhat advanced. Alternative notation Those who dislike Leibniz's notation may prefer to write this as :\frac \frac = g(x), but that ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Cours D'Analyse
''Cours d'Analyse de l’École Royale Polytechnique; I.re Partie. Analyse algébrique'' is a seminal textbook in infinitesimal calculus published by Augustin-Louis Cauchy in 1821. The article follows the translation by Bradley and Sandifer in describing its contents. Introduction On page 1 of the Introduction, Cauchy writes: "In speaking of the continuity of functions, I could not dispense with a treatment of the principal properties of infinitely small quantities, properties which serve as the foundation of the infinitesimal calculus." The translators comment in a footnote: "It is interesting that Cauchy does not also mention limits here." Cauchy continues: "As for the methods, I have sought to give them all the rigor which one demands from geometry, so that one need never rely on arguments drawn from the generality of algebra." Preliminaries On page 6, Cauchy first discusses variable quantities and then introduces the limit notion in the following terms: "When the values ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Nova Methodus Pro Maximis Et Minimis
"Nova Methodus pro Maximis et Minimis" is the first published work on the subject of calculus. It was published by Gottfried Leibniz in the ''Acta Eruditorum'' in October 1684. It is considered to be the birth of infinitesimal calculus. Full title The full title of the published work is "Nova methodus pro maximis et minimis, itemque tangentibus, quae nec fractas nec irrationales quantitates moratur, et singulare pro illis calculi genus." In English, the full title can be translated as "A new method for maxima and minima, and for tangents, that is not hindered by fractional or irrational quantities, and a singular kind of calculus for the above mentioned." It is from this title that this branch of mathematics takes the name calculus. Influence Although calculus was independently co-invented by Isaac Newton, most of the notation in modern calculus is from Leibniz. Leibniz's careful attention to his notation makes some believe that "his contribution to calculus was much more influen ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Mathematical Association Of America
The Mathematical Association of America (MAA) is a professional society that focuses on mathematics accessible at the undergraduate level. Members include university, college, and high school teachers; graduate and undergraduate students; pure and applied mathematicians; computer scientists; statisticians; and many others in academia, government, business, and industry. The MAA was founded in 1915 and is headquartered at 1529 18th Street, Northwest in the Dupont Circle neighborhood of Washington, D.C. The organization publishes mathematics journals and books, including the '' American Mathematical Monthly'' (established in 1894 by Benjamin Finkel), the most widely read mathematics journal in the world according to records on JSTOR. Mission and Vision The mission of the MAA is to advance the understanding of mathematics and its impact on our world. We envision a society that values the power and beauty of mathematics and fully realizes its potential to promote human flourishing ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Acta Eruditorum
(from Latin: ''Acts of the Erudite'') was the first scientific journal of the German-speaking lands of Europe, published from 1682 to 1782. History ''Acta Eruditorum'' was founded in 1682 in Leipzig by Otto Mencke, who became its first editor, with support from Gottfried Leibniz in Hanover, who contributed 13 articles over the journal's first four years. It was published by Johann Friedrich Gleditsch, with sponsorship from the Duke of Saxony, and was patterned after the French ''Journal des savants'' and the Italian ''Giornale de'letterati''. The journal was published monthly, entirely in Latin, and contained excerpts from new writings, reviews, small essays and notes. Most of the articles were devoted to the natural sciences and mathematics, including contributions (apart from Leibniz) from, e.g., Jakob Bernoulli, Humphry Ditton, Leonhard Euler, Ehrenfried Walther von Tschirnhaus, Pierre-Simon Laplace and Jérôme Lalande, but also from humanists and philosophers such as Veit ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Integral Sign
The integral symbol: : (Unicode), \displaystyle \int (LaTeX) is used to denote integrals and antiderivatives in mathematics, especially in calculus. History The notation was introduced by the German mathematician Gottfried Wilhelm Leibniz in 1675 in his private writings; it first appeared publicly in the article "" (On a hidden geometry and analysis of indivisibles and infinites), published in ''Acta Eruditorum'' in June 1686. The symbol was based on the ſ (long s) character and was chosen because Leibniz thought of the integral as an infinite sum of infinitesimal summands. Typography in Unicode and LaTeX Fundamental symbol The integral symbol is in Unicode and \int in LaTeX. In HTML, it is written as ∫ (hexadecimal), ∫ (decimal) and ∫ (named entity). The original IBM PC code page 437 character set included a couple of characters ⌠ and ⌡ (codes 244 and 245 respectively) to build the integral symbol. These were deprecated in subsequent ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Long S
The long s , also known as the medial s or initial s, is an archaism, archaic form of the lowercase letter . It replaced the single ''s'', or one or both of the letters ''s'' in a 'double ''s''' sequence (e.g., "ſinfulneſs" for "sinfulness" and "poſſeſs" or "poſseſs" for "possess"—but never asterisk#Ungrammaticality, *"poſſeſſ"). The modern letterform is known as the 'short', 'terminal', or 'round' s. In typography, it is known as a type of swash letter, commonly referred to as a "swash s". The long s is the basis of the first half of the grapheme of the German alphabet Orthographic ligature, ligature letter , (''eszett'' or [sharp s]). Rules This list of rules for the long s is not exhaustive, and it applies only to books printed during the 17th and 18th centuries in English-speaking countries. Similar rules exist for other European languages. * A round s is always used at the end of a word ending with s: "his", "complains", "ſucceſs" ** However, long s is m ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
