|
Lamb–Chaplygin Dipole
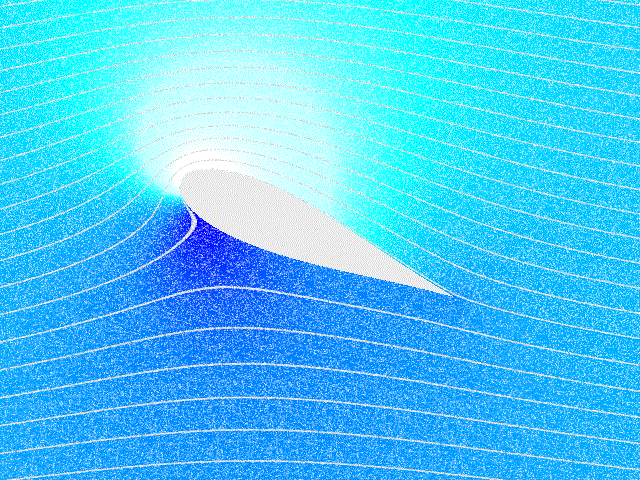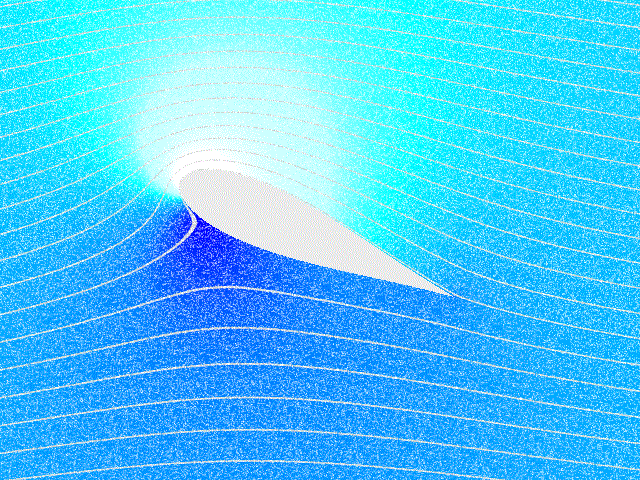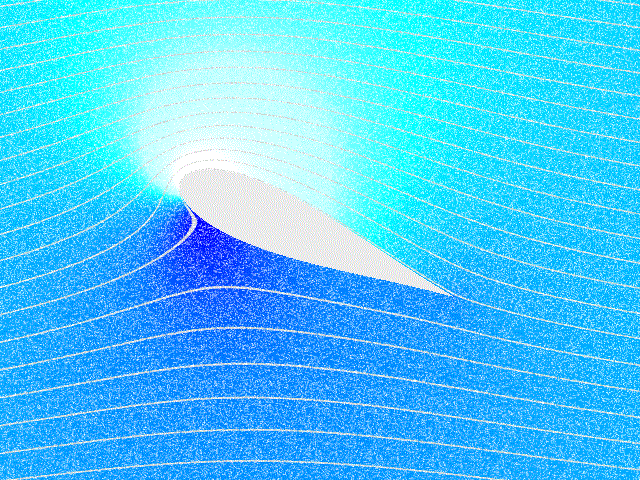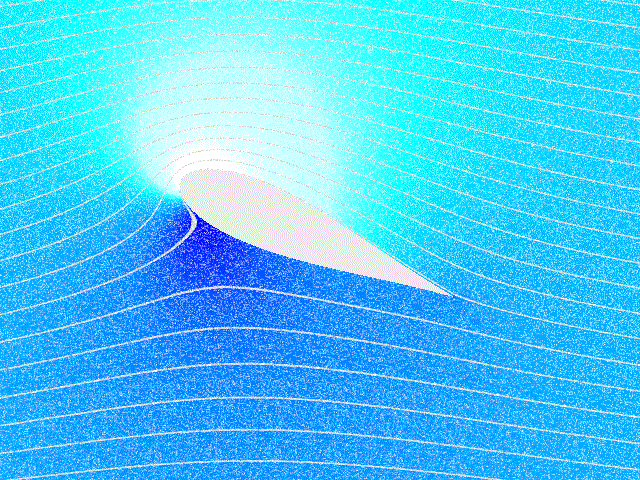
The Lamb–Chaplygin dipole model is a mathematical description for a particular inviscid and steady dipolar vortex flow. It is a non-trivial solution to the two-dimensional Euler equations. The model is named after Horace Lamb and Sergey Alexeyevich Chaplygin, who independently discovered this flow structure. This dipole is the two-dimensional analogue of Hill's spherical vortex. __TOC__ The model A two-dimensional (2D), solenoidal vector field \mathbf may be described by a scalar stream function \psi, via \mathbf = -\mathbf \times \mathbf \psi, where \mathbf is the right-handed unit vector perpendicular to the 2D plane. By definition, the stream function is related to the vorticity \omega via a Poisson equation: -\nabla^2\psi = \omega. The Lamb–Chaplygin model follows from demanding the following characteristics: * The dipole has a circular atmosphere/separatrix with radius R: \psi\left(r = R\right) = 0. * The dipole propages through an otherwise irrorational fluid (\ome ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Euler Equations (fluid Dynamics)
In fluid dynamics, the Euler equations are a set of quasilinear partial differential equations governing adiabatic and inviscid flow. They are named after Leonhard Euler. In particular, they correspond to the Navier–Stokes equations with zero viscosity and zero thermal conductivity. The Euler equations can be applied to incompressible or compressible flow. The incompressible Euler equations consist of Cauchy equations for conservation of mass and balance of momentum, together with the incompressibility condition that the flow velocity is a solenoidal field. The compressible Euler equations consist of equations for conservation of mass, balance of momentum, and balance of energy, together with a suitable constitutive equation for the specific energy density of the fluid. Historically, only the equations of conservation of mass and balance of momentum were derived by Euler. However, fluid dynamics literature often refers to the full set of the compressible Euler equations – ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Horace Lamb
Sir Horace Lamb (27 November 1849 – 4 December 1934)R. B. Potts,, ''Australian Dictionary of Biography'', Volume 5, MUP, 1974, pp 54–55. Retrieved 5 Sep 2009 was a British applied mathematician and author of several influential texts on classical physics, among them ''Hydrodynamics'' (1895) and ''Dynamical Theory of Sound'' (1910). Both of these books remain in print. The word vorticity was coined by Lamb in 1916. Biography Early life and education Lamb was born in Stockport, Cheshire, the son of John Lamb and his wife Elizabeth, ''née'' Rangeley. John Lamb was a foreman in a cotton mill who had gained some distinction by the invention of an improvement to spinning machines, he died when his son was a child. Lamb's mother married again, and shortly afterwards Horace went to live with his strict but maternal aunt, Mrs. Holland. He studied at Stockport Grammar School, where he made the acquaintance of a wise and kindly headmaster in the Rev. Charles Hamilton, and a gradu ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Sergey Alexeyevich Chaplygin
Sergey Alexeyevich Chaplygin (russian: Серге́й Алексе́евич Чаплы́гин; 5 April 1869 – 8 October 1942) was a Russian and USSR, Soviet physicist, mathematician, and mechanical engineer. He is known for mathematical formulas such as Chaplygin's equation and for a hypothetical substance in cosmology called Chaplygin gas, named after him. He graduated in 1890 from Moscow University, and later became a professor. He taught mechanical engineering at Moscow Higher Courses for Women in 1901, and of applied mathematics at Moscow School of Technology, 1903. He was appointed Director of the courses in 1905. Leonid I. Sedov was one of his students. Chaplygin's theories were greatly inspired by Nikolay Yegorovich Zhukovsky, N. Ye. Zhukovsky, who founded the TsAGI, Central Institute of Aerodynamics. His early research consisted of hydromechanics. His "Collected Works" in four volumes were published in 1948. Honours and awards * Hero of Socialist L ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hill's Spherical Vortex
Hill's spherical vortex is an exact solution of the Euler equations that is commonly used to model a vortex ring. The solution is also used to model the velocity distribution inside a spherical drop of one fluid moving at a constant velocity through another fluid at small Reynolds number. The vortex is named after Micaiah John Muller Hill who discovered the exact solution in 1894. The two-dimensional analogue of this vortex is the Lamb–Chaplygin dipole. The solution is described in the spherical polar coordinates system (r,\theta,\phi) with corresponding velocity components (v_r,v_\theta,0). The velocity components are identified from Stokes stream function \psi(r,\theta) as follows :v_r = \frac\frac, \quad v_\theta = - \frac\frac. The Hill's spherical vortex is described by : \psi=\begin-\frac \left(1-\frac\right) r^2\sin^2\theta \quad \text \quad r\leq a\\ \frac \left(1 - \frac\right)r^2\sin^2\theta \quad \text \quad r\geq a \end where U is a constant freestream velocity fa ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Solenoidal Vector Field
In vector calculus a solenoidal vector field (also known as an incompressible vector field, a divergence-free vector field, or a Helmholtz decomposition#Longitudinal and transverse fields, transverse vector field) is a vector field v with divergence zero at all points in the field: \nabla \cdot \mathbf = 0. A common way of expressing this property is to say that the field has no sources or sinks.This statement does not mean that the field lines of a solenoidal field must be closed, neither that they cannot begin or end. For a detailed discussion of the subject, see J. Slepian: "Lines of Force in Electric and Magnetic Fields", American Journal of Physics, vol. 19, pp. 87-90, 1951, and L. Zilberti: "The Misconception of Closed Magnetic Flux Lines", IEEE Magnetics Letters, vol. 8, art. 1306005, 2017. Properties The divergence theorem gives an equivalent integral definition of a solenoidal field; namely that for any closed surface, the net total flux through the surface must be zero ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Stream Function
The stream function is defined for incompressible flow, incompressible (divergence-free) fluid flow, flows in two dimensions – as well as in three dimensions with axisymmetry. The flow velocity components can be expressed as the derivatives of the scalar field, scalar stream function. The stream function can be used to plot Streamlines, streaklines, and pathlines, streamlines, which represent the trajectories of particles in a steady flow. The two-dimensional Lagrange stream function was introduced by Joseph Louis Lagrange in 1781. The Stokes stream function is for axisymmetrical three-dimensional flow, and is named after George Gabriel Stokes. Considering the particular case of fluid dynamics, the difference between the stream function values at any two points gives the volumetric flow rate (or volumetric flux) through a line connecting the two points. Since streamlines are tangent to the flow velocity vector of the flow, the value of the stream function must be constant along ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Vorticity
In continuum mechanics, vorticity is a pseudovector field that describes the local spinning motion of a continuum near some point (the tendency of something to rotate), as would be seen by an observer located at that point and traveling along with the flow. It is an important quantity in the dynamical theory of fluids and provides a convenient framework for understanding a variety of complex flow phenomena, such as the formation and motion of vortex rings. Mathematically, the vorticity \vec is the curl of the flow velocity \vec: :\vec \equiv \nabla \times \vec\,, where \nabla is the nabla operator. Conceptually, \vec could be determined by marking parts of a continuum in a small neighborhood of the point in question, and watching their ''relative'' displacements as they move along the flow. The vorticity \vec would be twice the mean angular velocity vector of those particles relative to their center of mass, oriented according to the right-hand rule. In a two-dimensional fl ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Poisson Equation
Poisson's equation is an elliptic partial differential equation of broad utility in theoretical physics. For example, the solution to Poisson's equation is the potential field caused by a given electric charge or mass density distribution; with the potential field known, one can then calculate electrostatic or gravitational (force) field. It is a generalization of Laplace's equation, which is also frequently seen in physics. The equation is named after French mathematician and physicist Siméon Denis Poisson. Statement of the equation Poisson's equation is \Delta\varphi = f where \Delta is the Laplace operator, and f and \varphi are real or complex-valued functions on a manifold. Usually, f is given and \varphi is sought. When the manifold is Euclidean space, the Laplace operator is often denoted as and so Poisson's equation is frequently written as \nabla^2 \varphi = f. In three-dimensional Cartesian coordinates, it takes the form \left( \frac + \frac + \frac \right)\varph ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Cylindrical Coordinates
A cylindrical coordinate system is a three-dimensional coordinate system that specifies point positions by the distance from a chosen reference axis ''(axis L in the image opposite)'', the direction from the axis relative to a chosen reference direction ''(axis A)'', and the distance from a chosen reference plane perpendicular to the axis ''(plane containing the purple section)''. The latter distance is given as a positive or negative number depending on which side of the reference plane faces the point. The ''origin'' of the system is the point where all three coordinates can be given as zero. This is the intersection between the reference plane and the axis. The axis is variously called the ''cylindrical'' or ''longitudinal'' axis, to differentiate it from the ''polar axis'', which is the ray that lies in the reference plane, starting at the origin and pointing in the reference direction. Other directions perpendicular to the longitudinal axis are called ''radial lines''. The ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Bessel Functions
Bessel functions, first defined by the mathematician Daniel Bernoulli and then generalized by Friedrich Bessel, are canonical solutions of Bessel's differential equation x^2 \frac + x \frac + \left(x^2 - \alpha^2 \right)y = 0 for an arbitrary complex number \alpha, the ''order'' of the Bessel function. Although \alpha and -\alpha produce the same differential equation, it is conventional to define different Bessel functions for these two values in such a way that the Bessel functions are mostly smooth functions of \alpha. The most important cases are when \alpha is an integer or half-integer. Bessel functions for integer \alpha are also known as cylinder functions or the cylindrical harmonics because they appear in the solution to Laplace's equation in cylindrical coordinates. Spherical Bessel functions with half-integer \alpha are obtained when the Helmholtz equation is solved in spherical coordinates. Applications of Bessel functions The Bessel function is a generalization ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |