|
Knot Operation
In knot theory, a knot move or operation is a change or changes which preserve crossing number. Operations are used to investigate whether knots are equivalent, prime or reduced. Knot moves or operations include the flype, Habiro move, Markov moves (I. conjugation and II. stabilization), pass move, Perko move, and Reidemeister moves (I. twist move, II. poke move, and III. slide move). See also *Knot sum *Mutation (knot theory) In the mathematical field of knot theory, a mutation is an operation on a knot that can produce different knots. Suppose ''K'' is a knot given in the form of a knot diagram. Consider a disc ''D'' in the projection plane of the diagram whose bo ... References {{Knottheory-stub ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Knot Theory
In the mathematical field of topology, knot theory is the study of knot (mathematics), mathematical knots. While inspired by knots which appear in daily life, such as those in shoelaces and rope, a mathematical knot differs in that the ends are joined so it cannot be undone, Unknot, the simplest knot being a ring (or "unknot"). In mathematical language, a knot is an embedding of a circle in 3-dimensional Euclidean space, \mathbb^3 (in topology, a circle is not bound to the classical geometric concept, but to all of its homeomorphisms). Two mathematical knots are equivalent if one can be transformed into the other via a deformation of \mathbb^3 upon itself (known as an ambient isotopy); these transformations correspond to manipulations of a knotted string that do not involve cutting it or passing through itself. Knots can be described in various ways. Using different description methods, there may be more than one description of the same knot. For example, a common method of descr ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Crossing Number (knot Theory)
In the mathematical area of knot theory, the crossing number of a knot is the smallest number of crossings of any diagram of the knot. It is a knot invariant. Examples By way of example, the unknot has crossing number zero, the trefoil knot three and the figure-eight knot four. There are no other knots with a crossing number this low, and just two knots have crossing number five, but the number of knots with a particular crossing number increases rapidly as the crossing number increases. Tabulation Tables of prime knots are traditionally indexed by crossing number, with a subscript to indicate which particular knot out of those with this many crossings is meant (this sub-ordering is not based on anything in particular, except that torus knots then twist knots are listed first). The listing goes 31 (the trefoil knot), 41 (the figure-eight knot), 51, 52, 61, etc. This order has not changed significantly since P. G. Tait published a tabulation of knots in 1877. Additivity There ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
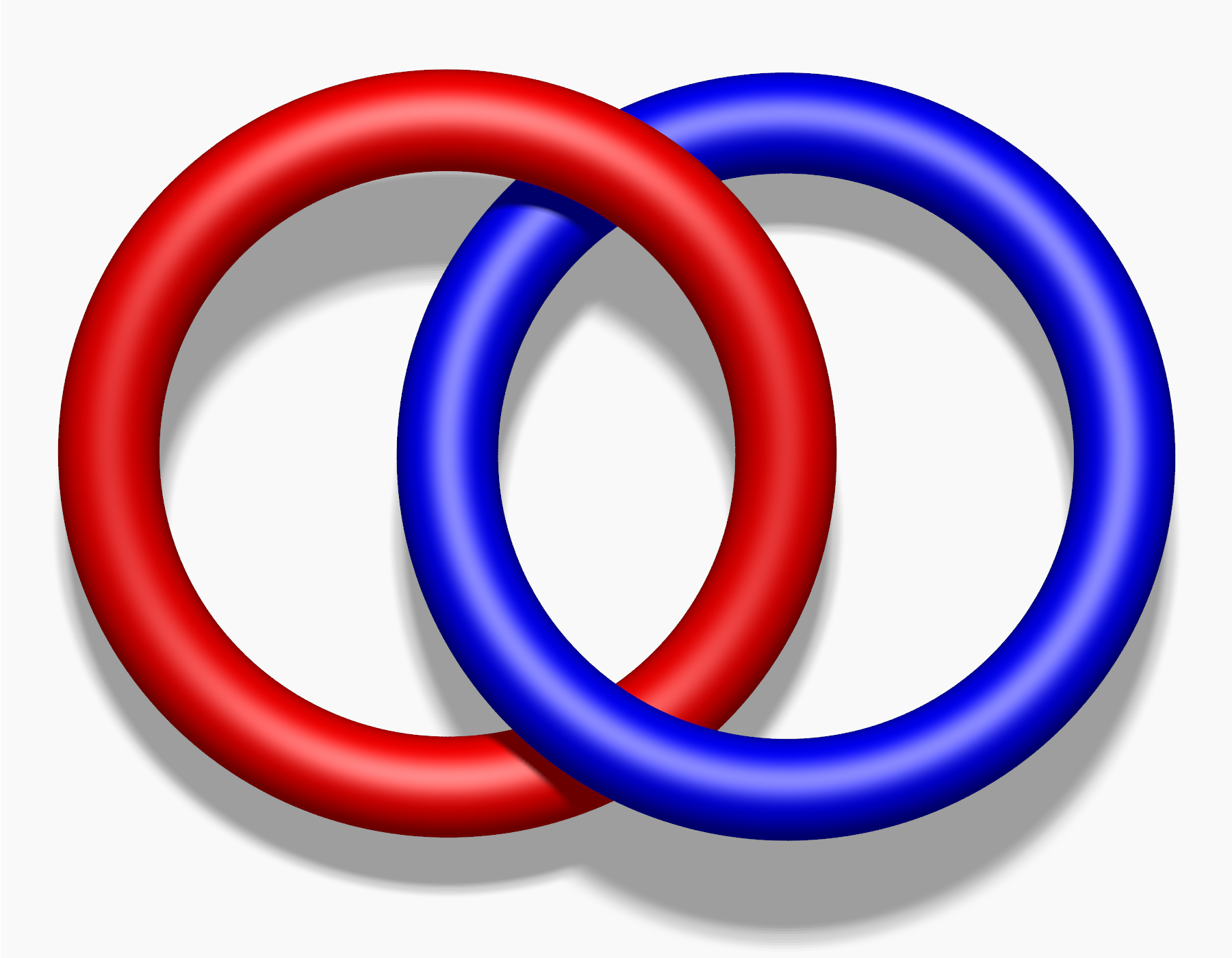
Prime Knot
In knot theory, a prime knot or prime link is a knot that is, in a certain sense, indecomposable. Specifically, it is a non-trivial knot which cannot be written as the knot sum of two non-trivial knots. Knots that are not prime are said to be composite knots or composite links. It can be a nontrivial problem to determine whether a given knot is prime or not. A family of examples of prime knots are the torus knots. These are formed by wrapping a circle around a torus ''p'' times in one direction and ''q'' times in the other, where ''p'' and ''q'' are coprime integers. Knots are characterized by their crossing numbers. The simplest prime knot is the trefoil with three crossings. The trefoil is actually a (2, 3)-torus knot. The figure-eight knot, with four crossings, is the simplest non-torus knot. For any positive integer ''n'', there are a finite number of prime knots with ''n'' crossings. The first few values are given in the following table. : Enantiomorphs are counted ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Nugatory Crossing
In the mathematical field of topology, knot theory is the study of mathematical knots. While inspired by knots which appear in daily life, such as those in shoelaces and rope, a mathematical knot differs in that the ends are joined so it cannot be undone, the simplest knot being a ring (or "unknot"). In mathematical language, a knot is an embedding of a circle in 3-dimensional Euclidean space, \mathbb^3 (in topology, a circle is not bound to the classical geometric concept, but to all of its homeomorphisms). Two mathematical knots are equivalent if one can be transformed into the other via a deformation of \mathbb^3 upon itself (known as an ambient isotopy); these transformations correspond to manipulations of a knotted string that do not involve cutting it or passing through itself. Knots can be described in various ways. Using different description methods, there may be more than one description of the same knot. For example, a common method of describing a knot is a planar di ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Flype
In the mathematical theory of knots, a flype is a kind of manipulation of knot and link diagrams used in the Tait flyping conjecture. It consists of twisting a part of a knot, a tangle T, by 180 degrees. Flype comes from a Scots word meaning ''to fold'' or ''to turn back'' ("as with a sock").. Tait used the term to mean, "a change of infinite complementary region"). Two reduced alternating diagrams of an alternating link can be transformed to each other using flypes. This is the Tait flyping conjecture, proven in 1991 by Morwen Thistlethwaite and William Menasco. See also * Reidemeister move Kurt Werner Friedrich Reidemeister (13 October 1893 – 8 July 1971) was a mathematician born in Braunschweig (Brunswick), Germany. Life He was a brother of Marie Neurath. Beginning in 1912, he studied in Freiburg, Munich, Marburg, and Göttinge ...s are another commonly studied kind of manipulation to knot diagrams. References Knot operations {{knottheory-stub ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Clasper (mathematics)
In the mathematical field of low-dimensional topology, a clasper is a surface (with extra structure) in a 3-manifold on which surgery can be performed. Motivation Beginning with the Jones polynomial, infinitely many new invariants of knots, links, and 3-manifolds were found during the 1980s. The study of these new `quantum' invariants expanded rapidly into a sub-discipline of low-dimensional topology called quantum topology. A quantum invariant is typically constructed from two ingredients: a formal sum of Jacobi diagrams (which carry a Lie algebra structure), and a representation of a ribbon Hopf algebra such as a quantum group. It is not clear a-priori why either of these ingredients should have anything to do with low-dimensional topology. Thus one of the main problems in quantum topology has been to interpret quantum invariants topologically. The theory of claspers comes to provide such an interpretation. A clasper, like a framed link, is an embedded topological object ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Arf Invariant Of A Knot
In the mathematical field of knot theory, the Arf invariant of a knot, named after Cahit Arf, is a knot invariant obtained from a quadratic form associated to a Seifert surface. If ''F'' is a Seifert surface of a knot, then the homology group has a quadratic form whose value is the number of full twists mod 2 in a neighborhood of an embedded circle representing an element of the homology group. The Arf invariant of this quadratic form is the Arf invariant of the knot. Definition by Seifert matrix Let V = v_ be a Seifert matrix of the knot, constructed from a set of curves on a Seifert surface of genus ''g'' which represent a basis for the first homology of the surface. This means that ''V'' is a matrix with the property that is a symplectic matrix. The ''Arf invariant'' of the knot is the residue of :\sum\limits^g_ v_ v_ \pmod 2. Specifically, if \, i = 1 \ldots g, is a symplectic basis for the intersection form on the Seifert surface, then :\operatorname(K) = \sum\limit ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Perko Pair
In the mathematical theory of knots, the Perko pair, named after Kenneth Perko, is a pair of entries in classical knot tables that actually represent the same knot. In Dale Rolfsen's knot table, this supposed pair of distinct knots is labeled 10161 and 10162. In 1973, while working to complete the classification by knot type of the Tait–Little knot tables of knots up to 10 crossings (dating from the late 19th century), Perko found the duplication in Charles Newton Little's table. This duplication had been missed by John Horton Conway several years before in his knot table and subsequently found its way into Rolfsen's table. The Perko pair gives a counterexample to a "theorem" claimed by Little in 1900 that the writhe In knot theory, there are several competing notions of the quantity writhe, or \operatorname. In one sense, it is purely a property of an oriented link diagram and assumes integer values. In another sense, it is a quantity that describes the amou ... of a red ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Reidemeister Move
Kurt Werner Friedrich Reidemeister (13 October 1893 – 8 July 1971) was a mathematician born in Braunschweig (Brunswick), Germany. Life He was a brother of Marie Neurath. Beginning in 1912, he studied in Freiburg, Munich, Marburg, and Göttingen. In 1920, he got the staatsexamen (master's degree) in mathematics, philosophy, physics, chemistry, and geology. He received his doctorate in 1921 with a thesis in algebraic number theory at the University of Hamburg under the supervision of Erich Hecke. He became interested in differential geometry; he edited Wilhelm Blaschke's 2nd volume about that issue, and both made an acclaimed contribution to the Jena DMV conference in Sep 1921. In October 1922 (or 1923) he was appointed assistant professor at the University of Vienna. While there he became familiar with the work of Wilhelm Wirtinger on knot theory, and became closely connected to Hans Hahn and the Vienna Circle. Its manifesto (1929) lists one of Reidemeister's publications i ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Knot Sum
In mathematics, specifically in topology, the operation of connected sum is a geometric modification on manifolds. Its effect is to join two given manifolds together near a chosen point on each. This construction plays a key role in the classification of closed surfaces. More generally, one can also join manifolds together along identical submanifolds; this generalization is often called the fiber sum. There is also a closely related notion of a connected sum on knots, called the knot sum or composition of knots. Connected sum at a point A connected sum of two ''m''-dimensional manifolds is a manifold formed by deleting a ball inside each manifold and gluing together the resulting boundary spheres. If both manifolds are oriented, there is a unique connected sum defined by having the gluing map reverse orientation. Although the construction uses the choice of the balls, the result is unique up to homeomorphism. One can also make this operation work in the smooth category, a ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Mutation (knot Theory)
In the mathematical field of knot theory, a mutation is an operation on a knot that can produce different knots. Suppose ''K'' is a knot given in the form of a knot diagram. Consider a disc ''D'' in the projection plane of the diagram whose boundary circle intersects ''K'' exactly four times. We may suppose that (after planar isotopy) the disc is geometrically round and the four points of intersection on its boundary with ''K'' are equally spaced. The part of the knot inside the disc is a tangle. There are two reflections that switch pairs of endpoints of the tangle. There is also a rotation that results from composition of the reflections. A mutation replaces the original tangle by a tangle given by any of these operations. The result will always be a knot and is called a mutant of ''K''. Mutants can be difficult to distinguish as they have a number of the same invariants. They have the same hyperbolic volume (by a result of Ruberman), and have the same HOMFLY polynomial In th ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |