|
Khatri–Rao Product
In mathematics, the Khatri–Rao product of matrices defined as : \mathbf \ast \mathbf = \left(\mathbf_ \otimes \mathbf_\right)_ in which the ''ij''-th block is the sized Kronecker product of the corresponding blocks of A and B, assuming the number of row and column partitions of both matrices is equal. The size of the product is then . For example, if A and B both are partitioned matrices e.g.: : \mathbf = \left \begin \mathbf_ & \mathbf_ \\ \hline \mathbf_ & \mathbf_ \end \right= \left \begin 1 & 2 & 3 \\ 4 & 5 & 6 \\ \hline 7 & 8 & 9 \end \right,\quad \mathbf = \left \begin \mathbf_ & \mathbf_ \\ \hline \mathbf_ & \mathbf_ \end \right= \left \begin 1 & 4 & 7 \\ \hline 2 & 5 & 8 \\ 3 & 6 & 9 \end \right, we obtain: : \mathbf \ast \mathbf = \left \begin \mathbf_ \otimes \mathbf_ & \mathbf_ \otimes \mathbf_ \\ \hline \mathbf_ \otimes \mathbf_ & \mathbf_ \otimes \mathbf_ \end \right= \left \begin 1 & 2 & 12 & 21 \\ 4 & 5 & 24 & 42 \\ \hline 14 & 16 & 45 & 72 \\ 21 & ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Sankhya (journal)
''Sankhyā: The Indian Journal of Statistics'' is a quarterly peer-reviewed scientific journal on statistics published by the Indian Statistical Institute (ISI). It was established in 1933 by Prasanta Chandra Mahalanobis, founding director of ISI, along the lines of Karl Pearson's ''Biometrika''. Mahalanobis was the founding editor-in-chief. Each volume of ''Sankhya'' consists of four issues, two of them are in Series A, containing articles on theoretical statistics, probability theory, and stochastic processes, whereas the other two issues form Series B, containing articles on applied statistics, i.e. applied probability, applied stochastic processes, econometrics, and statistical computing. ''Sankhya'' is considered as "core journal" of statistics by the Current Index to Statistics. Publication history ''Sankhya'' was first published in June 1933. In 1961, the journal split into two series: Series A which focused on mathematical statistics and Series B which focused on st ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Count Sketch
Count sketch is a type of dimensionality reduction that is particularly efficient in statistics, machine learning and algorithms. It was invented by Moses Charikar, Kevin Chen and Martin Farach-Colton in an effort to speed up the AMS Sketch by Alon, Matias and Szegedy for approximating the frequency moments of streams. The sketch is nearly identical to the Feature hashing algorithm by John Moody, but differs in its use of hash functions with low dependence, which makes it more practical. In order to still have a high probability of success, the median trick is used to aggregate multiple count sketches, rather than the mean. These properties allow use for explicit kernel methods, bilinear pooling in neural networks and is a cornerstone in many numerical linear algebra algorithms.Woodruff, David P. "Sketching as a Tool for Numerical Linear Algebra." Theoretical Computer Science 10.1-2 (2014): 1–157. Mathematical definition 1. For constants w and t (to be defined later) ind ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Statistics
Statistics (from German: '' Statistik'', "description of a state, a country") is the discipline that concerns the collection, organization, analysis, interpretation, and presentation of data. In applying statistics to a scientific, industrial, or social problem, it is conventional to begin with a statistical population or a statistical model to be studied. Populations can be diverse groups of people or objects such as "all people living in a country" or "every atom composing a crystal". Statistics deals with every aspect of data, including the planning of data collection in terms of the design of surveys and experiments.Dodge, Y. (2006) ''The Oxford Dictionary of Statistical Terms'', Oxford University Press. When census data cannot be collected, statisticians collect data by developing specific experiment designs and survey samples. Representative sampling assures that inferences and conclusions can reasonably extend from the sample to the population as a whole. An ex ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Generalized Linear Array Model
In statistics, the generalized linear array model (GLAM) is used for analyzing data sets with array structures. It based on the generalized linear model with the design matrix written as a Kronecker product. Overview The generalized linear array model or GLAM was introduced in 2006. Such models provide a structure and a computational procedure for fitting generalized linear models or GLMs whose model matrix can be written as a Kronecker product and whose data can be written as an array. In a large GLM, the GLAM approach gives very substantial savings in both storage and computational time over the usual GLM algorithm. Suppose that the data \mathbf Y is arranged in a d-dimensional array with size n_1\times n_2\times\dots\times n_d; thus, the corresponding data vector \mathbf y = \operatorname(\mathbf Y) has size n_1n_2n_3\cdots n_d. Suppose also that the design matrix is of the form :\mathbf X = \mathbf X_d\otimes\mathbf X_\otimes\dots\otimes\mathbf X_1. The standard analysis ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Natural Language Processing
Natural language processing (NLP) is an interdisciplinary subfield of linguistics, computer science, and artificial intelligence concerned with the interactions between computers and human language, in particular how to program computers to process and analyze large amounts of natural language data. The goal is a computer capable of "understanding" the contents of documents, including the contextual nuances of the language within them. The technology can then accurately extract information and insights contained in the documents as well as categorize and organize the documents themselves. Challenges in natural language processing frequently involve speech recognition, natural-language understanding, and natural-language generation. History Natural language processing has its roots in the 1950s. Already in 1950, Alan Turing published an article titled " Computing Machinery and Intelligence" which proposed what is now called the Turing test as a criterion of intelligence, ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Tensor Sketch
In statistics, machine learning and algorithms, a tensor sketch is a type of dimensionality reduction that is particularly efficient when applied to vectors that have tensor structure. Such a sketch can be used to speed up explicit kernel methods, bilinear pooling in neural networks and is a cornerstone in many numerical linear algebra algorithms.Woodruff, David P.Sketching as a Tool for Numerical Linear Algebra." Theoretical Computer Science 10.1-2 (2014): 1–157. Mathematical definition Mathematically, a dimensionality reduction or sketching matrix is a matrix M\in\mathbb R^, where k |
Machine Learning
Machine learning (ML) is a field of inquiry devoted to understanding and building methods that 'learn', that is, methods that leverage data to improve performance on some set of tasks. It is seen as a part of artificial intelligence. Machine learning algorithms build a model based on sample data, known as training data, in order to make predictions or decisions without being explicitly programmed to do so. Machine learning algorithms are used in a wide variety of applications, such as in medicine, email filtering, speech recognition, agriculture, and computer vision, where it is difficult or unfeasible to develop conventional algorithms to perform the needed tasks.Hu, J.; Niu, H.; Carrasco, J.; Lennox, B.; Arvin, F.,Voronoi-Based Multi-Robot Autonomous Exploration in Unknown Environments via Deep Reinforcement Learning IEEE Transactions on Vehicular Technology, 2020. A subset of machine learning is closely related to computational statistics, which focuses on making pred ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Artificial Intelligence
Artificial intelligence (AI) is intelligence—perceiving, synthesizing, and inferring information—demonstrated by machines, as opposed to intelligence displayed by animals and humans. Example tasks in which this is done include speech recognition, computer vision, translation between (natural) languages, as well as other mappings of inputs. The ''Oxford English Dictionary'' of Oxford University Press defines artificial intelligence as: the theory and development of computer systems able to perform tasks that normally require human intelligence, such as visual perception, speech recognition, decision-making, and translation between languages. AI applications include advanced web search engines (e.g., Google), recommendation systems (used by YouTube, Amazon and Netflix), understanding human speech (such as Siri and Alexa), self-driving cars (e.g., Tesla), automated decision-making and competing at the highest level in strategic game systems (such as chess and Go). ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Tensor
In mathematics, a tensor is an algebraic object that describes a multilinear relationship between sets of algebraic objects related to a vector space. Tensors may map between different objects such as vectors, scalars, and even other tensors. There are many types of tensors, including scalars and vectors (which are the simplest tensors), dual vectors, multilinear maps between vector spaces, and even some operations such as the dot product. Tensors are defined independent of any basis, although they are often referred to by their components in a basis related to a particular coordinate system. Tensors have become important in physics because they provide a concise mathematical framework for formulating and solving physics problems in areas such as mechanics ( stress, elasticity, fluid mechanics, moment of inertia, ...), electrodynamics ( electromagnetic tensor, Maxwell tensor, permittivity, magnetic susceptibility, ...), general relativity (stress–energy tenso ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Block Matrix
In mathematics, a block matrix or a partitioned matrix is a matrix that is '' interpreted'' as having been broken into sections called blocks or submatrices. Intuitively, a matrix interpreted as a block matrix can be visualized as the original matrix with a collection of horizontal and vertical lines, which break it up, or partition it, into a collection of smaller matrices. Any matrix may be interpreted as a block matrix in one or more ways, with each interpretation defined by how its rows and columns are partitioned. This notion can be made more precise for an n by m matrix M by partitioning n into a collection \text, and then partitioning m into a collection \text. The original matrix is then considered as the "total" of these groups, in the sense that the (i, j) entry of the original matrix corresponds in a 1-to-1 way with some (s, t) offset entry of some (x,y), where x \in \text and y \in \text. Block matrix algebra arises in general from biproducts in categories of mat ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
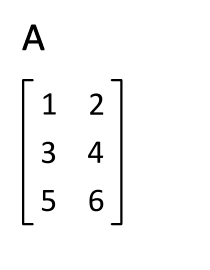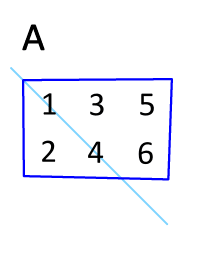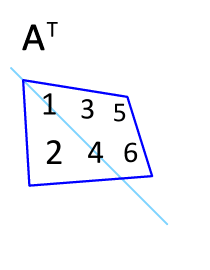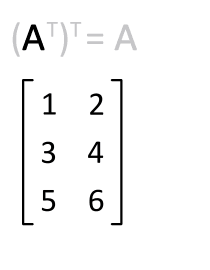
Transposed Block Face-Splitting Product
In linear algebra, the transpose of a matrix is an operator which flips a matrix over its diagonal; that is, it switches the row and column indices of the matrix by producing another matrix, often denoted by (among other notations). The transpose of a matrix was introduced in 1858 by the British mathematician Arthur Cayley. In the case of a logical matrix representing a binary relation R, the transpose corresponds to the converse relation RT. Transpose of a matrix Definition The transpose of a matrix , denoted by , , , A^, , , or , may be constructed by any one of the following methods: # Reflect over its main diagonal (which runs from top-left to bottom-right) to obtain #Write the rows of as the columns of #Write the columns of as the rows of Formally, the -th row, -th column element of is the -th row, -th column element of : :\left mathbf^\operatorname\right = \left mathbf\right. If is an matrix, then is an matrix. In the case of square matrices, ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Johnson–Lindenstrauss Lemma
In mathematics, the Johnson–Lindenstrauss lemma is a result named after William B. Johnson and Joram Lindenstrauss concerning low-distortion embeddings of points from high-dimensional into low-dimensional Euclidean space. The lemma states that a set of points in a high-dimensional space can be embedded into a space of much lower dimension in such a way that distances between the points are nearly preserved. The map used for the embedding is at least Lipschitz, and can even be taken to be an orthogonal projection. The lemma has applications in compressed sensing, manifold learning, dimensionality reduction, and graph embedding. Much of the data stored and manipulated on computers, including text and images, can be represented as points in a high-dimensional space (see vector space model for the case of text). However, the essential algorithms for working with such data tend to become bogged down very quickly as dimension increases. It is therefore desirable to reduce the dimens ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |