|
Jade Mirror Of The Four Unknowns
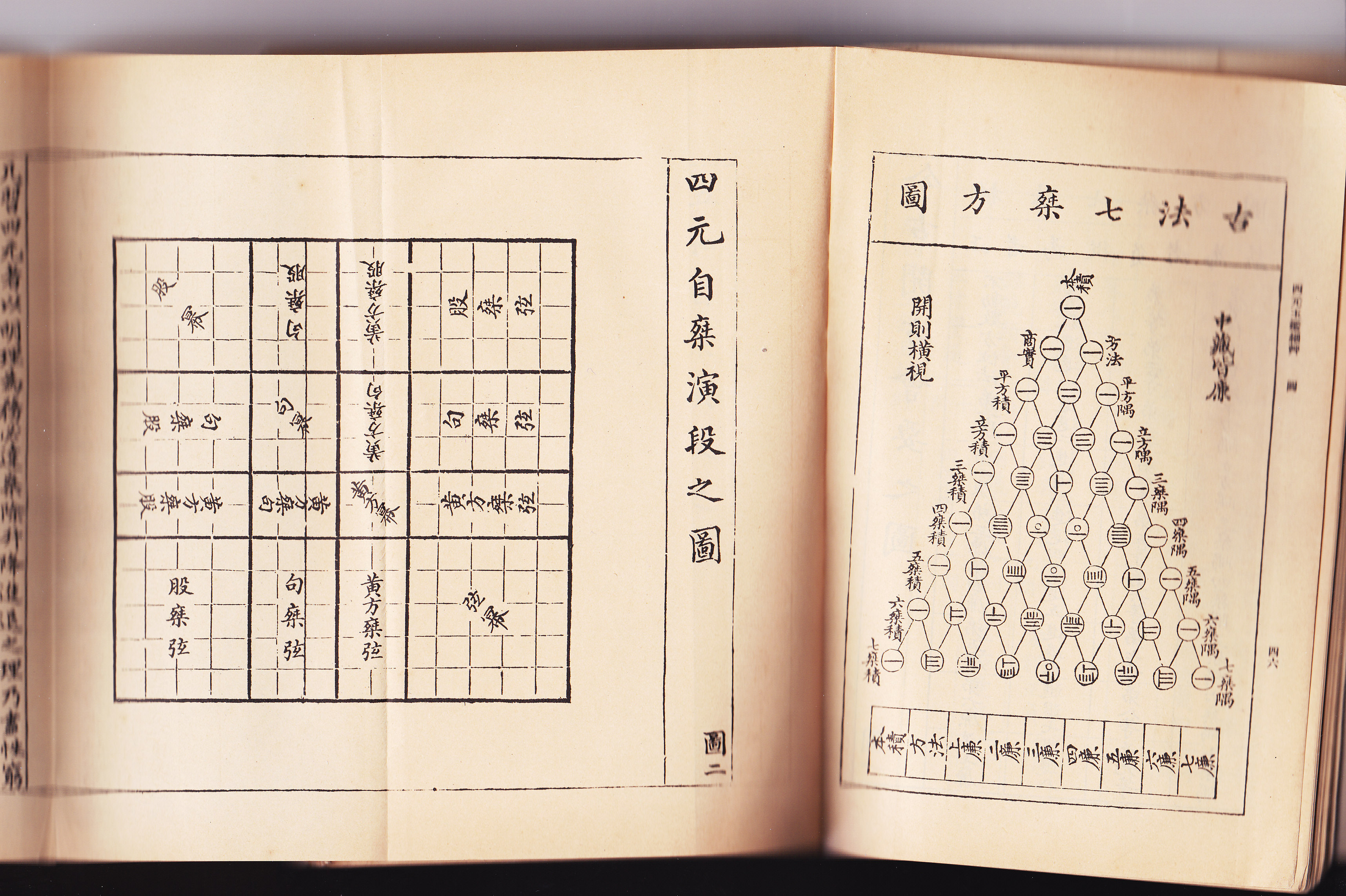
''Jade Mirror of the Four Unknowns'', ''Siyuan yujian'' (), also referred to as ''Jade Mirror of the Four Origins'', is a 1303 mathematical monograph by Yuan dynasty mathematician Zhu Shijie. Zhu advanced Chinese algebra with this Magnum opus. The book consists of an introduction and three books, with a total of 288 problems. The first four problems in the introduction illustrate his method of the four unknowns. He showed how to convert a problem stated verbally into a system of polynomial equations (up to the 14th order), by using up to four unknowns: 天 Heaven, 地 Earth, 人 Man, 物 Matter, and then how to reduce the system to a single polynomial equation in one unknown by successive elimination of unknowns. He then solved the high-order equation by Southern Song dynasty mathematician Qin Jiushao's "Ling long kai fang" method published in Shùshū Jiǔzhāng (“Mathematical Treatise in Nine Sections”) in 1247 (more than 570 years before English mathematician William Ho ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Blaise Pascal
Blaise Pascal ( , , ; ; 19 June 1623 – 19 August 1662) was a French mathematician, physicist, inventor, philosopher, and Catholic Church, Catholic writer. He was a child prodigy who was educated by his father, a tax collector in Rouen. Pascal's earliest mathematical work was on conic sections; he wrote a significant treatise on the subject of projective geometry at the age of 16. He later corresponded with Pierre de Fermat on probability theory, strongly influencing the development of modern economics and social sciences, social science. In 1642, while still a teenager, he started some pioneering work on calculating machines (called Pascal's calculators and later Pascalines), establishing him as one of the first two inventors of the mechanical calculator. Like his contemporary René Descartes, Pascal was also a pioneer in the natural and applied sciences. Pascal wrote in defense of the scientific method and produced several controversial results. He made important contribu ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Chinese Mathematics
Mathematics in China emerged independently by the 11th century BCE. The Chinese independently developed a real number system that includes significantly large and negative numbers, more than one numeral system ( base 2 and base 10), algebra, geometry, number theory and trigonometry. Since the Han Dynasty, as diophantine approximation being a prominent numerical method, the Chinese made substantial progress on polynomial evaluation. Algorithms like regula falsi and expressions like continued fractions are widely used and have been well-documented ever-since. They deliberately find the principal ''n''th root of positive numbers and the roots of equations. The major texts from the period, ''The Nine Chapters on the Mathematical Art'' and the ''Book on Numbers and Computation'' gave detailed processes for solving various mathematical problems in daily life. All procedures were computed using a counting board in both texts, and they included inverse elements as well as Euclidean divi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Yenching University
Yenching University (), was a university in Beijing, China, that was formed out of the merger of four Christian colleges between the years 1915 and 1920. The term "Yenching" comes from an alternative name for old Beijing, derived from its status as capital of the state of Yan, one of the seven Warring States that existed until the 3rd century BC. History Yenching University was formed through the merger of four Christian schools over the course of five years, from 1915 to 1920: * Hweiwen University (), also known as the Methodist Peking University, founded in 1890 by the Methodist Episcopal Church. This should not be confused with the National Peking University founded eight years later in 1898. Huiwen's precursor (崇內懷理書院) was founded in 1870. Hiram Harrison Lowry was its principal. * North China Union College in Tongzhou (). Its precursor (公理會潞河書院) was founded by the Congregational Church. Devello Z. Sheffield was the school's principal. *North Chin ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Haidao Suanjing
''Haidao Suanjing'' (; ''The Sea Island Mathematical Manual'') was written by the Chinese mathematician Liu Hui of the Three Kingdoms era (220–280) as an extension of chapter 9 of ''The Nine Chapters on the Mathematical Art''. L. van. Hee, ''Le Classique d I'Ile Maritime: Ouvrage Chinois de III'' siecle 1932 During the Tang Dynasty, this appendix was taken out from ''The Nine Chapters on the Mathematical Art'' as a separate book, titled ''Haidao suanjing'' (''Sea Island Mathematical Manual''), named after problem No 1 "Looking at a sea island." In the time of the early Tang dynasty, ''Haidao Suanjing'' was selected into one of The Ten Computational Canons as the official mathematical texts for imperial examinations in mathematics. Content This book contained many practical problems of surveying using geometry. This work provided detailed instructions on how to measure distances and heights with tall surveyor's poles and horizontal bars fixed at right angles to them. The ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Tian Yuan Shu
''Tian yuan shu'' () is a Chinese system of algebra for polynomial equations. Some of the earliest existing writings were created in the 13th century during the Yuan dynasty. However, the tianyuanshu method was known much earlier, in the Song dynasty and possibly before. History The Tianyuanshu was explained in the writings of Zhu Shijie (''Jade Mirror of the Four Unknowns'') and Li Zhi (''Ceyuan haijing''), two Chinese mathematicians during the Mongol Yuan dynasty. However, after the Ming overthrew the Mongol Yuan, Zhu and Li's mathematical works went into disuse as the Ming literati became suspicious of knowledge imported from Mongol Yuan times. Only recently, with the advent of modern mathematics in China has the tianyuanshu been re-deciphered. Meanwhile, ''tian yuan shu'' arrived in Japan, where it is called ''tengen-jutsu''. Zhu's text '' Suanxue qimeng'' was deciphered and was important in the development of Japanese mathematics (''wasan'') in the 17th and 18th centuri ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Diagonal Form
In mathematics, a diagonal form is an algebraic form (homogeneous polynomial) without cross-terms involving different indeterminates. That is, it is :\sum_^n a_i ^m\ for some given degree ''m''. Such forms ''F'', and the hypersurfaces ''F'' = 0 they define in projective space, are very special in geometric terms, with many symmetries. They also include famous cases like the Fermat curves, and other examples well known in the theory of Diophantine equations. A great deal has been worked out about their theory: algebraic geometry, local zeta-functions via Jacobi sums, Hardy-Littlewood circle method. Examples :X^2+Y^2-Z^2 = 0 is the unit circle in ''P''2 :X^2-Y^2-Z^2 = 0 is the unit hyperbola in ''P''2. :x_0^3+x_1^3+x_2^3+x_3^3=0 gives the Fermat cubic surface in ''P''3 with 27 lines. The 27 lines in this example are easy to describe explicitly: they are the 9 lines of the form (''x'' : ''ax'' : ''y'' : ''by'') where ''a'' and ''b'' are fixed numbers with cube −1, an ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
.png)



