|
Itô's Lemma
In mathematics, Itô's lemma or Itô's formula (also called the Itô-Doeblin formula, especially in French literature) is an identity used in Itô calculus to find the differential of a time-dependent function of a stochastic process. It serves as the stochastic calculus counterpart of the chain rule. It can be heuristically derived by forming the Taylor series expansion of the function up to its second derivatives and retaining terms up to first order in the time increment and second order in the Wiener process increment. The lemma is widely employed in mathematical finance, and its best known application is in the derivation of the Black–Scholes equation for option values. Motivation Suppose we are given the stochastic differential equation dX_t = \mu_t\ dt + \sigma_t\ dB_t, where is a Wiener process and the functions \mu_t, \sigma_t are deterministic (not stochastic) functions of time. In general, it's not possible to write a solution X_t directly in terms of B_t. Howeve ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Group Theory
In abstract algebra, group theory studies the algebraic structures known as group (mathematics), groups. The concept of a group is central to abstract algebra: other well-known algebraic structures, such as ring (mathematics), rings, field (mathematics), fields, and vector spaces, can all be seen as groups endowed with additional operation (mathematics), operations and axioms. Groups recur throughout mathematics, and the methods of group theory have influenced many parts of algebra. Linear algebraic groups and Lie groups are two branches of group theory that have experienced advances and have become subject areas in their own right. Various physical systems, such as crystals and the hydrogen atom, and Standard Model, three of the four known fundamental forces in the universe, may be modelled by symmetry groups. Thus group theory and the closely related representation theory have many important applications in physics, chemistry, and materials science. Group theory is also ce ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Gradient
In vector calculus, the gradient of a scalar-valued differentiable function of several variables is the vector field (or vector-valued function) \nabla f whose value at a point p is the "direction and rate of fastest increase". If the gradient of a function is non-zero at a point , the direction of the gradient is the direction in which the function increases most quickly from , and the magnitude of the gradient is the rate of increase in that direction, the greatest absolute directional derivative. Further, a point where the gradient is the zero vector is known as a stationary point. The gradient thus plays a fundamental role in optimization theory, where it is used to maximize a function by gradient ascent. In coordinate-free terms, the gradient of a function f(\bf) may be defined by: :df=\nabla f \cdot d\bf where ''df'' is the total infinitesimal change in ''f'' for an infinitesimal displacement d\bf, and is seen to be maximal when d\bf is in the direction of the gradi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Annualized Return
In finance, return is a profit on an investment. It comprises any change in value of the investment, and/or cash flows (or securities, or other investments) which the investor receives from that investment, such as interest payments, coupons, cash dividends, stock dividends or the payoff from a derivative or structured product. It may be measured either in absolute terms (e.g., dollars) or as a percentage of the amount invested. The latter is also called the holding period return. A loss instead of a profit is described as a '' negative return'', assuming the amount invested is greater than zero. To compare returns over time periods of different lengths on an equal basis, it is useful to convert each return into a return over a period of time of a standard length. The result of the conversion is called the rate of return. Typically, the period of time is a year, in which case the rate of return is also called the annualized return, and the conversion process, described bel ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Convexity Correction
In mathematical finance, convexity refers to non-linearities in a financial model. In other words, if the price of an underlying variable changes, the price of an output does not change linearly, but depends on the second derivative (or, loosely speaking, higher-order terms) of the modeling function. Geometrically, the model is no longer flat but curved, and the degree of curvature is called the convexity. Terminology Strictly speaking, convexity refers to the second derivative of output price with respect to an input price. In derivative pricing, this is referred to as Gamma (Γ), one of the Greeks. In practice the most significant of these is bond convexity, the second derivative of bond price with respect to interest rates. As the second derivative is the first non-linear term, and thus often the most significant, "convexity" is also used loosely to refer to non-linearities generally, including higher-order terms. Refining a model to account for non-linearities is referred ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
AM–GM Inequality
In mathematics, the inequality of arithmetic and geometric means, or more briefly the AM–GM inequality, states that the arithmetic mean of a list of non-negative real numbers is greater than or equal to the geometric mean of the same list; and further, that the two means are equal if and only if every number in the list is the same (in which case they are both that number). The simplest non-trivial case – i.e., with more than one variable – for two non-negative numbers and , is the statement that :\frac2 \ge \sqrt with equality if and only if . This case can be seen from the fact that the square of a real number is always non-negative (greater than or equal to zero) and from the elementary case of the binomial formula: :\begin 0 & \le (x-y)^2 \\ & = x^2-2xy+y^2 \\ & = x^2+2xy+y^2 - 4xy \\ & = (x+y)^2 - 4xy. \end Hence , with equality precisely when , i.e. . The AM–GM inequality then follows from taking the positive square root of both sides and then dividing both ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Log-normal Distribution
In probability theory, a log-normal (or lognormal) distribution is a continuous probability distribution of a random variable whose logarithm is normally distributed. Thus, if the random variable is log-normally distributed, then has a normal distribution. Equivalently, if has a normal distribution, then the exponential function of , , has a log-normal distribution. A random variable which is log-normally distributed takes only positive real values. It is a convenient and useful model for measurements in exact and engineering sciences, as well as medicine, economics and other topics (e.g., energies, concentrations, lengths, prices of financial instruments, and other metrics). The distribution is occasionally referred to as the Galton distribution or Galton's distribution, after Francis Galton. The log-normal distribution has also been associated with other names, such as McAlister, Gibrat and Cobb–Douglas. A log-normal process is the statistical realization of the multipl ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Geometric Brownian Motion
A geometric Brownian motion (GBM) (also known as exponential Brownian motion) is a continuous-time stochastic process in which the logarithm of the randomly varying quantity follows a Brownian motion (also called a Wiener process) with drift. It is an important example of stochastic processes satisfying a stochastic differential equation (SDE); in particular, it is used in mathematical finance to model stock prices in the Black–Scholes model. Technical definition: the SDE A stochastic process ''S''''t'' is said to follow a GBM if it satisfies the following stochastic differential equation (SDE): : dS_t = \mu S_t\,dt + \sigma S_t\,dW_t where W_t is a Wiener process or Brownian motion, and \mu ('the percentage drift') and \sigma ('the percentage volatility') are constants. The former is used to model deterministic trends, while the latter term is often used to model a set of unpredictable events occurring during this motion. Solving the SDE For an arbitrary initial ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Càdlàg
In mathematics, a càdlàg (French: "''continue à droite, limite à gauche''"), RCLL ("right continuous with left limits"), or corlol ("continuous on (the) right, limit on (the) left") function is a function defined on the real numbers (or a subset of them) that is everywhere right-continuous and has left limits everywhere. Càdlàg functions are important in the study of stochastic processes that admit (or even require) jumps, unlike Brownian motion, which has continuous sample paths. The collection of càdlàg functions on a given domain is known as Skorokhod space. Two related terms are càglàd, standing for "continue à gauche, limite à droite", the left-right reversal of càdlàg, and càllàl for "continue à l'un, limite à l’autre" (continuous on one side, limit on the other side), for a function which at each point of the domain is either càdlàg or càglàd. Definition Let be a metric space, and let . A function is called a càdlàg function if, for every , * the ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Semimartingale
In probability theory, a real valued stochastic process ''X'' is called a semimartingale if it can be decomposed as the sum of a local martingale and a càdlàg adapted finite-variation process. Semimartingales are "good integrators", forming the largest class of processes with respect to which the Itô integral and the Stratonovich integral can be defined. The class of semimartingales is quite large (including, for example, all continuously differentiable processes, Brownian motion and Poisson processes). Submartingales and supermartingales together represent a subset of the semimartingales. Definition A real valued process ''X'' defined on the filtered probability space (Ω,''F'',(''F''''t'')''t'' ≥ 0,P) is called a semimartingale if it can be decomposed as :X_t = M_t + A_t where ''M'' is a local martingale and ''A'' is a càdlàg adapted process of locally bounded variation. An R''n''-valued process ''X'' = (''X''1,…,''X''''n'') is a semimartingale i ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Martingale (probability Theory)
In probability theory, a martingale is a sequence of random variables (i.e., a stochastic process) for which, at a particular time, the conditional expectation of the next value in the sequence is equal to the present value, regardless of all prior values. History Originally, '' martingale'' referred to a class of betting strategies that was popular in 18th-century France. The simplest of these strategies was designed for a game in which the gambler wins their stake if a coin comes up heads and loses it if the coin comes up tails. The strategy had the gambler double their bet after every loss so that the first win would recover all previous losses plus win a profit equal to the original stake. As the gambler's wealth and available time jointly approach infinity, their probability of eventually flipping heads approaches 1, which makes the martingale betting strategy seem like a sure thing. However, the exponential growth of the bets eventually bankrupts its users due to f ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
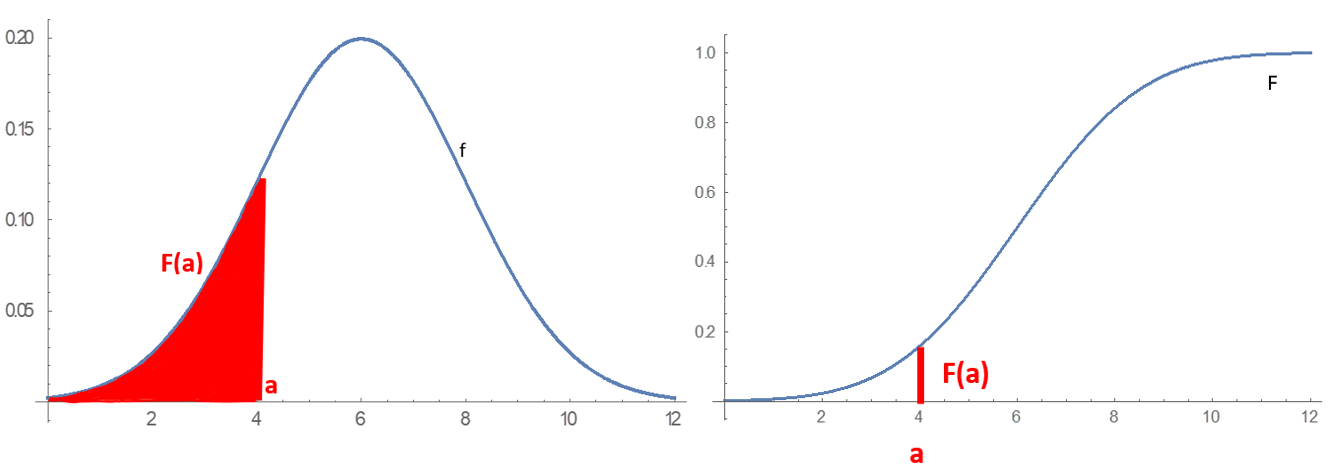
Probability Distribution
In probability theory and statistics, a probability distribution is the mathematical function that gives the probabilities of occurrence of different possible outcomes for an experiment. It is a mathematical description of a random phenomenon in terms of its sample space and the probabilities of events (subsets of the sample space). For instance, if is used to denote the outcome of a coin toss ("the experiment"), then the probability distribution of would take the value 0.5 (1 in 2 or 1/2) for , and 0.5 for (assuming that the coin is fair). Examples of random phenomena include the weather conditions at some future date, the height of a randomly selected person, the fraction of male students in a school, the results of a survey to be conducted, etc. Introduction A probability distribution is a mathematical description of the probabilities of events, subsets of the sample space. The sample space, often denoted by \Omega, is the set of all possible outcomes of a random phe ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
.svg "Click for more on -> Probability Distribution")