|
Hopkins Statistic
The Hopkins statistic (introduced by Brian Hopkins and John Gordon Skellam) is a way of measuring the cluster tendency of a data set. It belongs to the family of sparse sampling tests. It acts as a statistical hypothesis test where the null hypothesis is that the data is generated by a Poisson point process In probability theory, statistics and related fields, a Poisson point process (also known as: Poisson random measure, Poisson random point field and Poisson point field) is a type of mathematical object that consists of points randomly located ... and are thus uniformly randomly distributed. If individuals are aggregated, then its value approaches 1, and if they are randomly distributed along the value tends to 0.5. Preliminaries A typical formulation of the Hopkins statistic follows. :Let X be the set of n data points. :Generate a random sample \overset of m \ll n data points sampled without replacement from X. :Generate a set Y of m uniformly randomly distributed data p ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
John Gordon Skellam
John Gordon Skellam (1914-1979) was a statistician and ecologist, who discovered the Skellam distribution. Skellam was born in Staffordshire. He was educated at Hanley High School where he won several scholarships including free admission to New College in Oxford. He was one of the most respected members of the British Region of the Biometric Society. In 1951, John G. Skellam developed the reaction-diffusion model of invasion biology. This model describes the dynamics of populations, which simultaneously develops and spreads, and provides that the invasion front moves with constant speed. He explained on the basis of habitation muskrat introduced to Europe that only by chance the species may be in a place where it would have to grow. Skellam has provided a model that allows to take the dynamics of populations as a random variable A random variable (also called random quantity, aleatory variable, or stochastic variable) is a Mathematics, mathematical formalization of a quan ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Cluster Tendency
Cluster analysis or clustering is the data analyzing technique in which task of grouping a set of objects in such a way that objects in the same group (called a cluster) are more similar (in some specific sense defined by the analyst) to each other than to those in other groups (clusters). It is a main task of exploratory data analysis, and a common technique for statistical data analysis, used in many fields, including pattern recognition, image analysis, information retrieval, bioinformatics, data compression, computer graphics and machine learning. Cluster analysis refers to a family of algorithms and tasks rather than one specific algorithm. It can be achieved by various algorithms that differ significantly in their understanding of what constitutes a cluster and how to efficiently find them. Popular notions of clusters include groups with small distances between cluster members, dense areas of the data space, intervals or particular statistical distributions. Clustering can ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
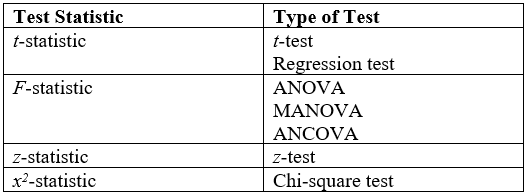
Statistical Hypothesis Testing
A statistical hypothesis test is a method of statistical inference used to decide whether the data provide sufficient evidence to reject a particular hypothesis. A statistical hypothesis test typically involves a calculation of a test statistic. Then a decision is made, either by comparing the test statistic to a Critical value (statistics), critical value or equivalently by evaluating a p-value, ''p''-value computed from the test statistic. Roughly 100 list of statistical tests, specialized statistical tests are in use and noteworthy. History While hypothesis testing was popularized early in the 20th century, early forms were used in the 1700s. The first use is credited to John Arbuthnot (1710), followed by Pierre-Simon Laplace (1770s), in analyzing the human sex ratio at birth; see . Choice of null hypothesis Paul Meehl has argued that the epistemological importance of the choice of null hypothesis has gone largely unacknowledged. When the null hypothesis is predicted by the ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Null Hypothesis
The null hypothesis (often denoted ''H''0) is the claim in scientific research that the effect being studied does not exist. The null hypothesis can also be described as the hypothesis in which no relationship exists between two sets of data or variables being analyzed. If the null hypothesis is true, any experimentally observed effect is due to chance alone, hence the term "null". In contrast with the null hypothesis, an alternative hypothesis (often denoted ''H''A or ''H''1) is developed, which claims that a relationship does exist between two variables. Basic definitions The null hypothesis and the ''alternative hypothesis'' are types of conjectures used in statistical tests to make statistical inferences, which are formal methods of reaching conclusions and separating scientific claims from statistical noise. The statement being tested in a test of statistical significance is called the null hypothesis. The test of significance is designed to assess the strength of the e ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Poisson Point Process
In probability theory, statistics and related fields, a Poisson point process (also known as: Poisson random measure, Poisson random point field and Poisson point field) is a type of mathematical object that consists of points randomly located on a mathematical space with the essential feature that the points occur independently of one another. The process's name derives from the fact that the number of points in any given finite region follows a Poisson distribution. The process and the distribution are named after French mathematician Siméon Denis Poisson. The process itself was discovered independently and repeatedly in several settings, including experiments on radioactive decay, telephone call arrivals and actuarial science. This point process is used as a mathematical model for seemingly random processes in numerous disciplines including astronomy,G. J. Babu and E. D. Feigelson. Spatial point processes in astronomy. ''Journal of statistical planning and inference'', 50( ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |