|
Hotelling's T-square Distribution
In statistics, particularly in hypothesis testing, the Hotelling's ''T''-squared distribution (''T''2), proposed by Harold Hotelling, is a multivariate probability distribution that is tightly related to the ''F''-distribution and is most notable for arising as the distribution of a set of sample statistics that are natural generalizations of the statistics underlying the Student's ''t''-distribution. The Hotelling's ''t''-squared statistic (''t''2) is a generalization of Student's ''t''-statistic that is used in multivariate hypothesis testing. Motivation The distribution arises in multivariate statistics in undertaking tests of the differences between the (multivariate) means of different populations, where tests for univariate problems would make use of a ''t''-test. The distribution is named for Harold Hotelling, who developed it as a generalization of Student's ''t''-distribution. Definition If the vector d is Gaussian multivariate-distributed with zero mean and un ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Degrees Of Freedom (statistics)
In statistics, the number of degrees of freedom is the number of values in the final calculation of a statistic that are free to vary. Estimates of statistical parameters can be based upon different amounts of information or data. The number of independent pieces of information that go into the estimate of a parameter is called the degrees of freedom. In general, the degrees of freedom of an estimate of a parameter are equal to the number of independent scores that go into the estimate minus the number of parameters used as intermediate steps in the estimation of the parameter itself. For example, if the variance is to be estimated from a random sample of ''N'' independent scores, then the degrees of freedom is equal to the number of independent scores (''N'') minus the number of parameters estimated as intermediate steps (one, namely, the sample mean) and is therefore equal to ''N'' − 1. Mathematically, degrees of freedom is the number of dimensions of the domain ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Random Variable
A random variable (also called random quantity, aleatory variable, or stochastic variable) is a mathematical formalization of a quantity or object which depends on random events. It is a mapping or a function from possible outcomes (e.g., the possible upper sides of a flipped coin such as heads H and tails T) in a sample space (e.g., the set \) to a measurable space, often the real numbers (e.g., \ in which 1 corresponding to H and -1 corresponding to T). Informally, randomness typically represents some fundamental element of chance, such as in the roll of a dice; it may also represent uncertainty, such as measurement error. However, the interpretation of probability is philosophically complicated, and even in specific cases is not always straightforward. The purely mathematical analysis of random variables is independent of such interpretational difficulties, and can be based upon a rigorous axiomatic setup. In the formal mathematical language of measure theory, a rando ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Location Parameter
In geography, location or place are used to denote a region (point, line, or area) on Earth's surface or elsewhere. The term ''location'' generally implies a higher degree of certainty than ''place'', the latter often indicating an entity with an ambiguous boundary, relying more on human or social attributes of place identity and sense of place than on geometry. Types Locality A locality, settlement, or populated place is likely to have a well-defined name but a boundary that is not well defined varies by context. London, for instance, has a legal boundary, but this is unlikely to completely match with general usage. An area within a town, such as Covent Garden in London, also almost always has some ambiguity as to its extent. In geography, location is considered to be more precise than "place". Relative location A relative location, or situation, is described as a displacement from another site. An example is "3 miles northwest of Seattle". Absolute location An absolute lo ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Multivariate Normal Distribution
In probability theory and statistics, the multivariate normal distribution, multivariate Gaussian distribution, or joint normal distribution is a generalization of the one-dimensional ( univariate) normal distribution to higher dimensions. One definition is that a random vector is said to be ''k''-variate normally distributed if every linear combination of its ''k'' components has a univariate normal distribution. Its importance derives mainly from the multivariate central limit theorem. The multivariate normal distribution is often used to describe, at least approximately, any set of (possibly) correlated real-valued random variables each of which clusters around a mean value. Definitions Notation and parameterization The multivariate normal distribution of a ''k''-dimensional random vector \mathbf = (X_1,\ldots,X_k)^ can be written in the following notation: : \mathbf\ \sim\ \mathcal(\boldsymbol\mu,\, \boldsymbol\Sigma), or to make it explicitly known that ''X ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Confidence Region
In statistics, a confidence region is a multi-dimensional generalization of a confidence interval. It is a set of points in an ''n''-dimensional space, often represented as an ellipsoid around a point which is an estimated solution to a problem, although other shapes can occur. Interpretation The confidence region is calculated in such a way that if a set of measurements were repeated many times and a confidence region calculated in the same way on each set of measurements, then a certain percentage of the time (e.g. 95%) the confidence region would include the point representing the "true" values of the set of variables being estimated. However, unless certain assumptions about prior probabilities are made, it does not mean, when one confidence region has been calculated, that there is a 95% probability that the "true" values lie inside the region, since we do not assume any particular probability distribution of the "true" values and we may or may not have other information ab ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
P-value
In null-hypothesis significance testing, the ''p''-value is the probability of obtaining test results at least as extreme as the result actually observed, under the assumption that the null hypothesis is correct. A very small ''p''-value means that such an extreme observed outcome would be very unlikely under the null hypothesis. Reporting ''p''-values of statistical tests is common practice in academic publications of many quantitative fields. Since the precise meaning of ''p''-value is hard to grasp, misuse is widespread and has been a major topic in metascience. Basic concepts In statistics, every conjecture concerning the unknown probability distribution of a collection of random variables representing the observed data X in some study is called a ''statistical hypothesis''. If we state one hypothesis only and the aim of the statistical test is to see whether this hypothesis is tenable, but not to investigate other specific hypotheses, then such a test is called a null ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Distribution
Distribution may refer to: Mathematics *Distribution (mathematics), generalized functions used to formulate solutions of partial differential equations *Probability distribution, the probability of a particular value or value range of a variable **Cumulative distribution function, in which the probability of being no greater than a particular value is a function of that value *Frequency distribution, a list of the values recorded in a sample *Inner distribution, and outer distribution, in coding theory *Distribution (differential geometry), a subset of the tangent bundle of a manifold *Distributed parameter system, systems that have an infinite-dimensional state-space *Distribution of terms, a situation in which all members of a category are accounted for *Distributivity, a property of binary operations that generalises the distributive law from elementary algebra *Distribution (number theory) *Distribution problems, a common type of problems in combinatorics where the goal is ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Mahalanobis Distance
The Mahalanobis distance is a measure of the distance between a point ''P'' and a distribution ''D'', introduced by P. C. Mahalanobis in 1936. Mahalanobis's definition was prompted by the problem of identifying the similarities of skulls based on measurements in 1927. It is a multi-dimensional generalization of the idea of measuring how many standard deviations away ''P'' is from the mean of ''D''. This distance is zero for ''P'' at the mean of ''D'' and grows as ''P'' moves away from the mean along each principal component axis. If each of these axes is re-scaled to have unit variance, then the Mahalanobis distance corresponds to standard Euclidean distance in the transformed space. The Mahalanobis distance is thus unitless, scale-invariant, and takes into account the correlations of the data set. Definition Given a probability distribution Q on \R^N, with mean \vec = (\mu_1, \mu_2, \mu_3, \dots , \mu_N)^\mathsf and positive-definite covariance matrix S, the Mahalanobis ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Positive-definite Matrix
In mathematics, a symmetric matrix M with real entries is positive-definite if the real number z^\textsfMz is positive for every nonzero real column vector z, where z^\textsf is the transpose of More generally, a Hermitian matrix (that is, a complex matrix equal to its conjugate transpose) is positive-definite if the real number z^* Mz is positive for every nonzero complex column vector z, where z^* denotes the conjugate transpose of z. Positive semi-definite matrices are defined similarly, except that the scalars z^\textsfMz and z^* Mz are required to be positive ''or zero'' (that is, nonnegative). Negative-definite and negative semi-definite matrices are defined analogously. A matrix that is not positive semi-definite and not negative semi-definite is sometimes called indefinite. A matrix is thus positive-definite if and only if it is the matrix of a positive-definite quadratic form or Hermitian form. In other words, a matrix is positive-definite if and only if it d ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Apostrophe
The apostrophe ( or ) is a punctuation mark, and sometimes a diacritical mark, in languages that use the Latin alphabet and some other alphabets. In English, the apostrophe is used for two basic purposes: * The marking of the omission of one or more letters, e.g. the contraction of "do not" to "don't". * The marking of possessive case of nouns (as in "the eagle's feathers", "in one month's time", "at your parents' ome). The word "apostrophe" comes ultimately from Greek (, 'he accent ofturning away or elision'), through Latin and French. For use in computer systems, Unicode has code points for three different forms of apostrophe. Usage in English Historical development The apostrophe was first used by Pietro Bembo in his edition of '' De Aetna'' (1496). It was introduced into English in the 16th century in imitation of French practice. French practice Introduced by Geoffroy Tory (1529), the apostrophe was used in place of a vowel letter to indicate elision ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
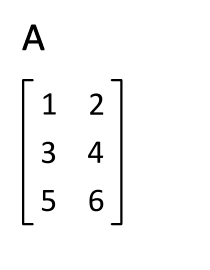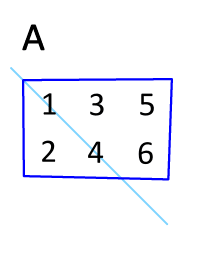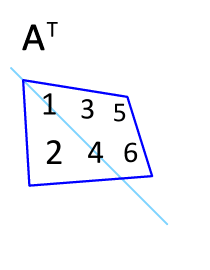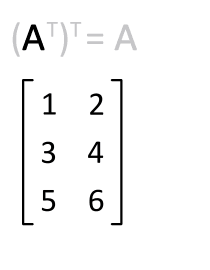
Transpose
In linear algebra, the transpose of a matrix is an operator which flips a matrix over its diagonal; that is, it switches the row and column indices of the matrix by producing another matrix, often denoted by (among other notations). The transpose of a matrix was introduced in 1858 by the British mathematician Arthur Cayley. In the case of a logical matrix representing a binary relation R, the transpose corresponds to the converse relation RT. Transpose of a matrix Definition The transpose of a matrix , denoted by , , , A^, , , or , may be constructed by any one of the following methods: # Reflect over its main diagonal (which runs from top-left to bottom-right) to obtain #Write the rows of as the columns of #Write the columns of as the rows of Formally, the -th row, -th column element of is the -th row, -th column element of : :\left mathbf^\operatorname\right = \left mathbf\right. If is an matrix, then is an matrix. In the case of square matric ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |