|
Holditch's Theorem
In plane geometry, Holditch's theorem states that if a chord of fixed length is allowed to rotate inside a convex closed curve, then the locus of a point on the chord a distance ''p'' from one end and a distance ''q'' from the other is a closed curve whose enclosed area is less than that of the original curve by \pi pq. The theorem was published in 1858 by Rev. Hamnet Holditch. While not mentioned by Holditch, the proof of the theorem requires an assumption that the chord be short enough that the traced locus is a simple closed curve. Observations The theorem is included as one of Clifford Pickover's 250 milestones in the history of mathematics. Some peculiarities of the theorem include that the area formula \pi pq is independent of both the shape and the size of the original curve, and that the area formula is the same as for that of the area of an ellipse with semi-axes ''p'' and ''q''. The theorem's author was a president of Caius College, Cambridge. Extensions Broman give ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Holditch S Theorem in plane geometry, named after Hamnet Holditch
*
{{Disambig, geo, surname ...
Holditch may refer to: * Holditch, a hamlet in Thorncombe, Dorset (formerly Devon), England * Holditch (ward), in the Borough of Newcastle-under-Lyme, England * Hamnet Holditch (1800-1867), English mathematician *Philip Holditch (died ), English merchant and politician, MP for Totnes in 1601 See also * Holditch Colliery disaster, of 1937 in Staffordshire, England *Holditch's theorem In plane geometry, Holditch's theorem states that if a chord of fixed length is allowed to rotate inside a convex closed curve, then the locus of a point on the chord a distance ''p'' from one end and a distance ''q'' from the other is a closed ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
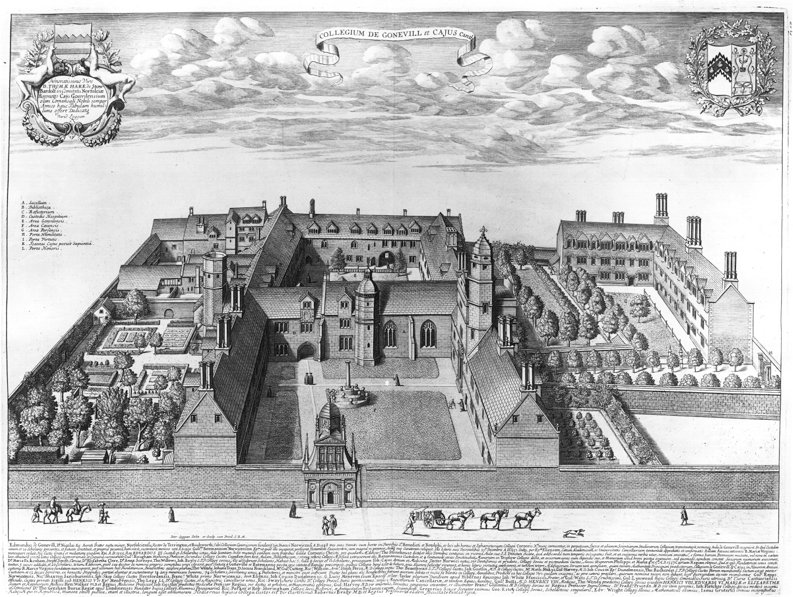
Caius College, Cambridge
Gonville and Caius College, often referred to simply as Caius ( ), is a constituent college of the University of Cambridge in Cambridge, England. Founded in 1348, it is the fourth-oldest of the University of Cambridge's 31 colleges and one of the wealthiest. The college has been attended by many students who have gone on to significant accomplishment, including fifteen Nobel Prize winners, the second-highest of any Oxbridge college after Trinity College, Cambridge. The college has long historical associations with the teaching of medicine, especially due to its prominent alumni in the medical profession. It also has globally-recognized and prestigious academic programmes in law, economics, English literature, and history. Famous Gonville and Caius alumni include physicians John Caius (who gave the college the caduceus in its insignia) and William Harvey. Other alumni in the sciences include Francis Crick (joint discoverer of the structure of DNA with James Watson), James Chad ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
The Lady's And Gentleman's Diary
''The Lady's and Gentleman's Diary'' was a recreational mathematics magazine formed as a successor of ''The Ladies' Diary'' and ''Gentleman's Diary'' in 1841. It was published annually between 1841 and 1871 by the Company of Stationers; its editor from 1844 to 1865 was Wesley S. B. Woolhouse. It consisted mostly of problems posed by its readers, with their solutions given in later volumes, though it also contained word puzzles and poetry. The magazine was based in London. It ceased publication in 1871. This should not be confused with ''Ladies and Gentlemens Diary, or Royal Almanack'' (1775 to 1786) which was printed by Thomas Carnan and edited by Reuben Burrow and was a short lived competitor to ''The Ladies' Diary''. Kirkman's schoolgirl problem Kirkman's schoolgirl problem is a problem in combinatorics proposed by Rev. Thomas Penyngton Kirkman in 1850 as Query VI in '' The Lady's and Gentleman's Diary'' (pg.48). The problem states: Fifteen young ladies in a school walk o ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Convex Polygon
In geometry, a convex polygon is a polygon that is the boundary of a convex set. This means that the line segment between two points of the polygon is contained in the union of the interior and the boundary of the polygon. In particular, it is a simple polygon (not self-intersecting). Equivalently, a polygon is convex if every line that does not contain any edge intersects the polygon in at most two points. A strictly convex polygon is a convex polygon such that no line contains two of its edges. In a convex polygon, all interior angles are less than or equal to 180 degrees, while in a strictly convex polygon all interior angles are strictly less than 180 degrees. Properties The following properties of a simple polygon are all equivalent to convexity: *Every internal angle is strictly less than 180 degrees. *Every point on every line segment between two points inside or on the boundary of the polygon remains inside or on the boundary. *The polygon is entirely contained in ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Altitude (triangle)
In geometry, an altitude of a triangle is a line segment through a vertex and perpendicular to (i.e., forming a right angle with) a line containing the base (the side opposite the vertex). This line containing the opposite side is called the ''extended base'' of the altitude. The intersection of the extended base and the altitude is called the ''foot'' of the altitude. The length of the altitude, often simply called "the altitude", is the distance between the extended base and the vertex. The process of drawing the altitude from the vertex to the foot is known as ''dropping the altitude'' at that vertex. It is a special case of orthogonal projection. Altitudes can be used in the computation of the area of a triangle: one half of the product of an altitude's length and its base's length equals the triangle's area. Thus, the longest altitude is perpendicular to the shortest side of the triangle. The altitudes are also related to the sides of the triangle through the trigonometri ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Acute Angle
In Euclidean geometry, an angle is the figure formed by two rays, called the '' sides'' of the angle, sharing a common endpoint, called the ''vertex'' of the angle. Angles formed by two rays lie in the plane that contains the rays. Angles are also formed by the intersection of two planes. These are called dihedral angles. Two intersecting curves may also define an angle, which is the angle of the rays lying tangent to the respective curves at their point of intersection. ''Angle'' is also used to designate the measure of an angle or of a rotation. This measure is the ratio of the length of a circular arc to its radius. In the case of a geometric angle, the arc is centered at the vertex and delimited by the sides. In the case of a rotation, the arc is centered at the center of the rotation and delimited by any other point and its image by the rotation. History and etymology The word ''angle'' comes from the Latin word ''angulus'', meaning "corner"; cognate words are the Greek ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Retrograde Motion
Retrograde motion in astronomy is, in general, orbital or rotational motion of an object in the direction opposite the rotation of its primary, that is, the central object (right figure). It may also describe other motions such as precession or nutation of an object's rotational axis. Prograde or direct motion is more normal motion in the same direction as the primary rotates. However, "retrograde" and "prograde" can also refer to an object other than the primary if so described. The direction of rotation is determined by an inertial frame of reference, such as distant fixed stars. In the Solar System, the orbits around the Sun of all planets and most other objects, except many comets, are prograde. They orbit around the Sun in the same direction as the sun rotates about its axis, which is counterclockwise when observed from above the Sun's north pole. Except for Venus and Uranus, planetary rotations around their axes are also prograde. Most natural satellites have prograde or ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Triangle
A triangle is a polygon with three Edge (geometry), edges and three Vertex (geometry), vertices. It is one of the basic shapes in geometry. A triangle with vertices ''A'', ''B'', and ''C'' is denoted \triangle ABC. In Euclidean geometry, any three points, when non-Collinearity, collinear, determine a unique triangle and simultaneously, a unique Plane (mathematics), plane (i.e. a two-dimensional Euclidean space). In other words, there is only one plane that contains that triangle, and every triangle is contained in some plane. If the entire geometry is only the Euclidean plane, there is only one plane and all triangles are contained in it; however, in higher-dimensional Euclidean spaces, this is no longer true. This article is about triangles in Euclidean geometry, and in particular, the Euclidean plane, except where otherwise noted. Types of triangle The terminology for categorizing triangles is more than two thousand years old, having been defined on the very first page of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Ellipse
In mathematics, an ellipse is a plane curve surrounding two focus (geometry), focal points, such that for all points on the curve, the sum of the two distances to the focal points is a constant. It generalizes a circle, which is the special type of ellipse in which the two focal points are the same. The elongation of an ellipse is measured by its eccentricity (mathematics), eccentricity e, a number ranging from e = 0 (the Limiting case (mathematics), limiting case of a circle) to e = 1 (the limiting case of infinite elongation, no longer an ellipse but a parabola). An ellipse has a simple algebraic solution for its area, but only approximations for its perimeter (also known as circumference), for which integration is required to obtain an exact solution. Analytic geometry, Analytically, the equation of a standard ellipse centered at the origin with width 2a and height 2b is: : \frac+\frac = 1 . Assuming a \ge b, the foci are (\pm c, 0) for c = \sqrt. The standard parametric e ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Plane Geometry
Euclidean geometry is a mathematical system attributed to ancient Greek mathematician Euclid, which he described in his textbook on geometry: the '' Elements''. Euclid's approach consists in assuming a small set of intuitively appealing axioms (postulates) and deducing many other propositions (theorems) from these. Although many of Euclid's results had been stated earlier,. Euclid was the first to organize these propositions into a logical system in which each result is '' proved'' from axioms and previously proved theorems. The ''Elements'' begins with plane geometry, still taught in secondary school (high school) as the first axiomatic system and the first examples of mathematical proofs. It goes on to the solid geometry of three dimensions. Much of the ''Elements'' states results of what are now called algebra and number theory, explained in geometrical language. For more than two thousand years, the adjective "Euclidean" was unnecessary because no other sort of geometry h ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
History Of Mathematics
The history of mathematics deals with the origin of discoveries in mathematics and the mathematical methods and notation of the past. Before the modern age and the worldwide spread of knowledge, written examples of new mathematical developments have come to light only in a few locales. From 3000 BC the Mesopotamian states of Sumer, Akkad and Assyria, followed closely by Ancient Egypt and the Levantine state of Ebla began using arithmetic, algebra and geometry for purposes of taxation, commerce, trade and also in the patterns in nature, the field of astronomy and to record time and formulate calendars. The earliest mathematical texts available are from Mesopotamia and Egypt – '' Plimpton 322'' ( Babylonian c. 2000 – 1900 BC), the ''Rhind Mathematical Papyrus'' ( Egyptian c. 1800 BC) and the '' Moscow Mathematical Papyrus'' (Egyptian c. 1890 BC). All of these texts mention the so-called Pythagorean triples, so, by inference, the Pythagorean theorem seems to be the most anci ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Clifford Pickover
Clifford Alan Pickover (born August 15, 1957) is an American author, editor, and columnist in the fields of science, mathematics, science fiction, innovation, and creativity. For many years, he was employed at the IBM Thomas J. Watson Research Center in Yorktown, New York where he was Editor-in-Chief of the '' IBM Journal of Research and Development''. He has been granted more than 700 U.S. patents, is an elected Fellow for the Committee for Skeptical Inquiry, and is author of more than 50 books, translated into more than a dozen languages. Pickover.com Biography Pickover was elected as a Fellow for the Committee for Skeptical Inquiry for his “significant contributions to the general public’s understanding of science, reason, and critical inquiry through their scholarship, writing, and work in the media.” Other Fellows have included Carl Sagan and Isaac Asimov. He has been awarded almost 700 United States patents, and his '' The Math Book'' was winner of the 2011 ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
