|
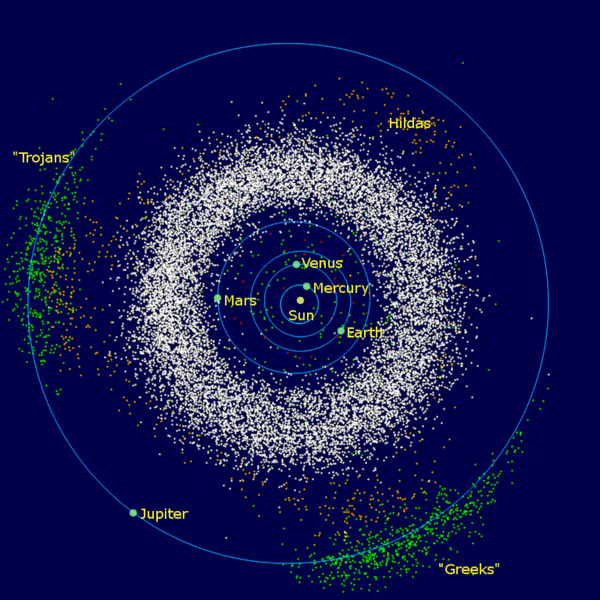
Hilda Asteroids
The Hilda asteroids (adj. ''Hildian'') are a dynamical group of more than 5,000 asteroids located beyond the asteroid belt but within Jupiter's orbit, in a 3:2 orbital resonance with Jupiter. The namesake is the asteroid 153 Hilda. Hildas move in their elliptical orbits in such a fashion that they arrive closest to Jupiter's orbit (i.e. at their aphelion) just when either one of Jupiter's , or Lagrange points arrives there. On their next orbit their aphelion will synchronize with the next Lagrange point in the –– sequence. Since , and are 120° apart, by the time a Hilda completes an orbit, Jupiter will have completed 360° − 120° or two-thirds of its own orbit. A Hilda's orbit has a semi-major axis between 3.7 and 4.2 AU (the average over a long time span is 3.97), an eccentricity less than 0.3, and an inclination less than 20°. Two collisional families exist within the Hilda group: the Hilda family and the Schubart family. The namesake for the latter fa ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
P-type Asteroid
P-type asteroids are asteroids that have low albedo and a featureless reddish spectrum. It has been suggested that they have a composition of organic rich silicates, carbon and anhydrous silicates, possibly with water ice in their interior. P-type asteroids are found in the outer asteroid belt and beyond. There are in the neighborhood of 33 known P-type asteroids, depending on the classification, including 46 Hestia, 65 Cybele, 76 Freia, 87 Sylvia, 153 Hilda, 476 Hedwig and, in some classifications, 107 Camilla. Taxonomy An early system of asteroid taxonomy was established in 1975 from the doctoral thesis work of David J. Tholen. This was based upon observations of a group of 110 asteroids. The U-type classification was used as a miscellaneous class for asteroids with unusual spectra that didn't fit into the C and S-type asteroid classifications. In 1976, some of these U-type asteroids with unusual moderate albedo levels were labeled as M-type. Around 1981, an offshoot of the ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Trojan Asteroid
In astronomy, a trojan is a small celestial body (mostly asteroids) that shares the orbit of a larger body, remaining in a stable orbit approximately 60° ahead of or behind the main body near one of its Lagrangian points and . Trojans can share the orbits of planets or of large moons. Trojans are one type of co-orbital object. In this arrangement, a star and a planet orbit about their common barycenter, which is close to the center of the star because it is usually much more massive than the orbiting planet. In turn, a much smaller mass than both the star and the planet, located at one of the Lagrangian points of the star–planet system, is subject to a combined gravitational force that acts through this barycenter. Hence the smallest object orbits around the barycenter with the same orbital period as the planet, and the arrangement can remain stable over time. In the Solar System, most known trojans share the orbit of Jupiter. They are divided into the Greek camp at (ah ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Perihelion
An apsis (; ) is the farthest or nearest point in the orbit of a planetary body about its primary body. For example, the apsides of the Earth are called the aphelion and perihelion. General description There are two apsides in any elliptic orbit. The name for each apsis is created from the prefixes ''ap-'', ''apo-'' (), or ''peri-'' (), each referring to the farthest and closest point to the primary body the affixing necessary suffix that describes the primary body in the orbit. In this case, the suffix for Earth is ''-gee'', so the apsides' names are ''apogee'' and ''perigee''. For the Sun, its suffix is ''-helion'', so the names are ''aphelion'' and ''perihelion''. According to Newton's laws of motion, all periodic orbits are ellipses. The barycenter of the two bodies may lie well within the bigger body—e.g., the Earth–Moon barycenter is about 75% of the way from Earth's center to its surface. If, compared to the larger mass, the smaller mass is negligible (e.g., f ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Aphelion
An apsis (; ) is the farthest or nearest point in the orbit of a planetary body about its primary body. For example, the apsides of the Earth are called the aphelion and perihelion. General description There are two apsides in any elliptic orbit. The name for each apsis is created from the prefixes ''ap-'', ''apo-'' (), or ''peri-'' (), each referring to the farthest and closest point to the primary body the affixing necessary suffix that describes the primary body in the orbit. In this case, the suffix for Earth is ''-gee'', so the apsides' names are ''apogee'' and ''perigee''. For the Sun, its suffix is ''-helion'', so the names are ''aphelion'' and ''perihelion''. According to Newton's laws of motion, all periodic orbits are ellipses. The barycenter of the two bodies may lie well within the bigger body—e.g., the Earth–Moon barycenter is about 75% of the way from Earth's center to its surface. If, compared to the larger mass, the smaller mass is negligible (e.g., f ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Equilateral
In geometry, an equilateral triangle is a triangle in which all three sides have the same length. In the familiar Euclidean geometry, an equilateral triangle is also equiangular; that is, all three internal angles are also congruent to each other and are each 60°. It is also a regular polygon, so it is also referred to as a regular triangle. Principal properties Denoting the common length of the sides of the equilateral triangle as a, we can determine using the Pythagorean theorem that: *The area is A=\frac a^2, *The perimeter is p=3a\,\! *The radius of the circumscribed circle is R = \frac *The radius of the inscribed circle is r=\frac a or r=\frac *The geometric center of the triangle is the center of the circumscribed and inscribed circles *The altitude (height) from any side is h=\frac a Denoting the radius of the circumscribed circle as ''R'', we can determine using trigonometry that: *The area of the triangle is \mathrm=\fracR^2 Many of these quantities have simple r ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Conjunction (astronomy And Astrology)
In astronomy, a conjunction occurs when two astronomical objects or spacecraft have either the same right ascension or the same ecliptic longitude, usually as observed from Earth. When two objects always appear close to the ecliptic—such as two planets, the Moon and a planet, or the Sun and a planet—this fact implies an apparent close approach between the objects as seen in the sky. A related word, ''appulse'', is the minimum apparent separation in the sky of two astronomical objects. Conjunctions involve either two objects in the Solar System or one object in the Solar System and a more distant object, such as a star. A conjunction is an apparent phenomenon caused by the observer's perspective: the two objects involved are not actually close to one another in space. Conjunctions between two bright objects close to the ecliptic, such as two bright planets, can be seen with the naked eye. The astronomical symbol for conjunction is (Unicode U+260C ☌). The conjunction sym ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Retrograde Motion
Retrograde motion in astronomy is, in general, orbital or rotational motion of an object in the direction opposite the rotation of its primary, that is, the central object (right figure). It may also describe other motions such as precession or nutation of an object's rotational axis. Prograde or direct motion is more normal motion in the same direction as the primary rotates. However, "retrograde" and "prograde" can also refer to an object other than the primary if so described. The direction of rotation is determined by an inertial frame of reference, such as distant fixed stars. In the Solar System, the orbits around the Sun of all planets and most other objects, except many comets, are prograde. They orbit around the Sun in the same direction as the sun rotates about its axis, which is counterclockwise when observed from above the Sun's north pole. Except for Venus and Uranus, planetary rotations around their axes are also prograde. Most natural satellites have prograde or ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Kepler's Laws Of Planetary Motion
In astronomy, Kepler's laws of planetary motion, published by Johannes Kepler between 1609 and 1619, describe the orbits of planets around the Sun. The laws modified the heliocentric theory of Nicolaus Copernicus, replacing its circular orbits and epicycles with elliptical trajectories, and explaining how planetary velocities vary. The three laws state that: # The orbit of a planet is an ellipse with the Sun at one of the two foci. # A line segment joining a planet and the Sun sweeps out equal areas during equal intervals of time. # The square of a planet's orbital period is proportional to the cube of the length of the semi-major axis of its orbit. The elliptical orbits of planets were indicated by calculations of the orbit of Mars. From this, Kepler inferred that other bodies in the Solar System, including those farther away from the Sun, also have elliptical orbits. The second law helps to establish that when a planet is closer to the Sun, it travels faster. The third law ex ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Astronomical Unit
The astronomical unit (symbol: au, or or AU) is a unit of length, roughly the distance from Earth to the Sun and approximately equal to or 8.3 light-minutes. The actual distance from Earth to the Sun varies by about 3% as Earth orbits the Sun, from a maximum (aphelion) to a minimum (perihelion) and back again once each year. The astronomical unit was originally conceived as the average of Earth's aphelion and perihelion; however, since 2012 it has been defined as exactly (see below for several conversions). The astronomical unit is used primarily for measuring distances within the Solar System or around other stars. It is also a fundamental component in the definition of another unit of astronomical length, the parsec. History of symbol usage A variety of unit symbols and abbreviations have been in use for the astronomical unit. In a 1976 resolution, the International Astronomical Union (IAU) had used the symbol ''A'' to denote a length equal to the astronomical ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Jupiter Trojan
The Jupiter trojans, commonly called trojan asteroids or simply trojans, are a large group of asteroids that share the planet Jupiter's orbit around the Sun. Relative to Jupiter, each trojan librates around one of Jupiter's stable Lagrange points: either ', existing 60° ahead of the planet in its orbit, or ', 60° behind. Jupiter trojans are distributed in two elongated, curved regions around these Lagrangian points with an average semi-major axis of about 5.2 AU. The first Jupiter trojan discovered, 588 Achilles, was spotted in 1906 by German astronomer Max Wolf. More than 9,800 Jupiter trojans have been found . By convention, they are each named from Greek mythology after a figure of the Trojan War, hence the name "trojan". The total number of Jupiter trojans larger than 1 km in diameter is believed to be about , approximately equal to the number of asteroids larger than 1 km in the asteroid belt. Like main-belt asteroids, Jupiter trojans form families. , man ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Orbital Period
The orbital period (also revolution period) is the amount of time a given astronomical object takes to complete one orbit around another object. In astronomy, it usually applies to planets or asteroids orbiting the Sun, moons orbiting planets, exoplanets orbiting other stars, or binary stars. For celestial objects in general, the sidereal period ( sidereal year) is referred to by the orbital period, determined by a 360° revolution of one body around its primary, e.g. Earth around the Sun, relative to the fixed stars projected in the sky. Orbital periods can be defined in several ways. The tropical period is more particularly about the position of the parent star. It is the basis for the solar year, and respectively the calendar year. The synodic period incorporates not only the orbital relation to the parent star, but also to other celestial objects, making it not a mere different approach to the orbit of an object around its parent, but a period of orbital relations ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |

.png)