|
Hesse Configuration
In geometry, the Hesse configuration, introduced by Colin Maclaurin and studied by , is a configuration of 9 points and 12 lines with three points per line and four lines through each point. It can be realized in the complex projective plane as the set of inflection points of an elliptic curve, but it has no realization in the Euclidean plane. Description The Hesse configuration has the same incidence relations as the lines and points of the affine plane over the field of 3 elements. That is, the points of the Hesse configuration may be identified with ordered pairs of numbers modulo 3, and the lines of the configuration may correspondingly be identified with the triples of points satisfying a linear equation . Alternatively, the points of the configuration may be identified by the squares of a tic-tac-toe board, and the lines may be identified with the lines and broken diagonals of the board. Each point belongs to four lines: in the tic tac toe interpretation of the configura ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hesse Configuration
In geometry, the Hesse configuration, introduced by Colin Maclaurin and studied by , is a configuration of 9 points and 12 lines with three points per line and four lines through each point. It can be realized in the complex projective plane as the set of inflection points of an elliptic curve, but it has no realization in the Euclidean plane. Description The Hesse configuration has the same incidence relations as the lines and points of the affine plane over the field of 3 elements. That is, the points of the Hesse configuration may be identified with ordered pairs of numbers modulo 3, and the lines of the configuration may correspondingly be identified with the triples of points satisfying a linear equation . Alternatively, the points of the configuration may be identified by the squares of a tic-tac-toe board, and the lines may be identified with the lines and broken diagonals of the board. Each point belongs to four lines: in the tic tac toe interpretation of the configura ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hessian Group
In mathematics, the Hessian group is a finite group of order 216, introduced by who named it for Otto Hesse. It may be represented as the group of affine transformations with determinant 1 of the affine plane over the field of 3 elements.Hessian group oGroupNames/ref> It has a normal subgroup that is an elementary abelian group of order 32, and the quotient by this subgroup is isomorphic to the group SL2(3) of order 24. It also acts on the Hesse pencil of elliptic curves, and forms the automorphism group of the Hesse configuration of the 9 inflection points of these curves and the 12 lines through triples of these points. The triple cover of this group is a complex reflection group In mathematics, a complex reflection group is a finite group acting on a finite-dimensional complex vector space that is generated by complex reflections: non-trivial elements that fix a complex hyperplane pointwise. Complex reflection groups arise ..., 3 sub>3 sub>3 or of order 648, and the product of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Discrete And Computational Geometry
'' Discrete & Computational Geometry'' is a peer-reviewed mathematics journal published quarterly by Springer. Founded in 1986 by Jacob E. Goodman and Richard M. Pollack, the journal publishes articles on discrete geometry and computational geometry. Abstracting and indexing The journal is indexed in: * ''Mathematical Reviews'' * ''Zentralblatt MATH'' * ''Science Citation Index'' * ''Current Contents''/Engineering, Computing and Technology Notable articles The articles by Gil Kalai with a proof of a subexponential upper bound on the diameter of a polyhedron and by Samuel Ferguson on the Kepler conjecture, both published in Discrete & Computational geometry, earned their author the Fulkerson Prize The Fulkerson Prize for outstanding papers in the area of discrete mathematics is sponsored jointly by the Mathematical Optimization Society (MOS) and the American Mathematical Society (AMS). Up to three awards of $1,500 each are presented at e .... References External link ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Sylvester–Gallai Theorem
The Sylvester–Gallai theorem in geometry states that every finite set of points in the Euclidean plane has a line that passes through exactly two of the points or a line that passes through all of them. It is named after James Joseph Sylvester, who posed it as a problem in 1893, and Tibor Gallai, who published one of the first proofs of this theorem in 1944. A line that contains exactly two of a set of points is known as an ''ordinary line''. Another way of stating the theorem is that every finite set of points that is not collinear has an ordinary line. According to a strengthening of the theorem, every finite point set (not all on one line) has at least a linear number of ordinary lines. An algorithm can find an ordinary line in a set of n points in time O(n\log n). History The Sylvester–Gallai theorem was posed as a problem by . suggests that Sylvester may have been motivated by a related phenomenon in algebraic geometry, in which the inflection points of a cubic curve ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Sylvester–Gallai Configuration
In geometry, a Sylvester–Gallai configuration consists of a finite subset of the points of a projective space with the property that the line through any two of the points in the subset also passes through at least one other point of the subset. Instead of defining Sylvester–Gallai configurations as subsets of the points of a projective space, they may be defined as abstract incidence structures of points and lines, satisfying the properties that, for every pair of points, the structure includes exactly one line containing the pair and that every line contains at least three points. In this more general form they are also called Sylvester–Gallai designs. A closely related concept is a Sylvester matroid, a matroid with the same property as a Sylvester–Gallai configuration of having no two-point lines. Real and complex embeddability In the Euclidean plane, the real projective plane, higher-dimensional Euclidean spaces or real projective spaces, or spaces with coordinates in a ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
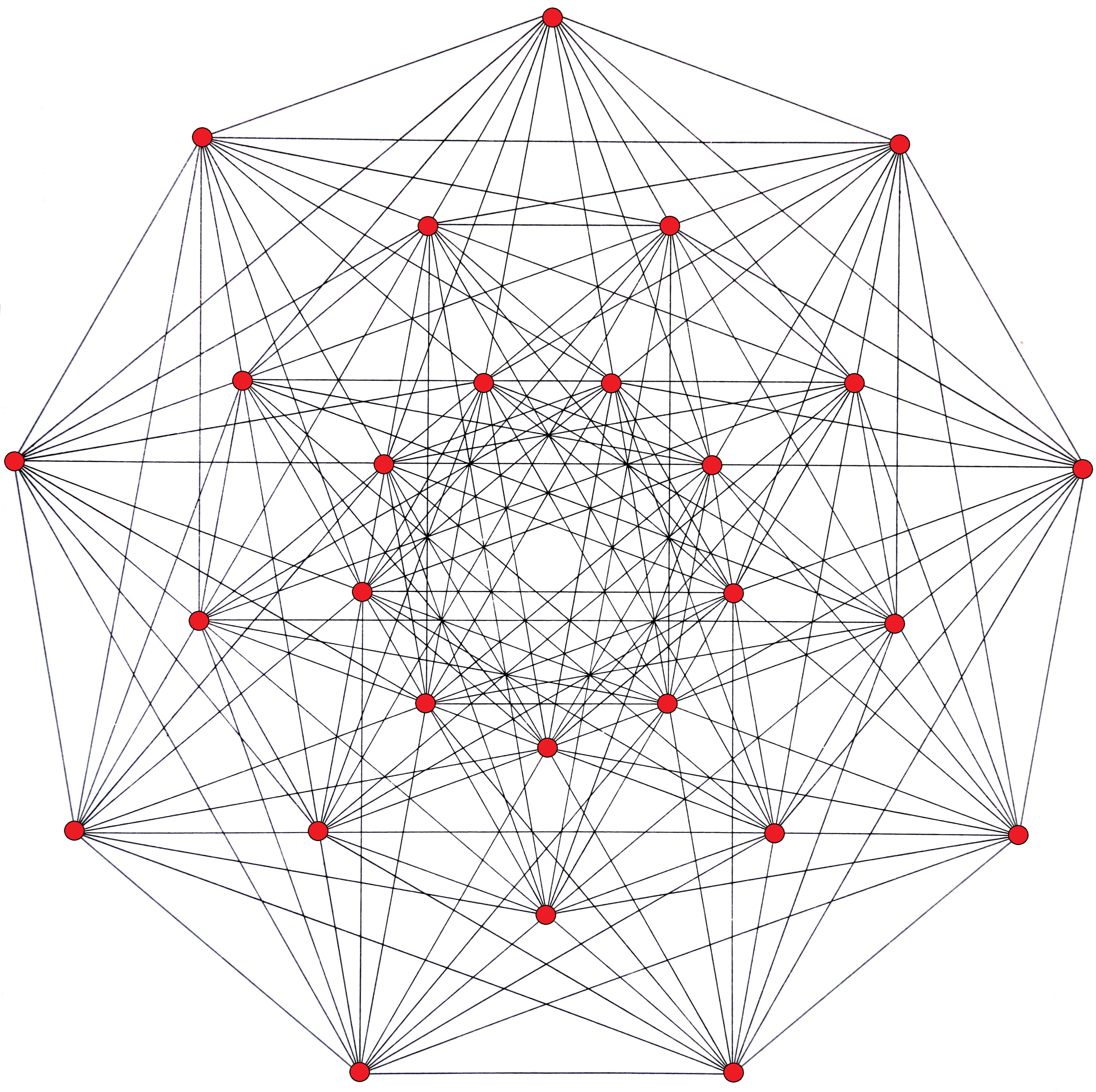
Hessian Polyhedron
In geometry, the Hessian polyhedron is a regular complex polyhedron 333, , in \mathbb^3. It has 27 vertices, 72 3 edges, and 27 33 faces. It is self-dual. Coxeter named it after Ludwig Otto Hesse for sharing the ''Hessian configuration'' \left begin 9&4\\3&12 \end\right /math> or (94123), 9 points lying by threes on twelve lines, with four lines through each point. Its complex reflection group is 3 sub>3 sub>3 or , order 648, also called a Hessian group. It has 27 copies of , order 24, at each vertex. It has 24 order-3 reflections. Its Coxeter number is 12, with degrees of the fundamental invariants 3, 6, and 12, which can be seen in projective symmetry of the polytopes. The Witting polytope, 3333, contains the Hessian polyhedron as cells and vertex figures. It has a real representation as the ''221'' polytope, , in 6-dimensional space, sharing the same 27 vertices. The 216 edges in ''221'' can be seen as the 72 3 edges represented as 3 simple edges. Coordinates Its 27 ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hesse Pencil
In mathematics, the syzygetic pencil or Hesse pencil, named for Otto Hesse, is a pencil (one-dimensional family) of cubic plane elliptic curves in the complex projective plane, defined by the equation :\lambda(x^3+y^3+z^3) + \mu xyz =0. Each curve in the family is determined by a pair of parameter values (\lambda,\mu) (not both zero) and consists of the points in the plane whose homogeneous coordinates (x,y,z) satisfy the equation for those parameters. Multiplying both \lambda and \mu by the same scalar does not change the curve, so there is only one degree of freedom in selecting a curve from the pencil, but the two-parameter form given above allows either \lambda or \mu (but not both) to be set to zero. Each curve in the pencil passes through the nine points of the complex projective plane whose homogeneous coordinates are some permutation of 0, –1, and a cube root of unity. There are three roots of unity, and six permutations per root, giving 18 choices for the homogeneous coor ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hessian Matrix
In mathematics, the Hessian matrix or Hessian is a square matrix of second-order partial derivatives of a scalar-valued function, or scalar field. It describes the local curvature of a function of many variables. The Hessian matrix was developed in the 19th century by the German mathematician Ludwig Otto Hesse and later named after him. Hesse originally used the term "functional determinants". Definitions and properties Suppose f : \R^n \to \R is a function taking as input a vector \mathbf \in \R^n and outputting a scalar f(\mathbf) \in \R. If all second-order partial derivatives of f exist, then the Hessian matrix \mathbf of f is a square n \times n matrix, usually defined and arranged as follows: \mathbf H_f= \begin \dfrac & \dfrac & \cdots & \dfrac \\ .2ex \dfrac & \dfrac & \cdots & \dfrac \\ .2ex \vdots & \vdots & \ddots & \vdots \\ .2ex \dfrac & \dfrac & \cdots & \dfrac \end, or, by stating an equation for the coefficients using indices i and j, (\mathbf H_f)_ = \fra ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Pencil (mathematics)
In geometry, a pencil is a family of geometric objects with a common property, for example the set of lines that pass through a given point in a plane, or the set of circles that pass through two given points in a plane. Although the definition of a pencil is rather vague, the common characteristic is that the pencil is completely determined by any two of its members. Analogously, a set of geometric objects that are determined by any three of its members is called a bundle. Thus, the set of all lines through a point in three-space is a bundle of lines, any two of which determine a pencil of lines. To emphasize the two dimensional nature of such a pencil, it is sometimes referred to as a ''flat pencil''. Any geometric object can be used in a pencil. The common ones are lines, planes, circles, conics, spheres, and general curves. Even points can be used. A pencil of points is the set of all points on a given line. A more common term for this set is a ''range'' of points. Penci ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Projective Plane
In mathematics, a projective plane is a geometric structure that extends the concept of a plane. In the ordinary Euclidean plane, two lines typically intersect in a single point, but there are some pairs of lines (namely, parallel lines) that do not intersect. A projective plane can be thought of as an ordinary plane equipped with additional "points at infinity" where parallel lines intersect. Thus ''any'' two distinct lines in a projective plane intersect at exactly one point. Renaissance artists, in developing the techniques of drawing in perspective, laid the groundwork for this mathematical topic. The archetypical example is the real projective plane, also known as the extended Euclidean plane. This example, in slightly different guises, is important in algebraic geometry, topology and projective geometry where it may be denoted variously by , RP2, or P2(R), among other notations. There are many other projective planes, both infinite, such as the complex projective plane, ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Pappus Configuration
In geometry, the Pappus configuration is a configuration of nine points and nine lines in the Euclidean plane, with three points per line and three lines through each point. History and construction This configuration is named after Pappus of Alexandria. Pappus's hexagon theorem states that every two triples of collinear points ''ABC'' and ''abc'' (none of which lie on the intersection of the two lines) can be completed to form a Pappus configuration, by adding the six lines ''Ab'', ''aB'', ''Ac'', ''aC'', ''Bc'', and ''bC'', and their three intersection points , , and . These three points are the intersection points of the "opposite" sides of the hexagon ''AbCaBc''. According to Pappus' theorem, the resulting system of nine points and eight lines always has a ninth line containing the three intersection points ''X'', ''Y'', and ''Z'', called the ''Pappus line''. The Pappus configuration can also be derived from two triangles ''XcC'' and ''YbB'' that are in perspective with e ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Bulletin Of The American Mathematical Society
The ''Bulletin of the American Mathematical Society'' is a quarterly mathematical journal published by the American Mathematical Society. Scope It publishes surveys on contemporary research topics, written at a level accessible to non-experts. It also publishes, by invitation only, book reviews and short ''Mathematical Perspectives'' articles. History It began as the ''Bulletin of the New York Mathematical Society'' and underwent a name change when the society became national. The Bulletin's function has changed over the years; its original function was to serve as a research journal for its members. Indexing The Bulletin is indexed in Mathematical Reviews, Science Citation Index, ISI Alerting Services, CompuMath Citation Index, and Current Contents/Physical, Chemical & Earth Sciences. See also *'' Journal of the American Mathematical Society'' *''Memoirs of the American Mathematical Society'' *''Notices of the American Mathematical Society'' *'' Proceedings of the American M ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
