|
Herringbone Pattern
The herringbone pattern is an arrangement of rectangles used for floor tilings and road pavement, so named for a fancied resemblance to the bones of a fish such as a herring. The blocks can be rectangles or parallelograms. The block edge length ratios are usually 2:1, and sometimes 3:1, but need not be even ratios. The herringbone pattern has a symmetry of wallpaper group pgg, as long as the blocks are not of different color (i.e., considering the borders alone). Herringbone patterns can be found in wallpaper, mosaics, seating, cloth and clothing ( herringbone cloth), shoe tread, security printing, herringbone gears, jewellery, sculpture, and elsewhere. Examples Related tilings As a geometric tessellation, the herringbone pattern is topologically identical to the regular hexagonal tiling. This can be seen if the rectangular blocks are distorted slightly. In parquetry, more casually known as flooring, herringbone patterns can be accomplished in wood, brick, and tile. Su ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
90 Degree Herringbone Bond
9 (nine) is the natural number following and preceding . Evolution of the Arabic digit In the beginning, various Indians wrote a digit 9 similar in shape to the modern closing question mark without the bottom dot. The Kshatrapa, Andhra and Gupta started curving the bottom vertical line coming up with a -look-alike. The Nagari continued the bottom stroke to make a circle and enclose the 3-look-alike, in much the same way that the sign @ encircles a lowercase ''a''. As time went on, the enclosing circle became bigger and its line continued beyond the circle downwards, as the 3-look-alike became smaller. Soon, all that was left of the 3-look-alike was a squiggle. The Arabs simply connected that squiggle to the downward stroke at the middle and subsequent European change was purely cosmetic. While the shape of the glyph for the digit 9 has an ascender in most modern typefaces, in typefaces with text figures the character usually has a descender, as, for example, in . The mod ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
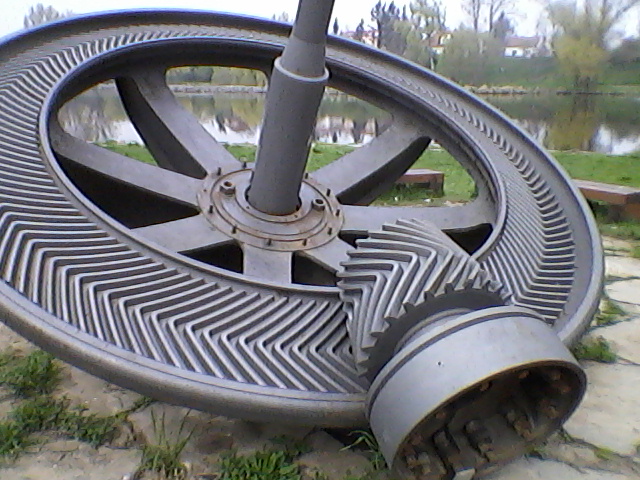
Herringbone Gear
A herringbone gear, a specific type of double helical gear, is a special type of gear that is a side-to-side (not face-to-face) combination of two Gear#Helical, helical gears of opposite Helix#Handedness, hands. From the top, each helical Groove (engineering), groove of this gear looks like the letter V, and many together form a herringbone pattern (resembling the fish anatomy#Vertebrae, bones of a fish such as a herring). Unlike helical gears, herringbone gears do not produce an additional axial load. Like helical gears, they have the advantage of transferring power smoothly, because more than two teeth will be enmeshed at any moment in time. Their advantage over the helical gears is that the side-thrust of one half is balanced by that of the other half. This means that herringbone gears can be used in torque gearboxes without requiring a substantial thrust bearing. Because of this, herringbone gears were an important step in the introduction of the steam turbine to marine propul ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Isohedral Tiling P6-8
In geometry, a tessellation of dimension (a plane tiling) or higher, or a polytope of dimension (a polyhedron) or higher, is isohedral or face-transitive if all its faces are the same. More specifically, all faces must be not merely congruent but must be ''transitive'', i.e. must lie within the same '' symmetry orbit''. In other words, for any two faces and , there must be a symmetry of the ''entire'' figure by translations, rotations, and/or reflections that maps onto . For this reason, convex isohedral polyhedra are the shapes that will make fair dice. Isohedral polyhedra are called isohedra. They can be described by their face configuration. An isohedron has an even number of faces. The dual of an isohedral polyhedron is vertex-transitive, i.e. isogonal. The Catalan solids, the bipyramids, and the trapezohedra are all isohedral. They are the duals of the (isogonal) Archimedean solids, prisms, and antiprisms, respectively. The Platonic solids, which are either self-dual ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Isohedral Tiling P4-19
In geometry, a tessellation of dimension (a plane tiling) or higher, or a polytope of dimension (a polyhedron) or higher, is isohedral or face-transitive if all its faces are the same. More specifically, all faces must be not merely congruent but must be ''transitive'', i.e. must lie within the same '' symmetry orbit''. In other words, for any two faces and , there must be a symmetry of the ''entire'' figure by translations, rotations, and/or reflections that maps onto . For this reason, convex isohedral polyhedra are the shapes that will make fair dice. Isohedral polyhedra are called isohedra. They can be described by their face configuration. An isohedron has an even number of faces. The dual of an isohedral polyhedron is vertex-transitive, i.e. isogonal. The Catalan solids, the bipyramids, and the trapezohedra are all isohedral. They are the duals of the (isogonal) Archimedean solids, prisms, and antiprisms, respectively. The Platonic solids, which are either self-dual ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hexagonal Tiling
In geometry, the hexagonal tiling or hexagonal tessellation is a regular tiling of the Euclidean plane, in which exactly three hexagons meet at each vertex. It has Schläfli symbol of or (as a truncated triangular tiling). English mathematician John Conway called it a hextille. The internal angle of the hexagon is 120 degrees, so three hexagons at a point make a full 360 degrees. It is one of three regular tilings of the plane. The other two are the triangular tiling and the square tiling. Applications The hexagonal tiling is the densest way to arrange circles in two dimensions. The honeycomb conjecture states that the hexagonal tiling is the best way to divide a surface into regions of equal area with the least total perimeter. The optimal three-dimensional structure for making honeycomb (or rather, soap bubbles) was investigated by Lord Kelvin, who believed that the Kelvin structure (or body-centered cubic lattice) is optimal. However, the less regular Weaire–Phel ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Tessellation
A tessellation or tiling is the covering of a surface, often a plane (mathematics), plane, using one or more geometric shapes, called ''tiles'', with no overlaps and no gaps. In mathematics, tessellation can be generalized to high-dimensional spaces, higher dimensions and a variety of geometries. A periodic tiling has a repeating pattern. Some special kinds include ''regular tilings'' with regular polygonal tiles all of the same shape, and ''semiregular tilings'' with regular tiles of more than one shape and with every corner identically arranged. The patterns formed by periodic tilings can be categorized into 17 wallpaper groups. A tiling that lacks a repeating pattern is called "non-periodic". An ''aperiodic tiling'' uses a small set of tile shapes that cannot form a repeating pattern. A ''tessellation of space'', also known as a space filling or honeycomb, can be defined in the geometry of higher dimensions. A real physical tessellation is a tiling made of materials such a ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Geometry
Geometry (; ) is, with arithmetic, one of the oldest branches of mathematics. It is concerned with properties of space such as the distance, shape, size, and relative position of figures. A mathematician who works in the field of geometry is called a ''geometer''. Until the 19th century, geometry was almost exclusively devoted to Euclidean geometry, which includes the notions of point, line, plane, distance, angle, surface, and curve, as fundamental concepts. During the 19th century several discoveries enlarged dramatically the scope of geometry. One of the oldest such discoveries is Carl Friedrich Gauss' ("remarkable theorem") that asserts roughly that the Gaussian curvature of a surface is independent from any specific embedding in a Euclidean space. This implies that surfaces can be studied ''intrinsically'', that is, as stand-alone spaces, and has been expanded into the theory of manifolds and Riemannian geometry. Later in the 19th century, it appeared that geometries ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Budapest, Hungary
Budapest (, ; ) is the capital and most populous city of Hungary. It is the ninth-largest city in the European Union by population within city limits and the second-largest city on the Danube river; the city has an estimated population of 1,752,286 over a land area of about . Budapest, which is both a city and county, forms the centre of the Budapest metropolitan area, which has an area of and a population of 3,303,786; it is a primate city, constituting 33% of the population of Hungary. The history of Budapest began when an early Celtic settlement transformed into the Roman town of Aquincum, the capital of Lower Pannonia. The Hungarians arrived in the territory in the late 9th century, but the area was pillaged by the Mongols in 1241–42. Re-established Buda became one of the centres of Renaissance humanist culture by the 15th century. The Battle of Mohács, in 1526, was followed by nearly 150 years of Ottoman rule. After the reconquest of Buda in 1686, the region en ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Salzburg, Austria
Salzburg (, ; literally "Salt-Castle"; bar, Soizbuag, label=Bavarian language, Austro-Bavarian) is the List of cities and towns in Austria, fourth-largest city in Austria. In 2020, it had a population of 156,872. The town is on the site of the Roman settlement of ''Iuvavum''. Salzburg was founded as an episcopal see in 696 and became a Prince-Archbishopric of Salzburg, seat of the archbishop in 798. Its main sources of income were salt extraction, trade, and gold mining. The fortress of Hohensalzburg Fortress, Hohensalzburg, one of the largest medieval fortresses in Europe, dates from the 11th century. In the 17th century, Salzburg became a center of the Counter-Reformation, with monasteries and numerous Baroque churches built. Historic Centre of the City of Salzburg, Salzburg's historic center (German language, German: ''Altstadt'') is renowned for its Baroque architecture and is one of the best-preserved city centers north of the Alps. The historic center was enlisted as a UN ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Wallpaper Group-pgg-2
Wallpaper is a material used in interior decoration to decorate the interior walls of domestic and public buildings. It is usually sold in rolls and is applied onto a wall using wallpaper paste. Wallpapers can come plain as "lining paper" (so that it can be painted or used to help cover uneven surfaces and minor wall defects thus giving a better surface), textured (such as Anaglypta), with a regular repeating pattern design, or, much less commonly today, with a single non-repeating large design carried over a set of sheets. The smallest rectangle that can be tiled to form the whole pattern is known as the pattern repeat. Wallpaper printing techniques include surface printing, gravure printing, silk screen-printing, rotary printing, and digital printing. Wallpaper is made in long rolls which are hung vertically on a wall. Patterned wallpapers are designed so that the pattern "repeats", and thus pieces cut from the same roll can be hung next to each other so as to continue the p ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Wallpaper Group-pg-2
Wallpaper is a material used in interior decoration to decorate the interior walls of domestic and public buildings. It is usually sold in rolls and is applied onto a wall using wallpaper paste. Wallpapers can come plain as "lining paper" (so that it can be painted or used to help cover uneven surfaces and minor wall defects thus giving a better surface), textured (such as Anaglypta), with a regular repeating pattern design, or, much less commonly today, with a single non-repeating large design carried over a set of sheets. The smallest rectangle that can be tiled to form the whole pattern is known as the pattern repeat. Wallpaper printing techniques include surface printing, gravure printing, silk screen-printing, rotary printing, and digital printing. Wallpaper is made in long rolls which are hung vertically on a wall. Patterned wallpapers are designed so that the pattern "repeats", and thus pieces cut from the same roll can be hung next to each other so as to continue the p ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Wallpaper Group-pg-1
Wallpaper is a material used in interior decoration to decorate the interior walls of domestic and public buildings. It is usually sold in rolls and is applied onto a wall using wallpaper paste. Wallpapers can come plain as "lining paper" (so that it can be painted or used to help cover uneven surfaces and minor wall defects thus giving a better surface), textured (such as Anaglypta), with a regular repeating pattern design, or, much less commonly today, with a single non-repeating large design carried over a set of sheets. The smallest rectangle that can be tiled to form the whole pattern is known as the pattern repeat. Wallpaper printing techniques include surface printing, gravure printing, silk screen-printing, rotary printing, and digital printing. Wallpaper is made in long rolls which are hung vertically on a wall. Patterned wallpapers are designed so that the pattern "repeats", and thus pieces cut from the same roll can be hung next to each other so as to continue the p ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
.svg "Click for more on -> Salzburg, Austria")
