|
Grigoriy Yablonsky
Grigoriy Yablonsky (or Yablonskii) (russian: Григорий Семенович Яблонский) is an expert in the area of chemical kinetics and chemical engineering, particularly in catalytic technology of complete and selective oxidation, which is one of the main driving forces of sustainable development. His theory of complex steady-state and non-steady state catalytic reactions, is widely used by research teams in many countries of the world (USA, UK, Belgium, Germany, France, Norway and Thailand). Now, Grigoriy Yablonsky serves as an Associate Research Professor of Chemistry at Saint Louis University’s Parks College of Engineering, Aviation and Technology and SLU’s College of Arts and Sciences. Since 2006, Yablonsky is an editor of the Russian-Americaalmanac "Middle West" Some recent scientific achievements Yablonsky – together with Lazman, developed the general form of steady-state kinetic description (‘kinetic polynomial’) which is a non-linear genera ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Yessentuki
Yessentuki ( rus, Ессентуки́, p=jɪsɪntʊˈkʲiˑ) is a types of inhabited localities in Russia, city in Stavropol Krai, Russia, located in the shadow of Mount Elbrus at the base of the Caucasus Mountains. The city serves as a railway station in the Mineralnye Vody—Kislovodsk branch, and is located southwest of Mineralnye Vody and west of Pyatigorsk. It is considered the cultural capital of Greeks in Russia and the Soviet Union, Russia's Greek population and even today close to ten percent of its population is of Greek descent. Population: History Research by the Soviet archaeologist M.E. Masson and excavations of eight mausoleums showed that there was a large Golden Horde settlement near the present-day Essentuki in the 13th-15th centuries. Masson believed that the name Essentuki came from the name of a certain Khan Essentug from the names "Yesan Forest" and "Yesan Field" that have survived to this day. In 1798, the Russian military and border redoubt of Yessentu ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Detailed Balance
The principle of detailed balance can be used in kinetic systems which are decomposed into elementary processes (collisions, or steps, or elementary reactions). It states that at equilibrium, each elementary process is in equilibrium with its reverse process. History The principle of detailed balance was explicitly introduced for collisions by Ludwig Boltzmann. In 1872, he proved his H-theorem using this principle.Boltzmann, L. (1964), Lectures on gas theory, Berkeley, CA, USA: U. of California Press. The arguments in favor of this property are founded upon microscopic reversibility. Tolman, R. C. (1938). ''The Principles of Statistical Mechanics''. Oxford University Press, London, UK. Five years before Boltzmann, James Clerk Maxwell used the principle of detailed balance for gas kinetics with the reference to the principle of sufficient reason. He compared the idea of detailed balance with other types of balancing (like cyclic balance) and found that "Now it is impossible to as ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Linear Span
In mathematics, the linear span (also called the linear hull or just span) of a set of vectors (from a vector space), denoted , pp. 29-30, §§ 2.5, 2.8 is defined as the set of all linear combinations of the vectors in . It can be characterized either as the intersection of all linear subspaces that contain , or as the smallest subspace containing . The linear span of a set of vectors is therefore a vector space itself. Spans can be generalized to matroids and modules. To express that a vector space is a linear span of a subset , one commonly uses the following phrases—either: spans , is a spanning set of , is spanned/generated by , or is a generator or generator set of . Definition Given a vector space over a field , the span of a set of vectors (not necessarily infinite) is defined to be the intersection of all subspaces of that contain . is referred to as the subspace ''spanned by'' , or by the vectors in . Conversely, is called a ''spanning set'' of , and we ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Convex Hull
In geometry, the convex hull or convex envelope or convex closure of a shape is the smallest convex set that contains it. The convex hull may be defined either as the intersection of all convex sets containing a given subset of a Euclidean space, or equivalently as the set of all convex combinations of points in the subset. For a bounded subset of the plane, the convex hull may be visualized as the shape enclosed by a rubber band stretched around the subset. Convex hulls of open sets are open, and convex hulls of compact sets are compact. Every compact convex set is the convex hull of its extreme points. The convex hull operator is an example of a closure operator, and every antimatroid can be represented by applying this closure operator to finite sets of points. The algorithmic problems of finding the convex hull of a finite set of points in the plane or other low-dimensional Euclidean spaces, and its dual problem of intersecting half-spaces, are fundamental problems of com ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Detailed Balance
The principle of detailed balance can be used in kinetic systems which are decomposed into elementary processes (collisions, or steps, or elementary reactions). It states that at equilibrium, each elementary process is in equilibrium with its reverse process. History The principle of detailed balance was explicitly introduced for collisions by Ludwig Boltzmann. In 1872, he proved his H-theorem using this principle.Boltzmann, L. (1964), Lectures on gas theory, Berkeley, CA, USA: U. of California Press. The arguments in favor of this property are founded upon microscopic reversibility. Tolman, R. C. (1938). ''The Principles of Statistical Mechanics''. Oxford University Press, London, UK. Five years before Boltzmann, James Clerk Maxwell used the principle of detailed balance for gas kinetics with the reference to the principle of sufficient reason. He compared the idea of detailed balance with other types of balancing (like cyclic balance) and found that "Now it is impossible to as ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Microscopic Reversibility
The principle of microscopic reversibility in physics and chemistry is twofold: * First, it states that the microscopic detailed dynamics of particles and fields is time-reversible because the microscopic equations of motion are symmetric with respect to inversion in time (T-symmetry); * Second, it relates to the statistical description of the kinetics of macroscopic or mesoscopic systems as an ensemble of elementary processes: collisions, elementary transitions or reactions. For these processes, the consequence of the microscopic T-symmetry is: ''Corresponding to every individual process there is a reverse process, and in a state of equilibrium the average rate of every process is equal to the average rate of its reverse process.'' History of microscopic reversibility The idea of microscopic reversibility was born together with physical kinetics. In 1872, Ludwig Boltzmann represented kinetics of gases as statistical ensemble of elementary collisions.Boltzmann, L. (1964), Lectures on ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Dynamical System
In mathematics, a dynamical system is a system in which a Function (mathematics), function describes the time dependence of a Point (geometry), point in an ambient space. Examples include the mathematical models that describe the swinging of a clock pendulum, fluid dynamics, the flow of water in a pipe, the Brownian motion, random motion of particles in the air, and population dynamics, the number of fish each springtime in a lake. The most general definition unifies several concepts in mathematics such as ordinary differential equations and ergodic theory by allowing different choices of the space and how time is measured. Time can be measured by integers, by real number, real or complex numbers or can be a more general algebraic object, losing the memory of its physical origin, and the space may be a manifold or simply a Set (mathematics), set, without the need of a Differentiability, smooth space-time structure defined on it. At any given time, a dynamical system has a State ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Mass Action Law
In chemistry, the law of mass action is the proposition that the rate of the chemical reaction is directly proportional to the product of the activities or concentrations of the reactants. It explains and predicts behaviors of solutions in dynamic equilibrium. Specifically, it implies that for a chemical reaction mixture that is in equilibrium, the ratio between the concentration of reactants and products is constant. Two aspects are involved in the initial formulation of the law: 1) the equilibrium aspect, concerning the composition of a reaction mixture at equilibrium and 2) the kinetic aspect concerning the rate equations for elementary reactions. Both aspects stem from the research performed by Cato M. Guldberg and Peter Waage between 1864 and 1879 in which equilibrium constants were derived by using kinetic data and the rate equation which they had proposed. Guldberg and Waage also recognized that chemical equilibrium is a dynamic process in which rates of reaction fo ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Reaction Rate
The reaction rate or rate of reaction is the speed at which a chemical reaction takes place, defined as proportional to the increase in the concentration of a product per unit time and to the decrease in the concentration of a reactant per unit time. Reaction rates can vary dramatically. For example, the oxidative rusting of iron under Earth's atmosphere is a slow reaction that can take many years, but the combustion of cellulose in a fire is a reaction that takes place in fractions of a second. For most reactions, the rate decreases as the reaction proceeds. A reaction's rate can be determined by measuring the changes in concentration over time. Chemical kinetics is the part of physical chemistry that concerns how rates of chemical reactions are measured and predicted, and how reaction-rate data can be used to deduce probable reaction mechanisms. The concepts of chemical kinetics are applied in many disciplines, such as chemical engineering, enzymology and environmental engin ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Eley–Rideal Mechanism
Reactions on surfaces are reactions in which at least one of the steps of the reaction mechanism is the adsorption of one or more reactants. The mechanisms for these reactions, and the rate equations are of extreme importance for heterogeneous catalysis. Via scanning tunneling microscopy, it is possible to observe reactions at the solid gas interface in real space, if the time scale of the reaction is in the correct range. Reactions at the solid–gas interface are in some cases related to catalysis. Simple decomposition If a reaction occurs through these steps: : A + S ⇌ AS → Products where A is the reactant and S is an adsorption site on the surface and the respective rate constants for the adsorption, desorption and reaction are ''k''1, ''k''−1 and ''k''2, then the global reaction rate is: :r=k_2 C_\mathrm=k_2 \theta C_\mathrm where: * ''r'' is the rate, mol·''m''−2·s−1 *C_Ais the concentration of adsorbate, ''mol·m−3'' *C_\mathrm is the surface concentrati ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
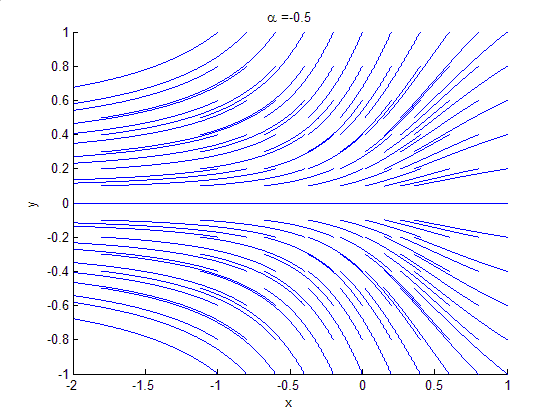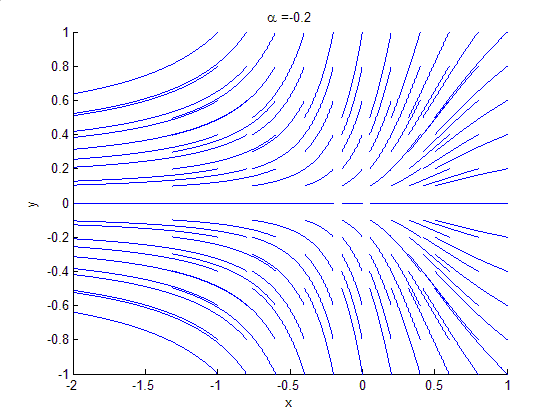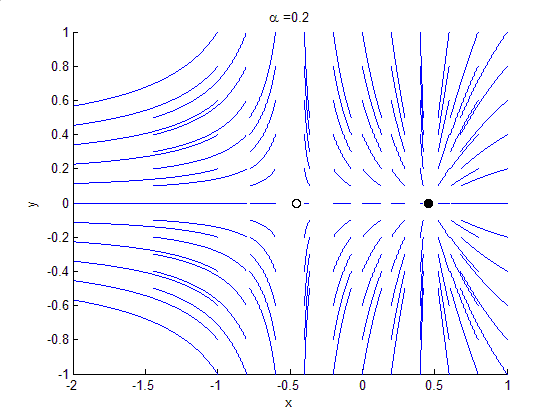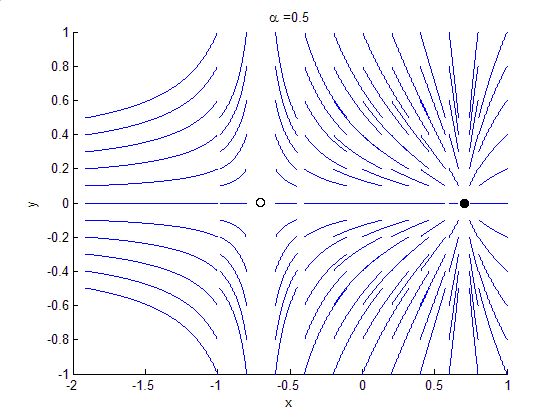
Bifurcation Theory
Bifurcation theory is the mathematical study of changes in the qualitative or topological structure of a given family of curves, such as the integral curves of a family of vector fields, and the solutions of a family of differential equations. Most commonly applied to the mathematical study of dynamical systems, a bifurcation occurs when a small smooth change made to the parameter values (the bifurcation parameters) of a system causes a sudden 'qualitative' or topological change in its behavior. Bifurcations occur in both continuous systems (described by ordinary, delay or partial differential equations) and discrete systems (described by maps). The name "bifurcation" was first introduced by Henri Poincaré in 1885 in the first paper in mathematics showing such a behavior. Henri Poincaré also later named various types of stationary points and classified them . Bifurcation types It is useful to divide bifurcations into two principal classes: * Local bifurcations, which can b ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Phase Portrait
A phase portrait is a geometric representation of the trajectories of a dynamical system in the phase plane. Each set of initial conditions is represented by a different curve, or point. Phase portraits are an invaluable tool in studying dynamical systems. They consist of a plot of typical trajectories in the state space. This reveals information such as whether an attractor, a repellor or limit cycle is present for the chosen parameter value. The concept of topological equivalence is important in classifying the behaviour of systems by specifying when two different phase portraits represent the same qualitative dynamic behavior. An attractor is a stable point which is also called "sink". The repeller is considered as an unstable point, which is also known as "source". A phase portrait graph of a dynamical system depicts the system's trajectories (with arrows) and stable steady states (with dots) and unstable steady states (with circles) in a state space. The axes are of st ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |