|
Green–Kubo Relations
The Green–Kubo relations ( Melville S. Green 1954, Ryogo Kubo 1957) give the exact mathematical expression for transport coefficients \gamma in terms of integrals of time correlation functions: :\gamma = \int_0^\infty \left\langle \dot(t) \dot(0) \right\rangle \;t. Thermal and mechanical transport processes Thermodynamic systems may be prevented from relaxing to equilibrium because of the application of a field (e.g. electric or magnetic field), or because the boundaries of the system are in relative motion (shear) or maintained at different temperatures, etc. This generates two classes of nonequilibrium system: mechanical nonequilibrium systems and thermal nonequilibrium systems. The standard example of an electrical transport process is Ohm's law, which states that, at least for sufficiently small applied voltages, the current ''I'' is linearly proportional to the applied voltage ''V'', : I = \sigma V.\, As the applied voltage increases one expects to see deviations from ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Melville S
Melville may refer to: Places Antarctica *Cape Melville (South Shetland Islands) *Melville Peak, King George Island * Melville Glacier, Graham Land * Melville Highlands, Laurie Island * Melville Point, Marie Byrd Land Australia *Cape Melville, Queensland *City of Melville, Western Australia, the local government authority *Electoral district of Melville, Western Australia * Melville Bay, Northern Territory *Melville Island, Northern Territory *Melville, Western Australia, a suburb of Perth Canada *Melville, Saskatchewan, a city *Melville (electoral district), Saskatchewan, a federal electoral district *Melville (provincial electoral district), Saskatchewan *Melville, a community within the town of Caledon, Ontario *Melville Peninsula, Nunavut *Melville Sound, Nunavut *Melville Island (Northwest Territories and Nunavut) *Melville Island (Nova Scotia), in Halifax Harbour *Melville Cove, Halifax, in Halifax Harbour *Melville Island, a small island in the Discovery Islands, British ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Fluctuation Theorem
The fluctuation theorem (FT), which originated from statistical mechanics, deals with the relative probability that the entropy of a system which is currently away from thermodynamic equilibrium (i.e., maximum entropy) will increase or decrease over a given amount of time. While the second law of thermodynamics predicts that the entropy of an isolated system should tend to increase until it reaches equilibrium, it became apparent after the discovery of statistical mechanics that the second law is only a statistical one, suggesting that there should always be some nonzero probability that the entropy of an isolated system might spontaneously ''decrease''; the fluctuation theorem precisely quantifies this probability. Statement Roughly, the fluctuation theorem relates to the probability distribution of the time-averaged irreversible entropy production, denoted \overline_t. The theorem states that, in systems away from equilibrium over a finite time ''t'', the ratio between the probab ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Thermodynamic Equations
Thermodynamics is expressed by a mathematical framework of ''thermodynamic equations'' which relate various thermodynamic quantities and physical properties measured in a laboratory or production process. Thermodynamics is based on a fundamental set of postulates, that became the laws of thermodynamics. Introduction One of the fundamental thermodynamic equations is the description of thermodynamic work in analogy to mechanical work, or weight lifted through an elevation against gravity, as defined in 1824 by French physicist Sadi Carnot. Carnot used the phrase motive power for work. In the footnotes to his famous ''On the Motive Power of Fire'', he states: “We use here the expression ''motive power'' to express the useful effect that a motor is capable of producing. This effect can always be likened to the elevation of a weight to a certain height. It has, as we know, as a measure, the product of the weight multiplied by the height to which it is raised.” With the inc ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Theoretical Physics
Theoretical physics is a branch of physics that employs mathematical models and abstractions of physical objects and systems to rationalize, explain and predict natural phenomena. This is in contrast to experimental physics, which uses experimental tools to probe these phenomena. The advancement of science generally depends on the interplay between experimental studies and theory. In some cases, theoretical physics adheres to standards of mathematical rigour while giving little weight to experiments and observations.There is some debate as to whether or not theoretical physics uses mathematics to build intuition and illustrativeness to extract physical insight (especially when normal experience fails), rather than as a tool in formalizing theories. This links to the question of it using mathematics in a less formally rigorous, and more intuitive or heuristic way than, say, mathematical physics. For example, while developing special relativity, Albert Einstein was concerned wit ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Linear Response Function
A linear response function describes the input-output relationship of a signal transducer such as a radio turning electromagnetic waves into music or a neuron turning synaptic input into a response. Because of its many applications in information theory, physics and engineering there exist alternative names for specific linear response functions such as susceptibility, impulse response or impedance, see also transfer function. The concept of a Green's function or fundamental solution of an ordinary differential equation is closely related. Mathematical definition Denote the input of a system by h(t) (e.g. a force), and the response of the system by x(t) (e.g. a position). Generally, the value of x(t) will depend not only on the present value of h(t), but also on past values. Approximately x(t) is a weighted sum of the previous values of h(t'), with the weights given by the linear response function \chi(t-t'): x(t) = \int_^t dt'\, \chi(t-t') h(t') + \cdots\,. The explicit term o ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Lindblad Equation
In quantum mechanics, the Gorini–Kossakowski–Sudarshan–Lindblad equation (GKSL equation, named after Vittorio Gorini, Andrzej Kossakowski, George Sudarshan and Göran Lindblad), master equation in Lindblad form, quantum Liouvillian, or Lindbladian is one of the general forms of Markovian and time-homogeneous master equations describing the (in general non-unitary) evolution of the density matrix that preserves the laws of quantum mechanics (i.e., is trace-preserving and completely positive for any initial condition). The Schrödinger equation is a special case of the more general Lindblad equation, which has led to some speculation that quantum mechanics may be productively extended and expanded through further application and analysis of the Lindblad equation. The Schrödinger equation deals with state vectors, which can only describe pure quantum states and are thus less general than density matrices, which can describe mixed states as well. Motivation In the ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Green's Function (many-body Theory)
In many-body theory, the term Green's function (or Green function) is sometimes used interchangeably with correlation function, but refers specifically to correlators of field operators or creation and annihilation operators. The name comes from the Green's functions used to solve inhomogeneous differential equations, to which they are loosely related. (Specifically, only two-point 'Green's functions' in the case of a non-interacting system are Green's functions in the mathematical sense; the linear operator that they invert is the Hamiltonian operator, which in the non-interacting case is quadratic in the fields.) Spatially uniform case Basic definitions We consider a many-body theory with field operator (annihilation operator written in the position basis) \psi(\mathbf). The Heisenberg operators can be written in terms of Schrödinger operators as \psi(\mathbf,t) = e^ \psi(\mathbf) e^, and the creation operator is \bar\psi(\mathbf,t) = psi(\mathbf,t)\dagger, where K = H - ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Fluctuation–dissipation Theorem
The fluctuation–dissipation theorem (FDT) or fluctuation–dissipation relation (FDR) is a powerful tool in statistical physics for predicting the behavior of systems that obey detailed balance. Given that a system obeys detailed balance, the theorem is a proof that thermodynamic fluctuations in a physical variable predict the response quantified by the admittance or impedance (to be intended in their general sense, not only in electromagnetic terms) of the same physical variable (like voltage, temperature difference, etc.), and vice versa. The fluctuation–dissipation theorem applies both to classical and quantum mechanical systems. The fluctuation–dissipation theorem was proven by Herbert Callen and Theodore Welton in 1951 and expanded by Ryogo Kubo. There are antecedents to the general theorem, including Einstein's explanation of Brownian motion during his ''annus mirabilis'' and Harry Nyquist's explanation in 1928 of Johnson noise in electrical resistors. Qualitat ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Fluctuation Theorem
The fluctuation theorem (FT), which originated from statistical mechanics, deals with the relative probability that the entropy of a system which is currently away from thermodynamic equilibrium (i.e., maximum entropy) will increase or decrease over a given amount of time. While the second law of thermodynamics predicts that the entropy of an isolated system should tend to increase until it reaches equilibrium, it became apparent after the discovery of statistical mechanics that the second law is only a statistical one, suggesting that there should always be some nonzero probability that the entropy of an isolated system might spontaneously ''decrease''; the fluctuation theorem precisely quantifies this probability. Statement Roughly, the fluctuation theorem relates to the probability distribution of the time-averaged irreversible entropy production, denoted \overline_t. The theorem states that, in systems away from equilibrium over a finite time ''t'', the ratio between the probab ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Density Matrix
In quantum mechanics, a density matrix (or density operator) is a matrix that describes the quantum state of a physical system. It allows for the calculation of the probabilities of the outcomes of any measurement performed upon this system, using the Born rule. It is a generalization of the more usual state vectors or wavefunctions: while those can only represent pure states, density matrices can also represent ''mixed states''. Mixed states arise in quantum mechanics in two different situations: first when the preparation of the system is not fully known, and thus one must deal with a statistical ensemble of possible preparations, and second when one wants to describe a physical system which is entangled with another, without describing their combined state. Density matrices are thus crucial tools in areas of quantum mechanics that deal with mixed states, such as quantum statistical mechanics, open quantum systems, quantum decoherence, and quantum information. Definition and ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
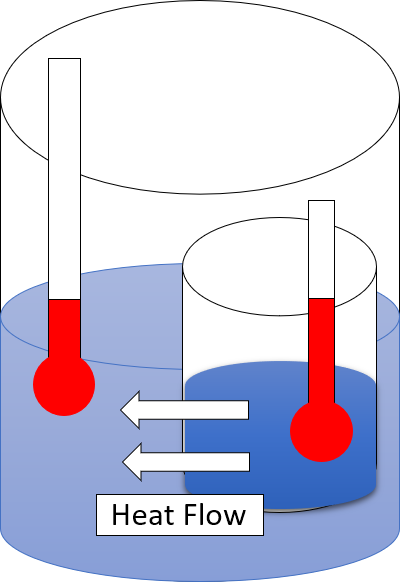
Second Law Of Thermodynamics
The second law of thermodynamics is a physical law based on universal experience concerning heat and Energy transformation, energy interconversions. One simple statement of the law is that heat always moves from hotter objects to colder objects (or "downhill"), unless energy in some form is supplied to reverse the direction of heat flow. Another definition is: "Not all heat energy can be converted into Work (thermodynamics), work in a cyclic process."Young, H. D; Freedman, R. A. (2004). ''University Physics'', 11th edition. Pearson. p. 764. The second law of thermodynamics in other versions establishes the concept of entropy as a physical property of a thermodynamic system. It can be used to predict whether processes are forbidden despite obeying the requirement of conservation of energy as expressed in the first law of thermodynamics and provides necessary criteria for spontaneous processes. The second law may be formulated by the observation that the entropy of isolated systems ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Central Limit Theorem
In probability theory, the central limit theorem (CLT) establishes that, in many situations, when independent random variables are summed up, their properly normalized sum tends toward a normal distribution even if the original variables themselves are not normally distributed. The theorem is a key concept in probability theory because it implies that probabilistic and statistical methods that work for normal distributions can be applicable to many problems involving other types of distributions. This theorem has seen many changes during the formal development of probability theory. Previous versions of the theorem date back to 1811, but in its modern general form, this fundamental result in probability theory was precisely stated as late as 1920, thereby serving as a bridge between classical and modern probability theory. If X_1, X_2, \dots, X_n, \dots are random samples drawn from a population with overall mean \mu and finite variance and if \bar_n is the sample mean of t ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |