|
Great Comet Of 1264
The Great Comet of 1264 (C/1264 N1) was one of the brightest comets on record. It appeared in July 1264 and remained visible to the end of September. It was first seen during the evenings after sunset, but appeared in its greatest splendor in weeks afterward, when it became visible during the mornings in the northeastern sky, with the tail perceived long before the comet itself rose above the horizon. The head of the comet seemed like an obscure and ill-defined star, and the tail passed from this portion of it like expanded flames, stretching forth towards the mid-heavens to a distance of one hundred degrees from the nucleus. The comet of 1264 was described to have been an object of great size and brilliancy. The comet's splendor was greatest at the end of August and the beginning of September. At that time, when the head was just visible above the eastern horizon in the morning sky, the tail stretched out past the mid-heaven towards the west, or was nearly 100° in length. The chr ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Great Comet Of 1264
The Great Comet of 1264 (C/1264 N1) was one of the brightest comets on record. It appeared in July 1264 and remained visible to the end of September. It was first seen during the evenings after sunset, but appeared in its greatest splendor in weeks afterward, when it became visible during the mornings in the northeastern sky, with the tail perceived long before the comet itself rose above the horizon. The head of the comet seemed like an obscure and ill-defined star, and the tail passed from this portion of it like expanded flames, stretching forth towards the mid-heavens to a distance of one hundred degrees from the nucleus. The comet of 1264 was described to have been an object of great size and brilliancy. The comet's splendor was greatest at the end of August and the beginning of September. At that time, when the head was just visible above the eastern horizon in the morning sky, the tail stretched out past the mid-heaven towards the west, or was nearly 100° in length. The chr ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Ellipse
In mathematics, an ellipse is a plane curve surrounding two focus (geometry), focal points, such that for all points on the curve, the sum of the two distances to the focal points is a constant. It generalizes a circle, which is the special type of ellipse in which the two focal points are the same. The elongation of an ellipse is measured by its eccentricity (mathematics), eccentricity e, a number ranging from e = 0 (the Limiting case (mathematics), limiting case of a circle) to e = 1 (the limiting case of infinite elongation, no longer an ellipse but a parabola). An ellipse has a simple algebraic solution for its area, but only approximations for its perimeter (also known as circumference), for which integration is required to obtain an exact solution. Analytic geometry, Analytically, the equation of a standard ellipse centered at the origin with width 2a and height 2b is: : \frac+\frac = 1 . Assuming a \ge b, the foci are (\pm c, 0) for c = \sqrt. The standard parametric e ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
13th Century In Science
In music or music theory, a thirteenth is the note thirteen scale degrees from the root of a chord and also the interval between the root and the thirteenth. The interval can be also described as a compound sixth, spanning an octave plus a sixth. The thirteenth is most commonly major or minor . A thirteenth chord is the stacking of six (major or minor) thirds, the last being above the 11th of an eleventh chord. Thus a thirteenth chord is a tertian (built from thirds) chord containing the interval of a thirteenth, and is an extended chord if it includes the ninth and/or the eleventh. "The jazzy thirteenth is a very versatile chord and is used in many genres." Since 13th chords tend to become unclear or confused with other chords when inverted, they are generally found in root position.Benward & Saker (2009). ''Music in Theory and Practice: Volume II'', p.179. Eighth Edition. . For example, depending on voicing, a major triad with an added major sixth is usually cal ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
1264
Year 1264 ( MCCLXIV) was a leap year starting on Tuesday (link will display the full calendar) of the Julian calendar. Events By place Byzantine Empire * Spring – Battle of Makryplagi: Constantine Palaiologos, half-brother of Emperor Michael III (Palaiologos), resumes operations against the Principality of Achaea. He advances up in northern Elis, and sets up his camp at a location called "St. Nicholas of Mesiskli". Prince William II of Villehardouin with his own troops march to meet him and arrays his men ready for battle. The Byzantine vanguard under Michael Kantakouzenos, ride forth from the Byzantine lines, but the force is ambushed and Michael is killed by the Achaeans. Constantine retreats and goes on to lay siege to the fortress of Nikli. There, Turkish mercenaries (some 1,000 horsemen), confront him and demand that he pay them their arrears of 6 months. Constantine refuses, whereupon the Turkish troops desert to William. He decides to raise the siege and d ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Non-periodic Comets
A periodic function is a function that repeats its values at regular intervals. For example, the trigonometric functions, which repeat at intervals of 2\pi radians, are periodic functions. Periodic functions are used throughout science to describe oscillations, waves, and other phenomena that exhibit periodicity. Any function that is not periodic is called aperiodic. Definition A function is said to be periodic if, for some nonzero constant , it is the case that :f(x+P) = f(x) for all values of in the domain. A nonzero constant for which this is the case is called a period of the function. If there exists a least positive constant with this property, it is called the fundamental period (also primitive period, basic period, or prime period.) Often, "the" period of a function is used to mean its fundamental period. A function with period will repeat on intervals of length , and these intervals are sometimes also referred to as periods of the function. Geometrically, a ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
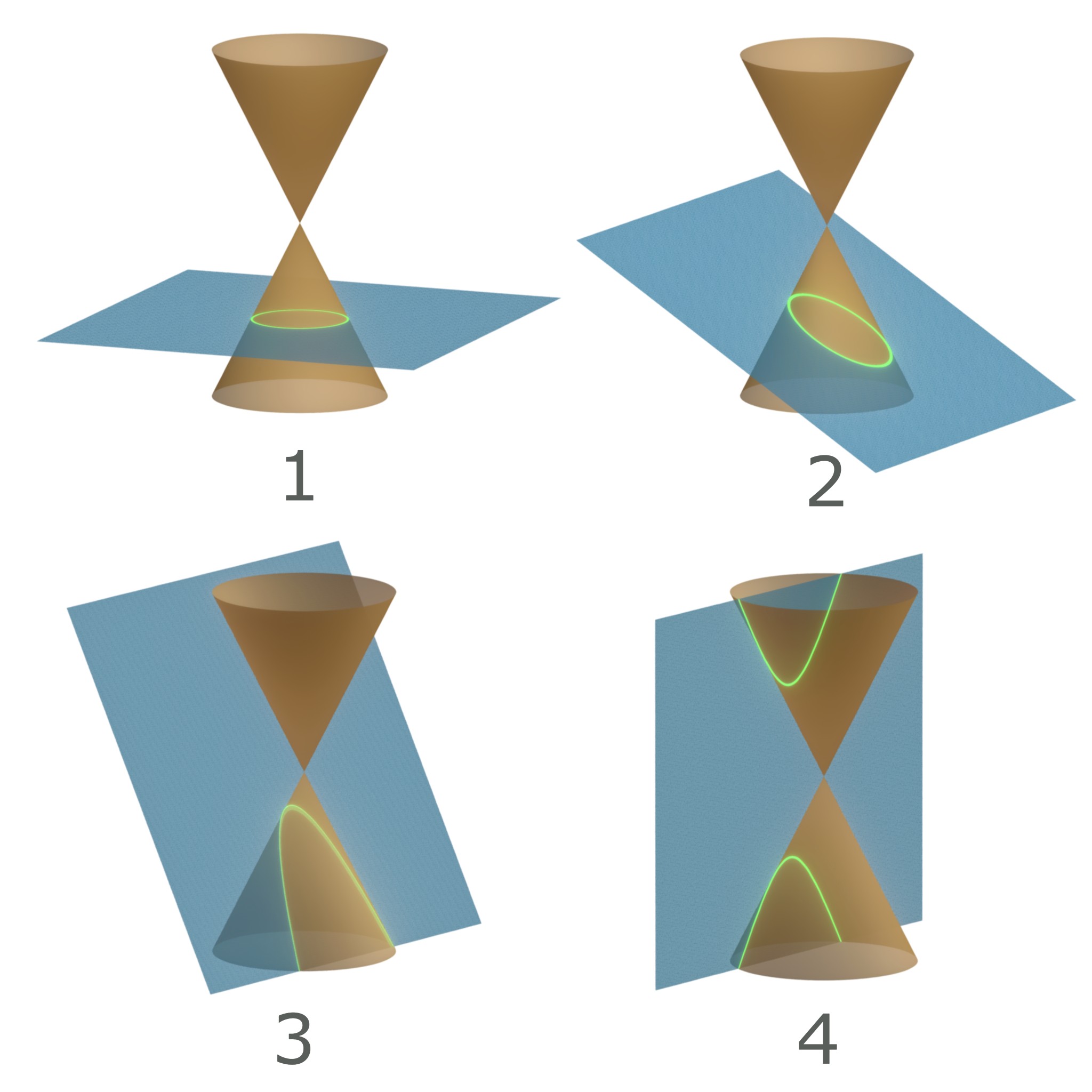
Conic Section
In mathematics, a conic section, quadratic curve or conic is a curve obtained as the intersection of the surface of a cone with a plane. The three types of conic section are the hyperbola, the parabola, and the ellipse; the circle is a special case of the ellipse, though historically it was sometimes called a fourth type. The ancient Greek mathematicians studied conic sections, culminating around 200 BC with Apollonius of Perga's systematic work on their properties. The conic sections in the Euclidean plane have various distinguishing properties, many of which can be used as alternative definitions. One such property defines a non-circular conic to be the set of those points whose distances to some particular point, called a ''focus'', and some particular line, called a ''directrix'', are in a fixed ratio, called the ''eccentricity''. The type of conic is determined by the value of the eccentricity. In analytic geometry, a conic may be defined as a plane algebraic curve of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Isaac Newton
Sir Isaac Newton (25 December 1642 – 20 March 1726/27) was an English mathematician, physicist, astronomer, alchemist, theologian, and author (described in his time as a "natural philosopher"), widely recognised as one of the greatest mathematicians and physicists and among the most influential scientists of all time. He was a key figure in the philosophical revolution known as the Enlightenment. His book (''Mathematical Principles of Natural Philosophy''), first published in 1687, established classical mechanics. Newton also made seminal contributions to optics, and shares credit with German mathematician Gottfried Wilhelm Leibniz for developing infinitesimal calculus. In the , Newton formulated the laws of motion and universal gravitation that formed the dominant scientific viewpoint for centuries until it was superseded by the theory of relativity. Newton used his mathematical description of gravity to derive Kepler's laws of planetary motion, account for ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hyperbola
In mathematics, a hyperbola (; pl. hyperbolas or hyperbolae ; adj. hyperbolic ) is a type of smooth curve lying in a plane, defined by its geometric properties or by equations for which it is the solution set. A hyperbola has two pieces, called connected components or branches, that are mirror images of each other and resemble two infinite bows. The hyperbola is one of the three kinds of conic section, formed by the intersection of a plane and a double cone. (The other conic sections are the parabola and the ellipse. A circle is a special case of an ellipse.) If the plane intersects both halves of the double cone but does not pass through the apex of the cones, then the conic is a hyperbola. Hyperbolas arise in many ways: * as the curve representing the reciprocal function y(x) = 1/x in the Cartesian plane, * as the path followed by the shadow of the tip of a sundial, * as the shape of an open orbit (as distinct from a closed elliptical orbit), such as the orbit of a s ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Parabola
In mathematics, a parabola is a plane curve which is mirror-symmetrical and is approximately U-shaped. It fits several superficially different mathematical descriptions, which can all be proved to define exactly the same curves. One description of a parabola involves a point (the focus) and a line (the directrix). The focus does not lie on the directrix. The parabola is the locus of points in that plane that are equidistant from both the directrix and the focus. Another description of a parabola is as a conic section, created from the intersection of a right circular conical surface and a plane parallel to another plane that is tangential to the conical surface. The line perpendicular to the directrix and passing through the focus (that is, the line that splits the parabola through the middle) is called the "axis of symmetry". The point where the parabola intersects its axis of symmetry is called the "vertex" and is the point where the parabola is most sharply curved. The ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Babinet , physics theorem
{{DEFAULTSORT:Babinet ...
Babinet is a surname. Notable people with the surname include: * Gilles Babinet (born 1967), French entrepreneur * Jacques Babinet (1794–1872), French scientist * Rémi Babinet (born 1957), French creative director Other uses * Babinet–Soleil compensator * Babinet's principle In physics, Babinet's principle states that the diffraction pattern from an opaque body is identical to that from a hole of the same size and shape except for the overall forward beam intensity. It was formulated in the 1800s by French physicist Ja ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Comet
A comet is an icy, small Solar System body that, when passing close to the Sun, warms and begins to release gases, a process that is called outgassing. This produces a visible atmosphere or coma, and sometimes also a tail. These phenomena are due to the effects of solar radiation and the solar wind acting upon the nucleus of the comet. Comet nuclei range from a few hundred meters to tens of kilometers across and are composed of loose collections of ice, dust, and small rocky particles. The coma may be up to 15 times Earth's diameter, while the tail may stretch beyond one astronomical unit. If sufficiently bright, a comet may be seen from Earth without the aid of a telescope and may subtend an arc of 30° (60 Moons) across the sky. Comets have been observed and recorded since ancient times by many cultures and religions. Comets usually have highly eccentric elliptical orbits, and they have a wide range of orbital periods, ranging from several years to potentially several mill ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Amédée Guillemin
Amédée Guillemin (born 5 July 1826 in Pierre-de-Bresse, died 2 January 1893 in Pierre-de-Bresse, France) was a French science writer and a journalist. Guillemin started his studies at Beaune Beaune () is the wine capital of Burgundy in the Côte d'Or department in eastern France. It is located between Lyon and Dijon. Beaune is one of the key wine centers in France, and the center of Burgundy wine production and business. The annua ... college before taking his final degree in Paris. From 1850 to 1860 he taught mathematics in a private school while writing articles for the Liberal press criticizing the Second French Empire. In 1860, he moved to Chambéry where he became a junior deputy editor of the weekly political magazine ''La Savoie''. After the Savoy#Annexation and opposition, annexation of Savoy by the French empire, he returned to Paris where he became the science editor of ''l’Avenir national'' (The Nation's Future). Guillemin presently started writing books o ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |



.jpg)
