|
Fourth-degree Equation
In algebra, a quartic function is a function of the form :f(x)=ax^4+bx^3+cx^2+dx+e, where ''a'' is nonzero, which is defined by a polynomial of degree four, called a quartic polynomial. A ''quartic equation'', or equation of the fourth degree, is an equation that equates a quartic polynomial to zero, of the form :ax^4+bx^3+cx^2+dx+e=0 , where . The derivative of a quartic function is a cubic function. Sometimes the term biquadratic is used instead of ''quartic'', but, usually, biquadratic function refers to a quadratic function of a square (or, equivalently, to the function defined by a quartic polynomial without terms of odd degree), having the form :f(x)=ax^4+cx^2+e. Since a quartic function is defined by a polynomial of even degree, it has the same infinite limit when the argument goes to positive or negative infinity. If ''a'' is positive, then the function increases to positive infinity at both ends; and thus the function has a global minimum. Likewise, if ''a'' is nega ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Computer-aided Manufacturing
Computer-aided manufacturing (CAM) also known as computer-aided modeling or computer-aided machining is the use of software to control machine tools in the manufacturing of work pieces. This is not the only definition for CAM, but it is the most common; CAM may also refer to the use of a computer to assist in all operations of a manufacturing plant, including planning, management, transportation and storage. Its primary purpose is to create a faster production process and components and tooling with more precise dimensions and material consistency, which in some cases, uses only the required amount of raw material (thus minimizing waste), while simultaneously reducing energy consumption. CAM is now a system used in schools and lower educational purposes. CAM is a subsequent computer-aided process after computer-aided design (CAD) and sometimes computer-aided engineering (CAE), as the model generated in CAD and verified in CAE can be input into CAM software, which then controls the ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Computer-aided Design
Computer-aided design (CAD) is the use of computers (or ) to aid in the creation, modification, analysis, or optimization of a design. This software is used to increase the productivity of the designer, improve the quality of design, improve communications through documentation, and to create a database for manufacturing. Designs made through CAD software are helpful in protecting products and inventions when used in patent applications. CAD output is often in the form of electronic files for print, machining, or other manufacturing operations. The terms computer-aided drafting (CAD) and computer aided design and drafting (CADD) are also used. Its use in designing electronic systems is known as '' electronic design automation'' (''EDA''). In mechanical design it is known as ''mechanical design automation'' (''MDA''), which includes the process of creating a technical drawing with the use of computer software. CAD software for mechanical design uses either vector-based graphics ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Computer Graphics
Computer graphics deals with generating images with the aid of computers. Today, computer graphics is a core technology in digital photography, film, video games, cell phone and computer displays, and many specialized applications. A great deal of specialized hardware and software has been developed, with the displays of most devices being driven by computer graphics hardware. It is a vast and recently developed area of computer science. The phrase was coined in 1960 by computer graphics researchers Verne Hudson and William Fetter of Boeing. It is often abbreviated as CG, or typically in the context of film as computer generated imagery (CGI). The non-artistic aspects of computer graphics are the subject of computer science research. Some topics in computer graphics include user interface design, sprite graphics, rendering, ray tracing, geometry processing, computer animation, vector graphics, 3D modeling, shaders, GPU design, implicit surfaces, visualization, scientific c ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Computational Geometry
Computational geometry is a branch of computer science devoted to the study of algorithms which can be stated in terms of geometry. Some purely geometrical problems arise out of the study of computational geometric algorithms, and such problems are also considered to be part of computational geometry. While modern computational geometry is a recent development, it is one of the oldest fields of computing with a history stretching back to antiquity. Analysis of algorithms, Computational complexity is central to computational geometry, with great practical significance if algorithms are used on very large datasets containing tens or hundreds of millions of points. For such sets, the difference between O(''n''2) and O(''n'' log ''n'') may be the difference between days and seconds of computation. The main impetus for the development of computational geometry as a discipline was progress in computer graphics and computer-aided design and manufacturing (Computer-aided design, CAD/Compu ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Torus
In geometry, a torus (plural tori, colloquially donut or doughnut) is a surface of revolution generated by revolving a circle in three-dimensional space about an axis that is coplanar with the circle. If the axis of revolution does not touch the circle, the surface has a ring shape and is called a torus of revolution. If the axis of revolution is tangent to the circle, the surface is a horn torus. If the axis of revolution passes twice through the circle, the surface is a spindle torus. If the axis of revolution passes through the center of the circle, the surface is a degenerate torus, a double-covered sphere. If the revolved curve is not a circle, the surface is called a ''toroid'', as in a square toroid. Real-world objects that approximate a torus of revolution include swim rings, inner tubes and ringette rings. Eyeglass lenses that combine spherical and cylindrical correction are toric lenses. A torus should not be confused with a '' solid torus'', which is formed by r ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
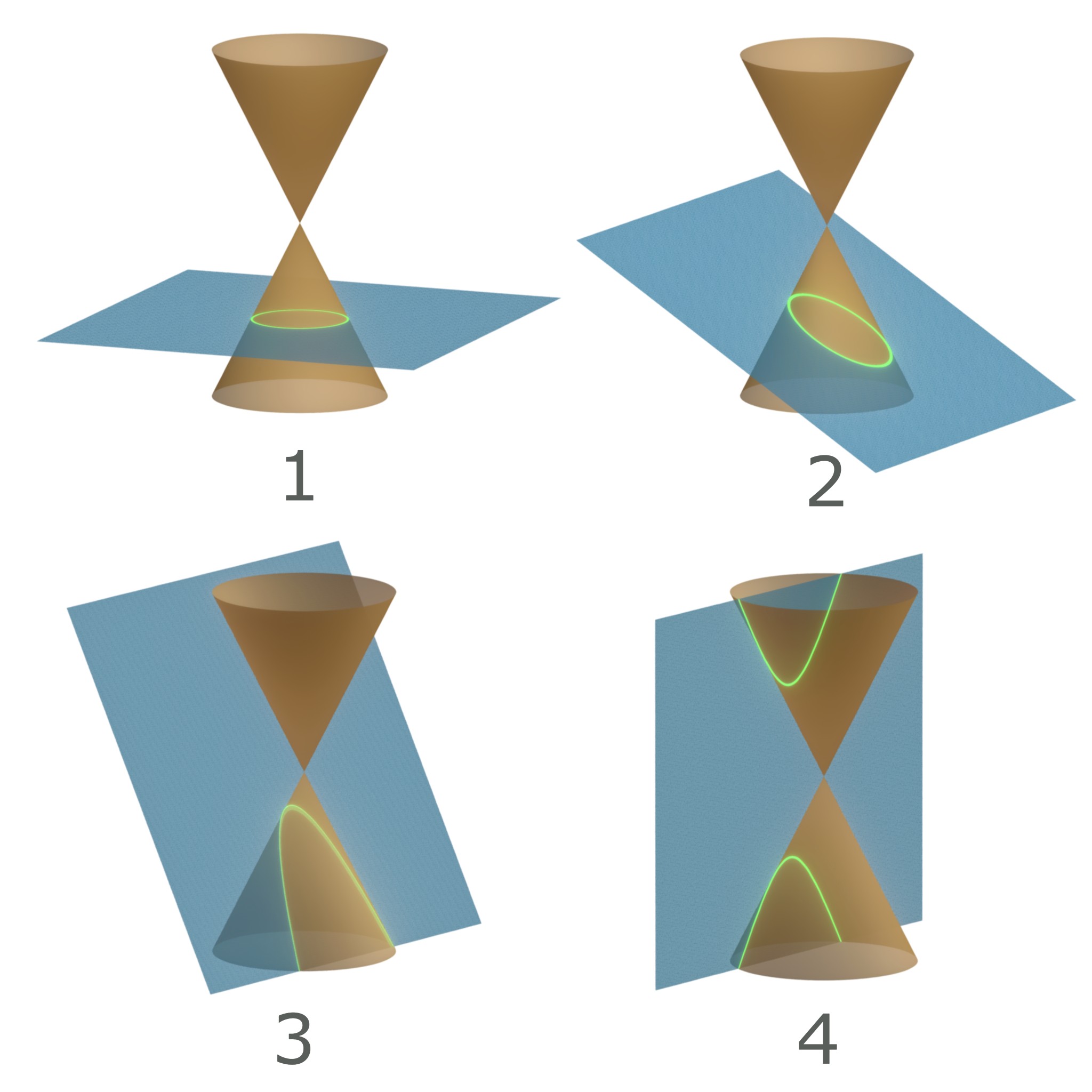
Conic Section
In mathematics, a conic section, quadratic curve or conic is a curve obtained as the intersection of the surface of a cone with a plane. The three types of conic section are the hyperbola, the parabola, and the ellipse; the circle is a special case of the ellipse, though historically it was sometimes called a fourth type. The ancient Greek mathematicians studied conic sections, culminating around 200 BC with Apollonius of Perga's systematic work on their properties. The conic sections in the Euclidean plane have various distinguishing properties, many of which can be used as alternative definitions. One such property defines a non-circular conic to be the set of those points whose distances to some particular point, called a ''focus'', and some particular line, called a ''directrix'', are in a fixed ratio, called the ''eccentricity''. The type of conic is determined by the value of the eccentricity. In analytic geometry, a conic may be defined as a plane algebraic curve of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Coordinate
In geometry, a coordinate system is a system that uses one or more numbers, or coordinates, to uniquely determine the position of the points or other geometric elements on a manifold such as Euclidean space. The order of the coordinates is significant, and they are sometimes identified by their position in an ordered tuple and sometimes by a letter, as in "the ''x''-coordinate". The coordinates are taken to be real numbers in elementary mathematics, but may be complex numbers or elements of a more abstract system such as a commutative ring. The use of a coordinate system allows problems in geometry to be translated into problems about numbers and ''vice versa''; this is the basis of analytic geometry. Common coordinate systems Number line The simplest example of a coordinate system is the identification of points on a line with real numbers using the ''number line''. In this system, an arbitrary point ''O'' (the ''origin'') is chosen on a given line. The coordinate of a po ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Galois Theory
In mathematics, Galois theory, originally introduced by Évariste Galois, provides a connection between field theory and group theory. This connection, the fundamental theorem of Galois theory, allows reducing certain problems in field theory to group theory, which makes them simpler and easier to understand. Galois introduced the subject for studying roots of polynomials. This allowed him to characterize the polynomial equations that are solvable by radicals in terms of properties of the permutation group of their roots—an equation is ''solvable by radicals'' if its roots may be expressed by a formula involving only integers, th roots, and the four basic arithmetic operations. This widely generalizes the Abel–Ruffini theorem, which asserts that a general polynomial of degree at least five cannot be solved by radicals. Galois theory has been used to solve classic problems including showing that two problems of antiquity cannot be solved as they were stated (doubling the cub ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Évariste Galois
Évariste Galois (; ; 25 October 1811 – 31 May 1832) was a French mathematician and political activist. While still in his teens, he was able to determine a necessary and sufficient condition for a polynomial to be solvable by radicals, thereby solving a problem that had been open for 350 years. His work laid the foundations for Galois theory and group theory, two major branches of abstract algebra. He was a staunch republican and was heavily involved in the political turmoil that surrounded the French Revolution of 1830. As a result of his political activism, he was arrested repeatedly, serving one jail sentence of several months. For reasons that remain obscure, shortly after his release from prison he fought in a duel and died of the wounds he suffered. Life Early life Galois was born on 25 October 1811 to Nicolas-Gabriel Galois and Adélaïde-Marie (née Demante). His father was a Republican and was head of Bourg-la-Reine's liberal party. His father became may ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Petr Beckmann
Petr Beckmann (November 13, 1924 – August 3, 1993) was a professor of electrical engineering who became a well-known advocate of libertarianism and nuclear power. Later in his life he disputed Albert Einstein's theory of relativity and other accepted theories in modern physics. Biography In 1939, when Beckmann was 14, his family fled their home in Prague, Czechoslovakia to escape the Nazis. From 1942 to 1945, he served in a Czech squadron of the Royal Air Force. He worked as a radar mechanic on the newly invented radar systems that helped Britain win the Battle of the Atlantic. He received a B.Sc. in 1949, a Ph.D. in 1955, and a D.Sc. in 1962, all from Prague's Czech Academy of Sciences in electrical engineering. He defected to the United States in 1963 and became a Professor (later, Emeritus) of electrical engineering at the University of Colorado. In the United States, he became acquainted with novelist Ayn Rand, a contributing editor to a publication devoted to her id ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |