|
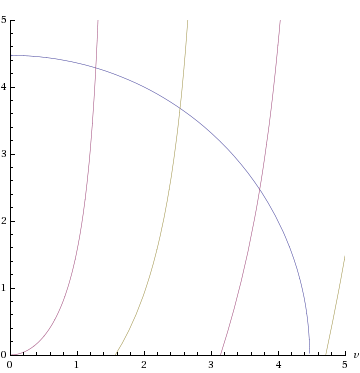
Finite Potential Well
The finite potential well (also known as the finite square well) is a concept from quantum mechanics. It is an extension of the infinite potential well, in which a particle is confined to a "box", but one which has finite potential "walls". Unlike the infinite potential well, there is a probability associated with the particle being found outside the box. The quantum mechanical interpretation is unlike the classical interpretation, where if the total energy of the particle is less than the potential energy barrier of the walls it cannot be found outside the box. In the quantum interpretation, there is a non-zero probability of the particle being outside the box even when the energy of the particle is less than the potential energy barrier of the walls (cf quantum tunnelling). Particle in a 1-dimensional box For the 1-dimensional case on the ''x''-axis, the time-independent Schrödinger equation can be written as: where *\hbar = \frac is the reduced Planck's constant, *h is Plan ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Quantum Mechanics
Quantum mechanics is a fundamental theory in physics that provides a description of the physical properties of nature at the scale of atoms and subatomic particles. It is the foundation of all quantum physics including quantum chemistry, quantum field theory, quantum technology, and quantum information science. Classical physics, the collection of theories that existed before the advent of quantum mechanics, describes many aspects of nature at an ordinary (macroscopic) scale, but is not sufficient for describing them at small (atomic and subatomic) scales. Most theories in classical physics can be derived from quantum mechanics as an approximation valid at large (macroscopic) scale. Quantum mechanics differs from classical physics in that energy, momentum, angular momentum, and other quantities of a bound system are restricted to discrete values ( quantization); objects have characteristics of both particles and waves (wave–particle duality); and there are limits to ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Prentice-Hall
Prentice Hall was an American major educational publisher owned by Savvas Learning Company. Prentice Hall publishes print and digital content for the 6–12 and higher-education market, and distributes its technical titles through the Safari Books Online e-reference service. History On October 13, 1913, law professor Charles Gerstenberg and his student Richard Ettinger founded Prentice Hall. Gerstenberg and Ettinger took their mothers' maiden names, Prentice and Hall, to name their new company. Prentice Hall became known as a publisher of trade books by authors such as Norman Vincent Peale; elementary, secondary, and college textbooks; loose-leaf information services; and professional books. Prentice Hall acquired the training provider Deltak in 1979. Prentice Hall was acquired by Gulf+Western in 1984, and became part of that company's publishing division Simon & Schuster. S&S sold several Prentice Hall subsidiaries: Deltak and Resource Systems were sold to National Education ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Rectangular Potential Barrier
In quantum mechanics, the rectangular (or, at times, square) potential barrier is a standard one-dimensional problem that demonstrates the phenomena of wave-mechanical tunneling (also called "quantum tunneling") and wave-mechanical reflection. The problem consists of solving the one-dimensional time-independent Schrödinger equation for a particle encountering a rectangular potential energy barrier. It is usually assumed, as here, that a free particle impinges on the barrier from the left. Although classically a particle behaving as a point mass would be reflected if its energy is less than a particle actually behaving as a matter wave has a non-zero probability of penetrating the barrier and continuing its travel as a wave on the other side. In classical wave-physics, this effect is known as evanescent wave coupling. The likelihood that the particle will pass through the barrier is given by the transmission coefficient, whereas the likelihood that it is reflected is given by ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Quantum Tunnelling
Quantum tunnelling, also known as tunneling ( US) is a quantum mechanical phenomenon whereby a wavefunction can propagate through a potential barrier. The transmission through the barrier can be finite and depends exponentially on the barrier height and barrier width. The wavefunction may disappear on one side and reappear on the other side. The wavefunction and its first derivative are continuous. In steady-state, the probability flux in the forward direction is spatially uniform. No particle or wave is lost. Tunneling occurs with barriers of thickness around 1–3 nm and smaller. Some authors also identify the mere penetration of the wavefunction into the barrier, without transmission on the other side as a tunneling effect. Quantum tunneling is not predicted by the laws of classical mechanics where surmounting a potential barrier requires sufficient kinetic energy. Quantum tunneling plays an essential role in physical phenomena such as nuclear fusion and alpha radioact ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Semicircle Potential Well
In quantum mechanics, the case of a particle in a one-dimensional ring is similar to the particle in a box. The particle follows the path of a semicircle from 0 to \pi where it cannot escape, because the potential from \pi to 2 \pi is infinite. Instead there is total reflection, meaning the particle bounces back and forth between 0 to \pi . The Schrödinger equation for a free particle which is restricted to a semicircle (technically, whose configuration space is the circle S^1) is Wave function Using cylindrical coordinates on the 1-dimensional semicircle, the wave function depends only on the angular coordinate, and so Substituting the Laplacian in cylindrical coordinates, the wave function is therefore expressed as The moment of inertia for a semicircle, best expressed in cylindrical coordinates, is I \ \stackrel\ \iiint_V r^2 \,\rho(r,\phi,z)\,r dr\,d\phi\,dz \!. Solving the integral, one finds that the moment of inertia of a semicircle is I=m s^2 , exact ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Infinite Potential Well
In quantum mechanics, the particle in a box model (also known as the infinite potential well or the infinite square well) describes a particle free to move in a small space surrounded by impenetrable barriers. The model is mainly used as a hypothetical example to illustrate the differences between classical and quantum systems. In classical systems, for example, a particle trapped inside a large box can move at any speed within the box and it is no more likely to be found at one position than another. However, when the well becomes very narrow (on the scale of a few nanometers), quantum effects become important. The particle may only occupy certain positive energy levels. Likewise, it can never have zero energy, meaning that the particle can never "sit still". Additionally, it is more likely to be found at certain positions than at others, depending on its energy level. The particle may never be detected at certain positions, known as spatial nodes. The particle in a box mo ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Potential Well
A potential well is the region surrounding a local minimum of potential energy. Energy captured in a potential well is unable to convert to another type of energy (kinetic energy in the case of a gravitational potential well) because it is captured in the local minimum of a potential well. Therefore, a body may not proceed to the global minimum of potential energy, as it would naturally tend to do due to entropy. Overview Energy may be released from a potential well if sufficient energy is added to the system such that the local maximum is surmounted. In quantum physics, potential energy may escape a potential well without added energy due to the probabilistic characteristics of quantum particles; in these cases a particle may be imagined to tunnel ''through'' the walls of a potential well. The graph of a 2D potential energy function is a potential energy surface that can be imagined as the Earth's surface in a landscape of hills and valleys. Then a potential well would be a val ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Delta Function Potential
In quantum mechanics the delta potential is a potential well mathematically described by the Dirac delta function - a generalized function. Qualitatively, it corresponds to a potential which is zero everywhere, except at a single point, where it takes an infinite value. This can be used to simulate situations where a particle is free to move in two regions of space with a barrier between the two regions. For example, an electron can move almost freely in a conducting material, but if two conducting surfaces are put close together, the interface between them acts as a barrier for the electron that can be approximated by a delta potential. The delta potential well is a limiting case of the finite potential well, which is obtained if one maintains the product of the width of the well and the potential constant while decreasing the well's width and increasing the potential. This article, for simplicity, only considers a one-dimensional potential well, but analysis could be expanded ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Infinite Square Well
In quantum mechanics, the particle in a box model (also known as the infinite potential well or the infinite square well) describes a particle free to move in a small space surrounded by impenetrable barriers. The model is mainly used as a hypothetical example to illustrate the differences between classical physics, classical and quantum systems. In classical systems, for example, a particle trapped inside a large box can move at any speed within the box and it is no more likely to be found at one position than another. However, when the well becomes very narrow (on the scale of a few nanometers), quantum effects become important. The particle may only occupy certain positive energy levels. Likewise, it can never have zero energy, meaning that the particle can never "sit still". Additionally, it is more likely to be found at certain positions than at others, depending on its energy level. The particle may never be detected at certain positions, known as spatial nodes. The pa ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |