|
Farkas' Lemma
Farkas' lemma is a solvability theorem for a finite system of linear inequalities in mathematics. It was originally proven by the Hungarian mathematician Gyula Farkas (natural scientist), Gyula Farkas. Farkas' Lemma (mathematics), lemma is the key result underpinning the linear programming duality and has played a central role in the development of mathematical optimization (alternatively, mathematical programming). It is used amongst other things in the proof of the Karush–Kuhn–Tucker, Karush–Kuhn–Tucker theorem in nonlinear programming. Remarkably, in the area of the foundations of quantum theory, the lemma also underlies the complete set of Bell's theorem, Bell inequalities in the form of necessary and sufficient conditions for the existence of a Local hidden-variable theory, local hidden-variable theory, given data from any specific set of measurements. Generalizations of the Farkas' lemma are about the solvability theorem for convex inequalities, i.e., infinite syste ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Linear Inequalities
Linearity is the property of a mathematical relationship (''function (mathematics), function'') that can be graph of a function, graphically represented as a straight Line (geometry), line. Linearity is closely related to ''Proportionality (mathematics), proportionality''. Examples in physics include rectilinear motion, the linear relationship of voltage and Electric current, current in an electrical conductor (Ohm's law), and the relationship of mass and weight. By contrast, more complicated relationships are ''nonlinear''. Generalized for functions in more than one dimension (mathematics), dimension, linearity means the property of a function of being compatible with addition and scale analysis (mathematics), scaling, also known as the superposition principle. The word linear comes from Latin ''linearis'', "pertaining to or resembling a line". In mathematics In mathematics, a linear map or linear function ''f''(''x'') is a function that satisfies the two properties: * Addi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Fredholm Alternative
In mathematics, the Fredholm alternative, named after Ivar Fredholm, is one of Fredholm's theorems and is a result in Fredholm theory. It may be expressed in several ways, as a theorem of linear algebra, a theorem of integral equations, or as a theorem on Fredholm operators. Part of the result states that a non-zero complex number in the spectrum of a compact operator is an eigenvalue. Linear algebra If ''V'' is an ''n''-dimensional vector space and T:V\to V is a linear transformation, then exactly one of the following holds: #For each vector ''v'' in ''V'' there is a vector ''u'' in ''V'' so that T(u) = v. In other words: ''T'' is surjective (and so also bijective, since ''V'' is finite-dimensional). #\dim(\ker(T)) > 0. A more elementary formulation, in terms of matrices, is as follows. Given an ''m''×''n'' matrix ''A'' and a ''m''×1 column vector b, exactly one of the following must hold: #''Either:'' ''A'' x = b has a solution x #''Or:'' ''A''T y = 0 has a s ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Lemmas In Linear Algebra
Lemma may refer to: Language and linguistics * Lemma (morphology), the canonical, dictionary or citation form of a word * Lemma (psycholinguistics), a mental abstraction of a word about to be uttered Science and mathematics * Lemma (botany), a part of a grass plant * Lemma (mathematics), a type of proposition Other uses * ''Lemma'' (album), by John Zorn (2013) * Lemma (logic), an informal contention See also *Analemma, a diagram showing the variation of the position of the Sun in the sky *Dilemma *Lema (other) Lema may refer to: Nature Amphibians * Lema tree frog, ''Hypsiboas lemai'', a species of frog *''Centrolene lema'', a synonym for '' Vitreorana gorzulae'', the Bolivar giant glass frog Insects *'' Kedestes lema'', the Lema ranger, a butterfly * ... * Lemmatisation * Neurolemma, part of a neuron {{Disambiguation ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Semen Samsonovich Kutateladze
Semen, also known as seminal fluid, is an organic bodily fluid created to contain spermatozoon, spermatozoa. It is secreted by the gonads (sexual glands) and other sexual organs of male or hermaphrodite, hermaphroditic animals and can fertilization, fertilize the female ovum. Semen is produced and originates from the seminal vesicle, which is located in the pelvis. The process that results in the discharge of semen from the Ureteral orifice, urethral orifice is called ejaculation. In humans, seminal fluid contains several components besides spermatozoa: Proteolytic enzyme, proteolytic and other enzymes as well as fructose are elements of seminal fluid which promote the survival of spermatozoa, and provide a medium through which they can move or "swim". The fluid is designed to be discharged deep into the vagina, so the spermatozoa can pass into the uterus and form a zygote with an egg. Semen is also a form of genetic material. In animals, semen has been collected for cryoconse ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Fourier–Motzkin Elimination
Fourier–Motzkin elimination, also known as the FME method, is a mathematical algorithm for eliminating variables from a system of linear inequalities. It can output real solutions. The algorithm is named after Joseph Fourier who proposed the method in 1826 and Theodore Motzkin who re-discovered it in 1936. Elimination The elimination of a set of variables, say ''V'', from a system of relations (here linear inequalities) refers to the creation of another system of the same sort, but without the variables in ''V'', such that both systems have the same solutions over the remaining variables. If all variables are eliminated from a system of linear inequalities, then one obtains a system of constant inequalities. It is then trivial to decide whether the resulting system is true or false. It is true if and only if the original system has solutions. As a consequence, elimination of all variables can be used to detect whether a system of inequalities has solutions or not. Consider a s ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Minimax Theorem
In the mathematical area of game theory, a minimax theorem is a theorem providing conditions that guarantee that the max–min inequality is also an equality. The first theorem in this sense is von Neumann's minimax theorem from 1928, which was considered the starting point of game theory. Since then, several generalizations and alternative versions of von Neumann's original theorem have appeared in the literature. Zero-sum games The minimax theorem was first proven and published in 1928 by John von Neumann, who is quoted as saying "''As far as I can see, there could be no theory of games ... without that theorem ... I thought there was nothing worth publishing until the Minimax Theorem was proved''". Formally, von Neumann's minimax theorem states: Let X \subset \mathbb^n and Y \subset \mathbb^m be compact convex sets. If f: X \times Y \rightarrow \mathbb is a continuous function that is concave-convex, i.e. : f(\cdot,y): X \to \mathbb is concave for fixed y, and : f(x,\cdo ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Karush–Kuhn–Tucker Conditions
In mathematical optimization, the Karush–Kuhn–Tucker (KKT) conditions, also known as the Kuhn–Tucker conditions, are first derivative tests (sometimes called first-order necessary conditions) for a solution in nonlinear programming to be optimal, provided that some regularity conditions are satisfied. Allowing inequality constraints, the KKT approach to nonlinear programming generalizes the method of Lagrange multipliers, which allows only equality constraints. Similar to the Lagrange approach, the constrained maximization (minimization) problem is rewritten as a Lagrange function whose optimal point is a saddle point, i.e. a global maximum (minimum) over the domain of the choice variables and a global minimum (maximum) over the multipliers, which is why the Karush–Kuhn–Tucker theorem is sometimes referred to as the saddle-point theorem. The KKT conditions were originally named after Harold W. Kuhn and Albert W. Tucker, who first published the conditions in 1951. L ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Dual Linear Program
The dual of a given linear program (LP) is another LP that is derived from the original (the primal) LP in the following schematic way: * Each variable in the primal LP becomes a constraint in the dual LP; * Each constraint in the primal LP becomes a variable in the dual LP; * The objective direction is inversed – maximum in the primal becomes minimum in the dual and vice versa. The weak duality theorem states that the objective value of the dual LP at any feasible solution is always a bound on the objective of the primal LP at any feasible solution (upper or lower bound, depending on whether it is a maximization or minimization problem). In fact, this bounding property holds for the optimal values of the dual and primal LPs. The strong duality theorem states that, moreover, if the primal has an optimal solution then the dual has an optimal solution too, ''and the two optima are equal''. Pages 81–104. These theorems belong to a larger class of duality theorems in optimizatio ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Gordan's Theorem
Gordan's lemma is a lemma in convex geometry and algebraic geometry. It can be stated in several ways. * Let A be a matrix of integers. Let M be the set of non-negative integer solutions of A \cdot x = 0. Then there exists a finite subset of vectors in M, such that every element of M is a linear combination of these vectors with non-negative integer coefficients. * The semigroup of integral points in a rational convex polyhedral cone is finitely generated. * An affine toric variety is an algebraic variety (this follows from the fact that the prime spectrum of the semigroup algebra of such a semigroup is, by definition, an affine toric variety). The lemma is named after the mathematician Paul Gordan (1837–1912). Some authors have misspelled it as "Gordon's lemma". Proofs There are topological and algebraic proofs. Topological proof Let \sigma be the dual cone of the given rational polyhedral cone. Let u_1, \dots, u_r be integral vectors so that \sigma = \. Then the u_i's ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Slater's Condition
In mathematics, Slater's condition (or Slater condition) is a sufficient condition for strong duality to hold for a convex optimization problem, named after Morton L. Slater. Informally, Slater's condition states that the feasible region must have an interior point (see technical details below). Slater's condition is a specific example of a constraint qualification. In particular, if Slater's condition holds for the primal problem, then the duality gap is 0, and if the dual value is finite then it is attained. Formulation Consider the optimization problem : \text\; f_0(x) : \text\ :: f_i(x) \le 0 , i = 1,\ldots,m :: Ax = b where f_0,\ldots,f_m are convex functions. This is an instance of convex programming. In words, Slater's condition for convex programming states that strong duality holds if there exists an x^* such that x^* is strictly feasible (i.e. all constraints are satisfied and the nonlinear constraints are satisfied with strict inequalities). Mathematically, Sla ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
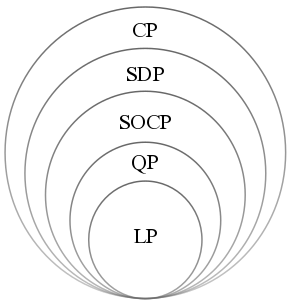
Convex Optimization
Convex optimization is a subfield of mathematical optimization that studies the problem of minimizing convex functions over convex sets (or, equivalently, maximizing concave functions over convex sets). Many classes of convex optimization problems admit polynomial-time algorithms, whereas mathematical optimization is in general NP-hard. Convex optimization has applications in a wide range of disciplines, such as automatic control systems, estimation and signal processing, communications and networks, electronic circuit design, data analysis and modeling, finance, statistics ( optimal experimental design), and structural optimization, where the approximation concept has proven to be efficient. With recent advancements in computing and optimization algorithms, convex programming is nearly as straightforward as linear programming. Definition A convex optimization problem is an optimization problem in which the objective function is a convex function and the feasible set is a c ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hyperplane Separation Theorem
In geometry, the hyperplane separation theorem is a theorem about disjoint convex sets in ''n''-dimensional Euclidean space. There are several rather similar versions. In one version of the theorem, if both these sets are closed and at least one of them is compact, then there is a hyperplane in between them and even two parallel hyperplanes in between them separated by a gap. In another version, if both disjoint convex sets are open, then there is a hyperplane in between them, but not necessarily any gap. An axis which is orthogonal to a separating hyperplane is a separating axis, because the orthogonal projections of the convex bodies onto the axis are disjoint. The hyperplane separation theorem is due to Hermann Minkowski. The Hahn–Banach separation theorem generalizes the result to topological vector spaces. A related result is the supporting hyperplane theorem. In the context of support-vector machines, the ''optimally separating hyperplane'' or ''maximum-margin hyp ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |