|
Ezra T. Newman
Ezra Theodore Newman (October 17, 1929 – March 24, 2021) was an American physicist, known for his many contributions to general relativity theory. He was Professor Emeritus at the University of Pittsburgh. Newman was awarded the 2011 Einstein Prize from the American Physical Society. Education Newman was born in the Bronx, New York City to David and Fannie (Slutsky) Newman.Allen G. Debus (1968) ''Who's Who in Science'', page 1250, A. N. Marquis He showed an early interest in science, pondering magnets, match flames, and science books. He was admitted to the Bronx High School of Science, where he excelled at physics. Ted's father hoped that he would follow him into dentistry, but instead Ted enrolled at New York University to further his study of physics, graduating with a B.A. in 1951. For graduate education he went to Syracuse University, obtaining an M.A. in 1955 and a Ph.D. the following year. Career in physics Newman joined the University of Pittsburgh faculty in 1956 ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Roger Penrose
Sir Roger Penrose (born 8 August 1931) is an English mathematician, mathematical physicist, philosopher of science and Nobel Laureate in Physics. He is Emeritus Rouse Ball Professor of Mathematics in the University of Oxford, an emeritus fellow of Wadham College, Oxford, and an honorary fellow of St John's College, Cambridge and University College London. Penrose has contributed to the mathematical physics of general relativity and cosmology. He has received several prizes and awards, including the 1988 Wolf Prize in Physics, which he shared with Stephen Hawking for the Penrose–Hawking singularity theorems, and one half of the 2020 Nobel Prize in Physics "for the discovery that black hole formation is a robust prediction of the general theory of relativity". He is regarded as one of the greatest living physicists, mathematicians and scientists, and is particularly noted for the breadth and depth of his work in both natural and formal sciences. Early life and education Bor ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
The Chronicle Of Higher Education
''The Chronicle of Higher Education'' is a newspaper and website that presents news, information, and jobs for college and university faculty and student affairs professionals (staff members and administrators). A subscription is required to read some articles. ''The Chronicle'', based in Washington, D.C., is a major news service in United States academic affairs. It is published every weekday online and appears weekly in print except for every other week in May, June, July, and August and the last three weeks in December. In print, ''The Chronicle'' is published in two sections: section A with news, section B with job listings, and ''The Chronicle Review,'' a magazine of arts and ideas. It also publishes ''The Chronicle of Philanthropy'', a newspaper for the nonprofit world; ''The Chronicle Guide to Grants'', an electronic database of corporate and foundation grants; and the web portal Arts & Letters Daily. History Corbin Gwaltney was the founder and had been the editor of t ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Gravitational Lens
A gravitational lens is a distribution of matter (such as a cluster of galaxies) between a distant light source and an observer that is capable of bending the light from the source as the light travels toward the observer. This effect is known as gravitational lensing, and the amount of bending is one of the predictions of Albert Einstein's general theory of relativity. Treating light as corpuscles travelling at the speed of light, Newtonian physics also predicts the bending of light, but only half of that predicted by general relativity. Although Einstein made unpublished calculations on the subject in 1912, Orest Khvolson (1924) and Frantisek Link (1936) are generally credited with being the first to discuss the effect in print. However, this effect is more commonly associated with Einstein, who published an article on the subject in 1936. Fritz Zwicky posited in 1937 that the effect could allow galaxy clusters to act as gravitational lenses. It was not until 1979 that this ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Journal Of Mathematical Physics
The ''Journal of Mathematical Physics'' is a peer-reviewed journal published monthly by the American Institute of Physics devoted to the publication of papers in mathematical physics. The journal was first published bimonthly beginning in January 1960; it became a monthly publication in 1963. The current editor is Jan Philip Solovej from University of Copenhagen The University of Copenhagen ( da, Københavns Universitet, KU) is a prestigious public university, public research university in Copenhagen, Copenhagen, Denmark. Founded in 1479, the University of Copenhagen is the second-oldest university in .... Its 2018 Impact Factor is 1.355 Abstracting and indexing This journal is indexed by the following services: 2013. References External links [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Complex Spacetime
In mathematics and mathematical physics, complex spacetime extends the traditional notion of spacetime described by real-valued space and time coordinates to complex-valued space and time coordinates. The notion is entirely mathematical with no physics implied, but should be seen as a tool, for instance, as exemplified by the Wick rotation. Real and complex spaces Mathematics The complexification of a real vector space results in a complex vector space (over the complex number field). To "complexify" a space means extending ordinary scalar multiplication of vectors by real numbers to scalar multiplication by complex numbers. For complexified inner product spaces, the complex inner product on vectors replaces the ordinary real-valued inner product, an example of the latter being the dot product. In mathematical physics, when we complexify a real coordinate space \mathbb^n we create a complex coordinate space \mathbb^n, referred to in differential geometry as a " complex manifol ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Complex Number
In mathematics, a complex number is an element of a number system that extends the real numbers with a specific element denoted , called the imaginary unit and satisfying the equation i^= -1; every complex number can be expressed in the form a + bi, where and are real numbers. Because no real number satisfies the above equation, was called an imaginary number by René Descartes. For the complex number a+bi, is called the , and is called the . The set of complex numbers is denoted by either of the symbols \mathbb C or . Despite the historical nomenclature "imaginary", complex numbers are regarded in the mathematical sciences as just as "real" as the real numbers and are fundamental in many aspects of the scientific description of the natural world. Complex numbers allow solutions to all polynomial equations, even those that have no solutions in real numbers. More precisely, the fundamental theorem of algebra asserts that every non-constant polynomial equation with real or ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Roy Kerr
Roy Patrick Kerr (; born 16 May 1934) is a New Zealand mathematician who discovered the Kerr metric, Kerr geometry, an exact solutions in general relativity, exact solution to the Einstein field equation of general relativity. His solution models the gravitational field outside an uncharged rotating massive object, including a rotating black hole.''Cracking the Einstein Code'' by Fulvio Melia, 2009 His solution to Einstein's equations predicted spinning black holes before they were discovered. Early life and education Kerr was born in 1934 in Kurow, New Zealand. He was born into a dysfunctional family, and his mother was forced to leave when he was three. When his father went to war, he was sent to a farm. After his father's return from war, they moved to ...[...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
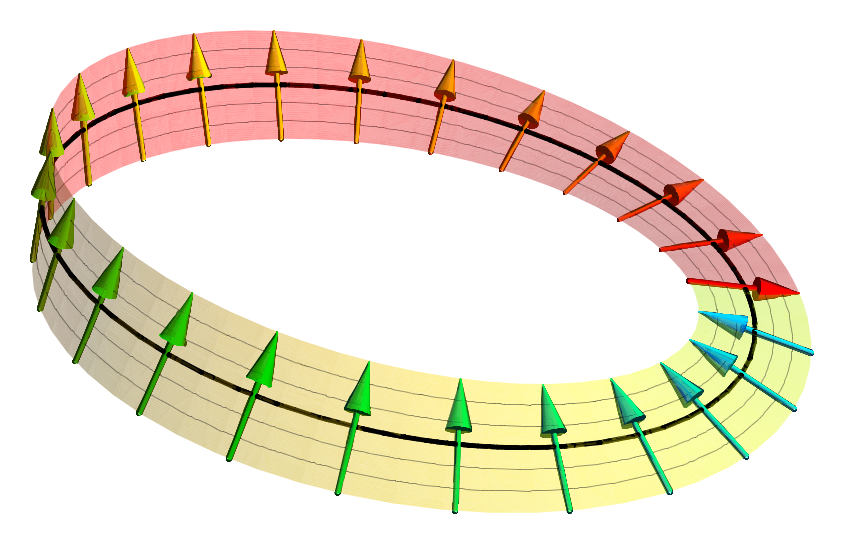
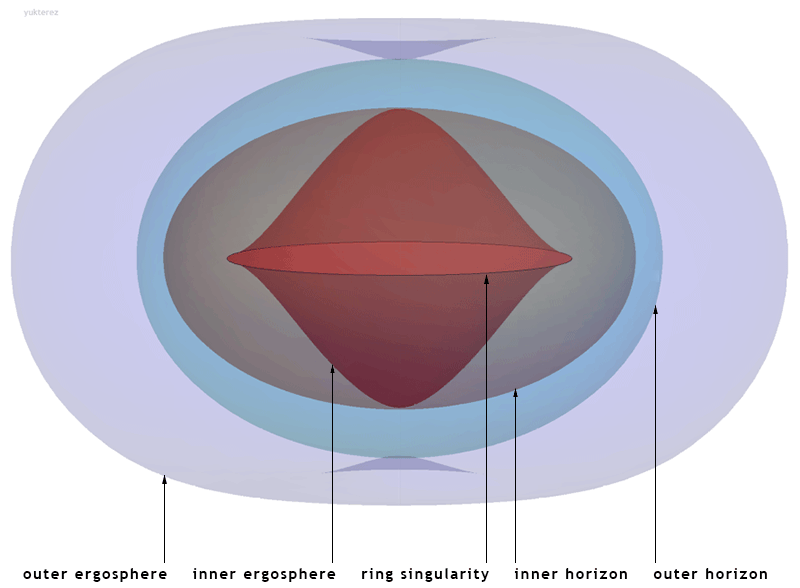
Kerr Metric
The Kerr metric or Kerr geometry describes the geometry of empty spacetime around a rotating uncharged axially symmetric black hole with a quasispherical event horizon. The Kerr metric is an exact solution of the Einstein field equations of general relativity; these equations are highly non-linear, which makes exact solutions very difficult to find. Overview The Kerr metric is a generalization to a rotating body of the Schwarzschild metric, discovered by Karl Schwarzschild in 1915, which described the geometry of spacetime around an uncharged, spherically symmetric, and non-rotating body. The corresponding solution for a ''charged'', spherical, non-rotating body, the Reissner–Nordström metric, was discovered soon afterwards (1916–1918). However, the exact solution for an uncharged, ''rotating'' black hole, the Kerr metric, remained unsolved until 1963, when it was discovered by Roy Kerr.Melia, Fulvio (2009). "Cracking the Einstein code: relativity and the birth of black ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Taub–NUT Space
The Taub–NUT metric (,McGraw-Hill ''Science & Technology Dictionary'': "Taub NUT space" ) is an exact solution to Einstein's equations. It may be considered a first attempt in finding the metric of a spinning black hole. It is sometimes also used in homogeneous but anisotropic cosmological models formulated in the framework of general relativity. The underlying Taub space was found by , and extended to a larger manifold by , whose initials form the "NUT" of "Taub–NUT". Taub's solution is an empty space solution of Einstein's equations with topology R×S3 and metric (or equivalently line element) :g =-dt^2/U(t) + 4l^2U(t)(d\psi+ \cos\theta d\phi)^2+(t^2+l^2)(d\theta^2+(\sin\theta)^2d\phi^2) where :U(t)=\frac and ''m'' and ''l'' are positive constants. Taub's metric has coordinate singularities at U=0, t=m+(m^2+l^2)^, and Newman, Tamburino and Unti showed how to extend the metric across these surfaces. When Roy Kerr developed the Kerr metric The Kerr metric or Kerr geom ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Einstein Field Equation
In the general theory of relativity, the Einstein field equations (EFE; also known as Einstein's equations) relate the geometry of spacetime to the distribution of matter within it. The equations were published by Einstein in 1915 in the form of a tensor equation which related the local ' (expressed by the Einstein tensor) with the local energy, momentum and stress within that spacetime (expressed by the stress–energy tensor). Analogously to the way that electromagnetic fields are related to the distribution of charges and currents via Maxwell's equations, the EFE relate the spacetime geometry to the distribution of mass–energy, momentum and stress, that is, they determine the metric tensor of spacetime for a given arrangement of stress–energy–momentum in the spacetime. The relationship between the metric tensor and the Einstein tensor allows the EFE to be written as a set of nonlinear partial differential equations when used in this way. The solutions of the EFE are ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Abraham H
Abraham, ; ar, , , name=, group= (originally Abram) is the common Hebrew patriarch of the Abrahamic religions, including Judaism, Christianity, and Islam. In Judaism, he is the founding father of the special relationship between the Jews and God; in Christianity, he is the spiritual progenitor of all believers, whether Jewish or non-Jewish; and in Islam, he is a link in the chain of Islamic prophets that begins with Adam (see Adam in Islam) and culminates in Muhammad. His life, told in the narrative of the Book of Genesis, revolves around the themes of posterity and land. Abraham is called by God to leave the house of his father Terah and settle in the land of Canaan, which God now promises to Abraham and his progeny. This promise is subsequently inherited by Isaac, Abraham's son by his wife Sarah, while Isaac's half-brother Ishmael is also promised that he will be the founder of a great nation. Abraham purchases a tomb (the Cave of the Patriarchs) at Hebron to be Sarah' ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Spinor
In geometry and physics, spinors are elements of a complex vector space that can be associated with Euclidean space. Like geometric vectors and more general tensors, spinors transform linearly when the Euclidean space is subjected to a slight (infinitesimal) rotation. Unlike vectors and tensors, a spinor transforms to its negative when the space is continuously rotated through a complete turn from 0° to 360° (see picture). This property characterizes spinors: spinors can be viewed as the "square roots" of vectors (although this is inaccurate and may be misleading; they are better viewed as "square roots" of sections of vector bundles – in the case of the exterior algebra bundle of the cotangent bundle, they thus become "square roots" of differential forms). It is also possible to associate a substantially similar notion of spinor to Minkowski space, in which case the Lorentz transformations of special relativity play the role of rotations. Spinors were introduced in geome ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
.svg "Click for more on -> Roger Penrose")
.jpg "Click for more on -> Abraham H")
