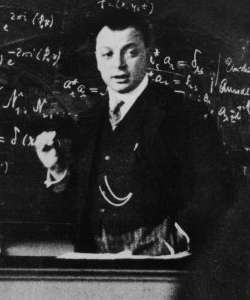|
Ernst Carl Gerlach Stueckelberg
Ernst Carl Gerlach Stueckelberg (baptised as Johann Melchior Ernst Karl Gerlach Stückelberg, full name after 1911: Baron Ernst Carl Gerlach Stueckelberg von Breidenbach zu Breidenstein und Melsbach; 1 February 1905 – 4 September 1984) was a Swiss mathematician and physicist, regarded as one of the most eminent physicists of the 20th century. Despite making key advances in theoretical physics, including the exchange particle model of fundamental forces, causal S-matrix theory, and the renormalization group, his idiosyncratic style and publication in minor journals led to his work not being widely recognized until the mid-1990s. Early life Born into a semi-aristocratic family in Basel in 1905, Stueckelberg's father was a lawyer, and his paternal grandfather a distinguished Swiss artist. A highly gifted school student, Stueckelberg initially began a physics degree at the University of Basel in 1923. Career While still a student, Stueckelberg was invited by the distinguished quan ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Stueckelberg Action
In field theory, the Stueckelberg action (named after Ernst Stueckelberg) describes a massive spin-1 field as an R (the real numbers are the Lie algebra of U(1)) Yang–Mills theory coupled to a real scalar field φ. This scalar field takes on values in a real 1D affine representation of R with'' m'' as the coupling strength. :\mathcal=-\frac(\partial^\mu A^\nu-\partial^\nu A^\mu)(\partial_\mu A_\nu-\partial_\nu A_\mu)+\frac(\partial^\mu \phi+m A^\mu)(\partial_\mu \phi+m A_\mu) This is a special case of the Higgs mechanism, where, in effect, and thus the mass of the Higgs scalar excitation has been taken to infinity, so the Higgs has decoupled and can be ignored, resulting in a nonlinear, affine representation of the field, instead of a linear representation — in contemporary terminology, a U(1) nonlinear -model. Gauge-fixing φ=0, yields the Proca action. This explains why, unlike the case for non-abelian vector fields, quantum electrodynamics with a massive pho ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
S-matrix
In physics, the ''S''-matrix or scattering matrix relates the initial state and the final state of a physical system undergoing a scattering process. It is used in quantum mechanics, scattering theory and quantum field theory (QFT). More formally, in the context of QFT, the ''S''-matrix is defined as the unitary matrix connecting sets of asymptotically free particle states (the ''in-states'' and the ''out-states'') in the Hilbert space of physical states. A multi-particle state is said to be ''free'' (non-interacting) if it transforms under Lorentz transformations as a tensor product, or ''direct product'' in physics parlance, of ''one-particle states'' as prescribed by equation below. ''Asymptotically free'' then means that the state has this appearance in either the distant past or the distant future. While the ''S''-matrix may be defined for any background (spacetime) that is asymptotically solvable and has no event horizons, it has a simple form in the case of the Minkow ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Lorentz-covariant
In relativistic mechanics, relativistic physics, Lorentz symmetry or Lorentz invariance, named after the Dutch physicist Hendrik Lorentz, is an equivalence of observation or observational symmetry due to special relativity implying that the laws of physics stay the same for all observers that are moving with respect to one another within an inertial frame. It has also been described as "the feature of nature that says experimental results are independent of the orientation or the boost velocity of the laboratory through space". Lorentz covariance, a related concept, is a property of the underlying spacetime manifold. Lorentz covariance has two distinct, but closely related meanings: # A physical quantity is said to be Lorentz covariant if it transforms under a given group representation, representation of the Lorentz group. According to the representation theory of the Lorentz group, these quantities are built out of scalar (physics), scalars, four-vectors, four-tensors, and spin ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Elementary Particles
In particle physics, an elementary particle or fundamental particle is a subatomic particle that is not composed of other particles. Particles currently thought to be elementary include electrons, the fundamental fermions (quarks, leptons, antiquarks, and antileptons, which generally are matter particles and antimatter particles), as well as the fundamental bosons ( gauge bosons and the Higgs boson), which generally are force particles that mediate interactions among fermions. A particle containing two or more elementary particles is a composite particle. Ordinary matter is composed of atoms, once presumed to be elementary particles – ''atomos'' meaning "unable to be cut" in Greek – although the atom's existence remained controversial until about 1905, as some leading physicists regarded molecules as mathematical illusions, and matter as ultimately composed of energy. Subatomic constituents of the atom were first identified in the early 1930s; the electron and the ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Gregor Wentzel
Gregor Wentzel (17 February 1898 – 12 August 1978) was a German physicist known for development of quantum mechanics. Wentzel, Hendrik Kramers, and Léon Brillouin developed the Wentzel–Kramers–Brillouin approximation in 1926. In his early years, he contributed to X-ray spectroscopy, but then broadened out to make contributions to quantum mechanics, quantum electrodynamics, and meson theory. Life and education Gregor Wentzel was born in Düsseldorf, Germany, as the first of four children of Joseph and Anna Wentzel. He married Anna "Anny" Pohlmann in 1929 and his only child, Donat Wentzel, was born in 1934. The family moved to the USA in 1948 until he and Anny returned to Ascona, Switzerland in 1970. Career Wentzel began his university education in mathematics and physics in 1916, at the University of Freiburg. During 1917 and 1918, he served in the armed forces during World War I. He then resumed his education at Freiburg until 1919, when he went to the University ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Wolfgang Pauli
Wolfgang Ernst Pauli (; ; 25 April 1900 – 15 December 1958) was an Austrian theoretical physicist and one of the pioneers of quantum physics. In 1945, after having been nominated by Albert Einstein, Pauli received the Nobel Prize in Physics for his "decisive contribution through his discovery of a new law of Nature, the exclusion principle or Pauli principle". The discovery involved spin theory, which is the basis of a theory of the structure of matter. Early years Pauli was born in Vienna to a chemist, Wolfgang Joseph Pauli (''né'' Wolf Pascheles, 1869–1955), and his wife, Bertha Camilla Schütz; his sister was Hertha Pauli, a writer and actress. Pauli's middle name was given in honor of his godfather, physicist Ernst Mach. Pauli's paternal grandparents were from prominent families of Prague; his great-grandfather was the publisher Wolf Pascheles. Pauli's mother, Bertha Schütz, was raised in her mother's Roman Catholic religion; Pauli was raised as a Roman Catholic, a ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
University Of Lausanne
The University of Lausanne (UNIL; french: links=no, Université de Lausanne) in Lausanne, Switzerland was founded in 1537 as a school of Protestant theology, before being made a university in 1890. The university is the second oldest in Switzerland, and one of the oldest universities in the world to be in continuous operation. As of fall 2017, about 15,000 students and 3,300 employees studied and worked at the university. Approximately 1,500 international students attend the university (120 nationalities), which has a wide curriculum including exchange programs with other universities. Since 2005, the university follows the requirements of the Bologna process. The 2011 Times Higher Education World University Rankings ranked the University of Lausanne 116th globally. The CWTS Leiden Ranking 2015 ranks the University of Lausanne 11th in Europe and 41st globally, out of 750 universities. Together with the École polytechnique fédérale de Lausanne (EPFL) the university forms ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
University Of Geneva
The University of Geneva (French: ''Université de Genève'') is a public research university located in Geneva, Switzerland. It was founded in 1559 by John Calvin as a theological seminary. It remained focused on theology until the 17th century, when it became a center for enlightenment scholarship. Today, it is the third largest university in Switzerland by number of students. In 1873, it dropped its religious affiliations and became officially secular. In 2009, the University of Geneva celebrated the 450th anniversary of its founding. Almost 40% of the students come from foreign countries. The university holds and actively pursues teaching, research, and community service as its primary objectives. In 2016, it was ranked 53rd worldwide by the Shanghai Academic Ranking of World Universities, 89th by the QS World University Rankings, and 131st in the Times Higher Education World University Ranking. UNIGE is a member of the League of European Research Universities (includi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
University Of Zurich
The University of Zürich (UZH, german: Universität Zürich) is a public research university located in the city of Zürich, Switzerland. It is the largest university in Switzerland, with its 28,000 enrolled students. It was founded in 1833 from the existing colleges of theology, law, medicine which go back to 1525, and a new faculty of philosophy. Currently, the university has seven faculties: Philosophy, Human Medicine, Economic Sciences, Law, Mathematics and Natural Sciences, Theology and Veterinary Medicine. The university offers the widest range of subjects and courses of any Swiss higher education institution. History The University of Zurich was founded on April 29, 1833, when the existing colleges of theology, the ''Carolinum'' founded by Huldrych Zwingli in 1525, law and medicine were merged with a new faculty of Philosophy. It was the first university in Europe to be founded by the state rather than a monarch or church. In the university's early years, ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
American Physical Society
The American Physical Society (APS) is a not-for-profit membership organization of professionals in physics and related disciplines, comprising nearly fifty divisions, sections, and other units. Its mission is the advancement and diffusion of knowledge of physics. The society publishes more than a dozen scientific journals, including the prestigious ''Physical Review'' and '' Physical Review Letters'', and organizes more than twenty science meetings each year. APS is a member society of the American Institute of Physics. Since January 2021 the organization has been led by chief executive officer Jonathan Bagger. History The American Physical Society was founded on May 20, 1899, when thirty-six physicists gathered at Columbia University for that purpose. They proclaimed the mission of the new Society to be "to advance and diffuse the knowledge of physics", and in one way or another the APS has been at that task ever since. In the early years, virtually the sole activity of th ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Princeton University
Princeton University is a private research university in Princeton, New Jersey. Founded in 1746 in Elizabeth as the College of New Jersey, Princeton is the fourth-oldest institution of higher education in the United States and one of the nine colonial colleges chartered before the American Revolution. It is one of the highest-ranked universities in the world. The institution moved to Newark in 1747, and then to the current site nine years later. It officially became a university in 1896 and was subsequently renamed Princeton University. It is a member of the Ivy League. The university is governed by the Trustees of Princeton University and has an endowment of $37.7 billion, the largest endowment per student in the United States. Princeton provides undergraduate and graduate instruction in the humanities, social sciences, natural sciences, and engineering to approximately 8,500 students on its main campus. It offers postgraduate degrees through the Princeton Schoo ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Cathode
A cathode is the electrode from which a conventional current leaves a polarized electrical device. This definition can be recalled by using the mnemonic ''CCD'' for ''Cathode Current Departs''. A conventional current describes the direction in which positive charges move. Electrons have a negative electrical charge, so the movement of electrons is opposite to that of the conventional current flow. Consequently, the mnemonic ''cathode current departs'' also means that electrons flow ''into'' the device's cathode from the external circuit. For example, the end of a household battery marked with a + (plus) is the cathode. The electrode through which conventional current flows the other way, into the device, is termed an anode. Charge flow Conventional current flows from cathode to anode outside the cell or device (with electrons moving in the opposite direction), regardless of the cell or device type and operating mode. Cathode polarity with respect to the anode can be posi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |