|
Ellipsoidal Coordinates
Ellipsoidal coordinates are a three-dimensional orthogonal coordinate system (\lambda, \mu, \nu) that generalizes the two-dimensional elliptic coordinate system. Unlike most three-dimensional orthogonal coordinate systems that feature quadratic coordinate surfaces, the ellipsoidal coordinate system is based on confocal quadrics. Basic formulae The Cartesian coordinates (x, y, z) can be produced from the ellipsoidal coordinates ( \lambda, \mu, \nu ) by the equations : x^ = \frac : y^ = \frac : z^ = \frac where the following limits apply to the coordinates : - \lambda < c^ < - \mu < b^ < -\nu < a^. Consequently, surfaces of constant are s : whereas surfaces of constant are |
Orthogonal Coordinates
In mathematics, orthogonal coordinates are defined as a set of ''d'' coordinates q = (''q''1, ''q''2, ..., ''q''''d'') in which the coordinate hypersurfaces all meet at right angles (note: superscripts are indices, not exponents). A coordinate surface for a particular coordinate ''q''''k'' is the curve, surface, or hypersurface on which ''q''''k'' is a constant. For example, the three-dimensional Cartesian coordinates (''x'', ''y'', ''z'') is an orthogonal coordinate system, since its coordinate surfaces ''x'' = constant, ''y'' = constant, and ''z'' = constant are planes that meet at right angles to one another, i.e., are perpendicular. Orthogonal coordinates are a special but extremely common case of curvilinear coordinates. Motivation While vector operations and physical laws are normally easiest to derive in Cartesian coordinates, non-Cartesian orthogonal coordinates are often used instead for the solution of various problems, especially boundary value problems, such as tho ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Laplacian
In mathematics, the Laplace operator or Laplacian is a differential operator given by the divergence of the gradient of a scalar function on Euclidean space. It is usually denoted by the symbols \nabla\cdot\nabla, \nabla^2 (where \nabla is the nabla operator), or \Delta. In a Cartesian coordinate system, the Laplacian is given by the sum of second partial derivatives of the function with respect to each independent variable. In other coordinate systems, such as cylindrical and spherical coordinates, the Laplacian also has a useful form. Informally, the Laplacian of a function at a point measures by how much the average value of over small spheres or balls centered at deviates from . The Laplace operator is named after the French mathematician Pierre-Simon de Laplace (1749–1827), who first applied the operator to the study of celestial mechanics: the Laplacian of the gravitational potential due to a given mass density distribution is a constant multiple of that ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Course Of Theoretical Physics
The ''Course of Theoretical Physics'' is a ten-volume series of books covering theoretical physics that was initiated by Lev Landau and written in collaboration with his student Evgeny Lifshitz starting in the late 1930s. It is said that Landau composed much of the series in his head while in an NKVD prison in 1938–1939. However, almost all of the actual writing of the early volumes was done by Lifshitz, giving rise to the witticism, "not a word of Landau and not a thought of Lifshitz". The first eight volumes were finished in the 1950s, written in Russian and translated into English in the late 1950s by John Stewart Bell, together with John Bradbury Sykes, M. J. Kearsley, and W. H. Reid. The last two volumes were written in the early 1980s. Vladimir Berestetskii and Lev Pitaevskii also contributed to the series. The series is often referred to as "Landau and Lifshitz", "Landafshitz" (Russian: "Ландафшиц"), or "Lanlifshitz" (Russian: "Ланлифшиц") in in ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Theresa M
Teresa (also Theresa, Therese; french: Thérèse) is a feminine given name. It originates in the Iberian Peninsula in late antiquity. Its derivation is uncertain, it may be derived from Greek θερίζω (''therízō'') "to harvest or reap", or from θέρος (''theros'') "summer". It is first recorded in the form ''Therasia'', the name of Therasia of Nola, an aristocrat of the 4th century. Its popularity outside of Iberia increased because of saint Teresa of Ávila, and more recently Thérèse of Lisieux and Mother Teresa. In the United States it was ranked as the 852nd most popular name for girls born in 2008, down from 226th in 1992 (it ranked 65th in 1950, and 102nd in 1900). Spelled "Teresa," it was the 580th most popular name for girls born in 2008, down from 206th in 1992 (it ranked 81st in 1950, and 220th in 1900). People In aristocracy: *Teresa of Portugal (other) ** Theresa, Countess of Portugal (1080–1130), mother of Afonso Henriques, the first K ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Map Projection Of The Triaxial Ellipsoid
In geodesy, a map projection of the triaxial ellipsoid maps Earth or some other astronomical body modeled as a triaxial ellipsoid to the plane. Such a model is called the reference ellipsoid. In most cases, reference ellipsoids are spheroids, and sometimes spheres. Massive objects have sufficient gravity to overcome their own rigidity and usually have an oblate ellipsoid shape. However, minor moons or small solar system bodies are not under hydrostatic equilibrium. Usually such bodies have irregular shapes. Furthermore, some of gravitationally rounded objects may have a tri-axial ellipsoid shape due to rapid rotation (such as Haumea) or unidirectional strong tidal forces (such as Io). Examples A triaxial equivalent of the Mercator projection was developed by John P. Snyder. Equidistant map projections of a triaxial ellipsoid were developed by Paweł Pędzich. Conic Projections of a triaxial ellipsoid were developed by Maxim Nyrtsov. Equal-area cylindrical and azimuthal projec ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Focaloid
In geometry, a focaloid is a shell bounded by two concentric, confocal ellipses (in 2D) or ellipsoids (in 3D). When the thickness of the shell becomes negligible, it is called a thin focaloid. Mathematical definition (3D) If one boundary surface is given by : \frac+\frac+\frac=1 with semiaxes ''a'', ''b'', ''c'' the second surface is given by : \frac+\frac+\frac=1. The thin focaloid is then given by the limit \lambda \to 0. In general, a focaloid could be understood as a shell consisting out of two closed coordinate surfaces of a confocal ellipsoidal coordinate system. Confocal Confocal ellipsoids share the same foci, which are given for the example above by : f_1^2=a^2-b^2=(a^2+\lambda)-(b^2+\lambda), \, : f_2^2=a^2-c^2=(a^2+\lambda)-(c^2+\lambda), \, : f_3^2=b^2-c^2=(b^2+\lambda)-(c^2+\lambda). Physical significance A focaloid can be used as a construction element of a matter or charge distribution. The particular importance of focaloids ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Ellipsoidal Latitude
The study of geodesics on an ellipsoid arose in connection with geodesy specifically with the solution of triangulation networks. The figure of the Earth is well approximated by an '' oblate ellipsoid'', a slightly flattened sphere. A ''geodesic'' is the shortest path between two points on a curved surface, analogous to a straight line on a plane surface. The solution of a triangulation network on an ellipsoid is therefore a set of exercises in spheroidal trigonometry . If the Earth is treated as a sphere, the geodesics are great circles (all of which are closed) and the problems reduce to ones in spherical trigonometry. However, showed that the effect of the rotation of the Earth results in its resembling a slightly oblate ellipsoid: in this case, the equator and the meridians are the only simple closed geodesics. Furthermore, the shortest path between two points on the equator does not necessarily run along the equator. Finally, if the ellipsoid is further perturbed to ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Spherical Coordinates
In mathematics, a spherical coordinate system is a coordinate system for three-dimensional space where the position of a point is specified by three numbers: the ''radial distance'' of that point from a fixed origin, its ''polar angle'' measured from a fixed zenith direction, and the '' azimuthal angle'' of its orthogonal projection on a reference plane that passes through the origin and is orthogonal to the zenith, measured from a fixed reference direction on that plane. It can be seen as the three-dimensional version of the polar coordinate system. The radial distance is also called the ''radius'' or ''radial coordinate''. The polar angle may be called '' colatitude'', '' zenith angle'', '' normal angle'', or '' inclination angle''. When radius is fixed, the two angular coordinates make a coordinate system on the sphere sometimes called spherical polar coordinates. The use of symbols and the order of the coordinates differs among sources and disciplines. This article wi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Curvilinear Coordinates
In geometry, curvilinear coordinates are a coordinate system for Euclidean space in which the coordinate lines may be curved. These coordinates may be derived from a set of Cartesian coordinates by using a transformation that is locally invertible (a one-to-one map) at each point. This means that one can convert a point given in a Cartesian coordinate system to its curvilinear coordinates and back. The name ''curvilinear coordinates'', coined by the French mathematician Lamé, derives from the fact that the coordinate surfaces of the curvilinear systems are curved. Well-known examples of curvilinear coordinate systems in three-dimensional Euclidean space (R3) are cylindrical and spherical coordinates. A Cartesian coordinate surface in this space is a coordinate plane; for example ''z'' = 0 defines the ''x''-''y'' plane. In the same space, the coordinate surface ''r'' = 1 in spherical coordinates is the surface of a unit sphere, which is curved. The formalism of curvil ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Coordinate System
In geometry, a coordinate system is a system that uses one or more numbers, or coordinates, to uniquely determine the position of the points or other geometric elements on a manifold such as Euclidean space. The order of the coordinates is significant, and they are sometimes identified by their position in an ordered tuple and sometimes by a letter, as in "the ''x''-coordinate". The coordinates are taken to be real numbers in elementary mathematics, but may be complex numbers or elements of a more abstract system such as a commutative ring. The use of a coordinate system allows problems in geometry to be translated into problems about numbers and ''vice versa''; this is the basis of analytic geometry. Common coordinate systems Number line The simplest example of a coordinate system is the identification of points on a line with real numbers using the '' number line''. In this system, an arbitrary point ''O'' (the ''origin'') is chosen on a given line. The coordinate of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
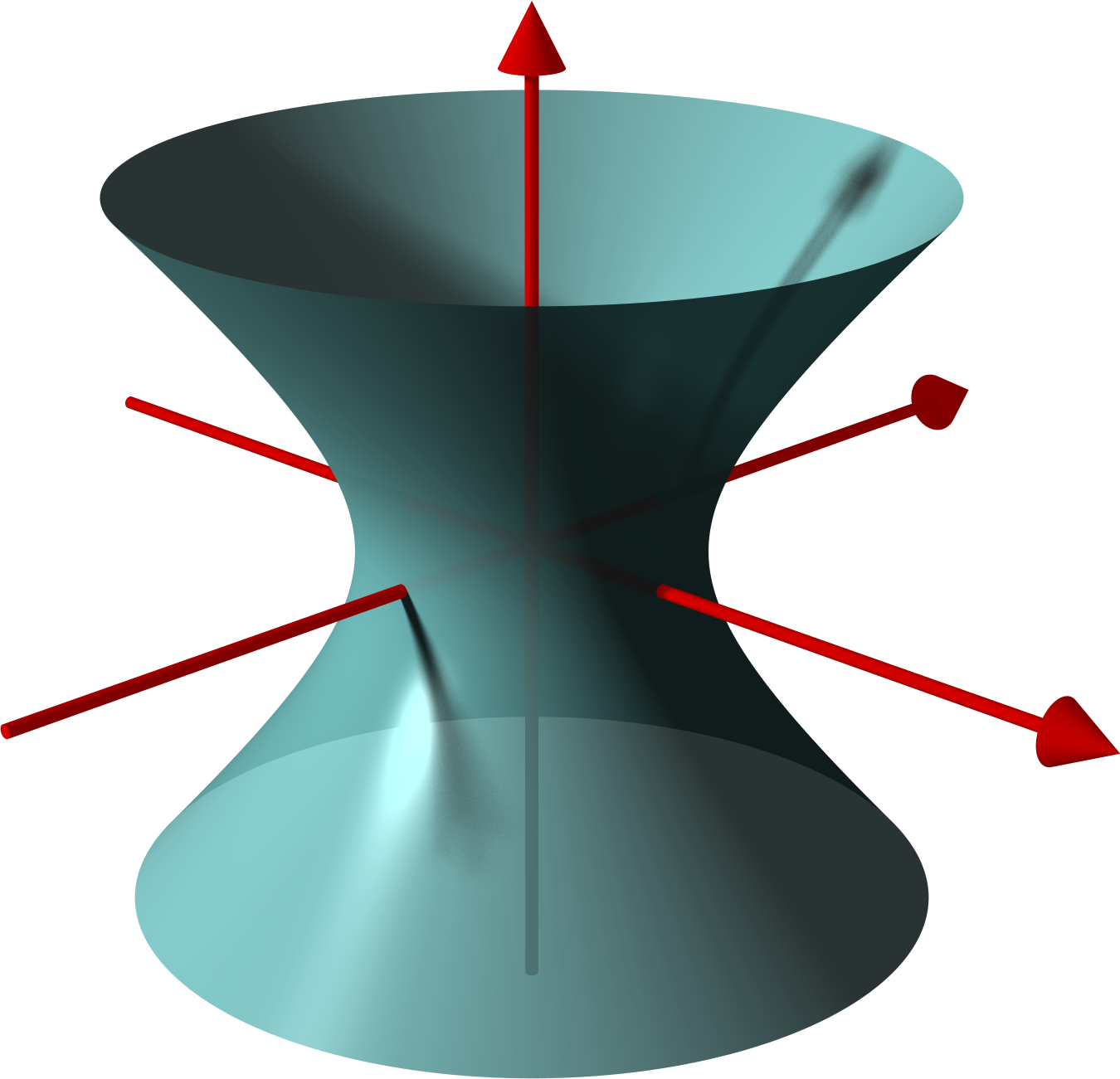
Hyperboloid
In geometry, a hyperboloid of revolution, sometimes called a circular hyperboloid, is the surface generated by rotating a hyperbola around one of its principal axes. A hyperboloid is the surface obtained from a hyperboloid of revolution by deforming it by means of directional scalings, or more generally, of an affine transformation. A hyperboloid is a quadric surface, that is, a surface defined as the zero set of a polynomial of degree two in three variables. Among quadric surfaces, a hyperboloid is characterized by not being a cone or a cylinder, having a center of symmetry, and intersecting many planes into hyperbolas. A hyperboloid has three pairwise perpendicular axes of symmetry, and three pairwise perpendicular planes of symmetry. Given a hyperboloid, one can choose a Cartesian coordinate system such that the hyperboloid is defined by one of the following equations: : + - = 1, or : + - = -1. The coordinate axes are axes of symmetry of the hyperboloid and th ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Ellipsoid
An ellipsoid is a surface that may be obtained from a sphere by deforming it by means of directional scalings, or more generally, of an affine transformation. An ellipsoid is a quadric surface; that is, a surface that may be defined as the zero set of a polynomial of degree two in three variables. Among quadric surfaces, an ellipsoid is characterized by either of the two following properties. Every planar cross section is either an ellipse, or is empty, or is reduced to a single point (this explains the name, meaning "ellipse-like"). It is bounded, which means that it may be enclosed in a sufficiently large sphere. An ellipsoid has three pairwise perpendicular axes of symmetry which intersect at a center of symmetry, called the center of the ellipsoid. The line segments that are delimited on the axes of symmetry by the ellipsoid are called the ''principal axes'', or simply axes of the ellipsoid. If the three axes have different lengths, the figure is a triaxial ellipsoi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |