|
Dynamic Scaling
Dynamic scaling (sometimes known as Family-Vicsek scaling) is a litmus test that shows whether an evolving system exhibits self-similarity. In general a function is said to exhibit dynamic scaling if it satisfies: :f(x,t)\sim t^\theta \varphi \left( \frac x \right). Here the exponent \theta is fixed by the dimensional requirement [f]=[t^\theta]. The numerical value of f/t^\theta should remain invariant despite the unit of measurement of t is changed by some factor since \varphi is a dimensionless quantity. Many of these systems evolve in a self-similar fashion in the sense that data obtained from the snapshot at any fixed time is similar to the respective data taken from the snapshot of any earlier or later time. That is, the system is similar to itself at different times. The litmus test of such self-similarity is provided by the dynamic scaling. History The term "dynamic scaling" as one of the essential concepts to describe the dynamics of critical phenomena seems to origina ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Self-similarity
__NOTOC__ In mathematics, a self-similar object is exactly or approximately similar to a part of itself (i.e., the whole has the same shape as one or more of the parts). Many objects in the real world, such as coastlines, are statistically self-similar: parts of them show the same statistical properties at many scales. Self-similarity is a typical property of fractals. Scale invariance is an exact form of self-similarity where at any magnification there is a smaller piece of the object that is similar to the whole. For instance, a side of the Koch snowflake is both symmetrical and scale-invariant; it can be continually magnified 3x without changing shape. The non-trivial similarity evident in fractals is distinguished by their fine structure, or detail on arbitrarily small scales. As a counterexample, whereas any portion of a straight line may resemble the whole, further detail is not revealed. A time developing phenomenon is said to exhibit self-similarity if the numerical v ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Internet
The Internet (or internet) is the global system of interconnected computer networks that uses the Internet protocol suite (TCP/IP) to communicate between networks and devices. It is a '' network of networks'' that consists of private, public, academic, business, and government networks of local to global scope, linked by a broad array of electronic, wireless, and optical networking technologies. The Internet carries a vast range of information resources and services, such as the inter-linked hypertext documents and applications of the World Wide Web (WWW), electronic mail, telephony, and file sharing. The origins of the Internet date back to the development of packet switching and research commissioned by the United States Department of Defense in the 1960s to enable time-sharing of computers. The primary precursor network, the ARPANET, initially served as a backbone for interconnection of regional academic and military networks in the 1970s to enable resource shari ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Weighted Planar Stochastic Lattice (WPSL)
Physicists often use various lattices to apply their favorite models in them. For instance, the most favorite lattice is perhaps the square lattice. There are 14 Bravais space lattice where every cell has exactly the same number of nearest, next nearest, nearest of next nearest etc. neighbors and hence they are called regular lattice. Often physicists and mathematicians study phenomena which require disordered lattice where each cell do not have exactly the same number of neighbors rather the number of neighbors can vary wildly. For instance, if one wants to study the spread of disease, viruses, rumors etc. then the last thing one would look for is the square lattice. In such cases a disordered lattice is necessary. One way of constructing a disordered lattice is by doing the following. Starting with a square, say of unit area, and dividing randomly at each step only one block, after picking it preferentially with respect to ares, into four smaller blocks creates weighted planar s ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Surface Growth
In mathematics and physics, surface growth refers to models used in the dynamical study of the growth of a surface, usually by means of a stochastic differential equation of a field. Examples Popular growth models include: * KPZ equation * Dimer model * Eden growth model * SOS model *Self-avoiding walk *Abelian sandpile model *Kuramoto–Sivashinsky equation (or the flame equation, for studying the surface of a flame front) They are studied for their fractal properties, scaling behavior, critical exponents, universality classes, and relations to chaos theory, dynamical system, non-equilibrium / disordered / complex systems. Popular tools include statistical mechanics, renormalization group, rough path theory, etc. Kinetic Monte Carlo surface growth model Kinetic Monte Carlo (KMC) is a form of computer simulation in which atoms and molecules are allowed to interact at given rate that could be controlled based on known physics. This simulation method is typically used in the ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Cantor Set
In mathematics, the Cantor set is a set of points lying on a single line segment that has a number of unintuitive properties. It was discovered in 1874 by Henry John Stephen Smith and introduced by German mathematician Georg Cantor in 1883. Through consideration of this set, Cantor and others helped lay the foundations of modern point-set topology. The most common construction is the Cantor ternary set, built by removing the middle third of a line segment and then repeating the process with the remaining shorter segments. Cantor mentioned the ternary construction only in passing, as an example of a more general idea, that of a perfect set that is nowhere dense. More generally, in topology, ''a'' Cantor space is a topological space homeomorphic to the Cantor ternary set (equipped with its subspace topology). By a theorem of Brouwer, this is equivalent to being perfect nonempty, compact metrizable and zero dimensional. Construction and formula of the ternary set The Cantor tern ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Complex Network
In the context of network theory, a complex network is a graph (network) with non-trivial topological features—features that do not occur in simple networks such as lattices or random graphs but often occur in networks representing real systems. The study of complex networks is a young and active area of scientific research (since 2000) inspired largely by empirical findings of real-world networks such as computer networks, biological networks, technological networks, brain networks, climate networks and social networks. Definition Most social, biological, and technological networks display substantial non-trivial topological features, with patterns of connection between their elements that are neither purely regular nor purely random. Such features include a heavy tail in the degree distribution, a high clustering coefficient, assortativity or disassortativity among vertices, community structure, and hierarchical structure. In the case of directed networks these feat ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Smoluchowski Coagulation Equation
In statistical physics, the Smoluchowski coagulation equation is a population balance equation introduced by Marian Smoluchowski in a seminal 1916 publication, describing the time evolution of the number density of particles as they coagulate (in this context "clumping together") to size ''x'' at time ''t''. Simultaneous coagulation (or aggregation) is encountered in processes involving polymerization, coalescence of aerosols, emulsication, flocculation. Equation The distribution of particle size changes in time according to the interrelation of all particles of the system. Therefore, the Smoluchowski coagulation equation is an integrodifferential equation of the particle-size distribution. In the case when the sizes of the coagulated particles are continuous variables, the equation involves an integral: : \frac=\frac\int^x_0K(x-y,y)n(x-y,t)n(y,t)\,dy - \int^\infty_0K(x,y)n(x,t)n(y,t)\,dy. If ''dy'' is interpreted as a discrete measure, i.e. when particles join in discrete s ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
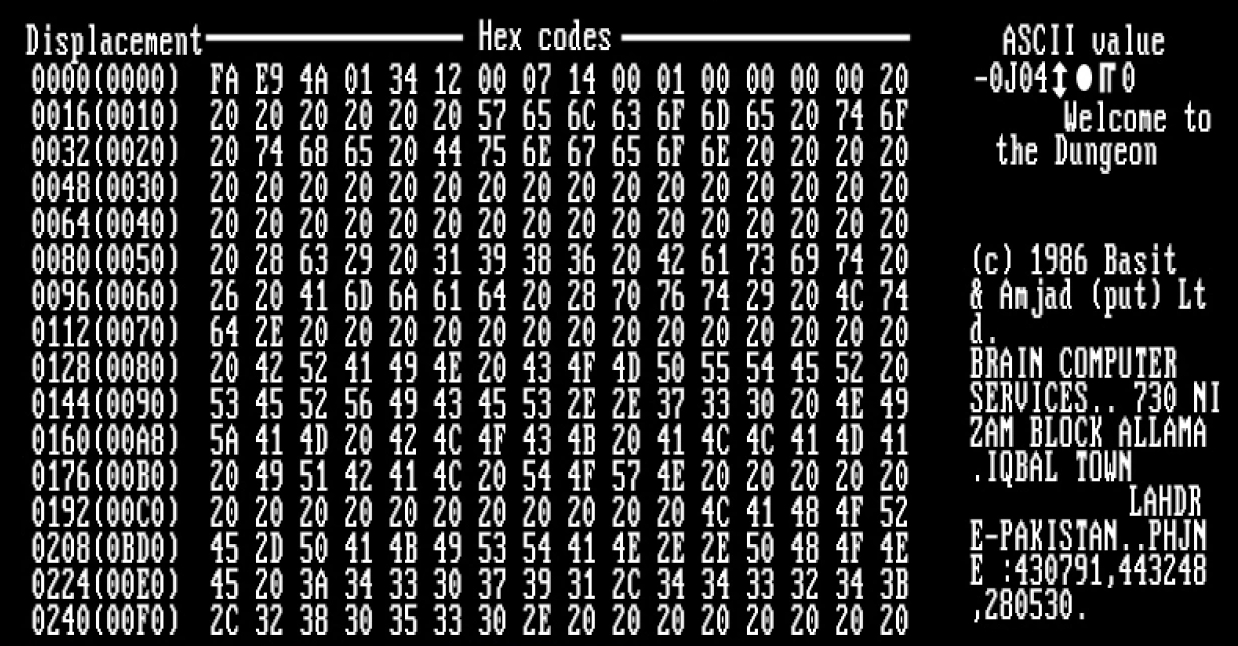
Computer Virus
A computer virus is a type of computer program that, when executed, replicates itself by modifying other computer programs and inserting its own code. If this replication succeeds, the affected areas are then said to be "infected" with a computer virus, a metaphor derived from biological viruses. Computer viruses generally require a host program. The virus writes its own code into the host program. When the program runs, the written virus program is executed first, causing infection and damage. A computer worm does not need a host program, as it is an independent program or code chunk. Therefore, it is not restricted by the host program, but can run independently and actively carry out attacks. Virus writers use social engineering deceptions and exploit detailed knowledge of security vulnerabilities to initially infect systems and to spread the virus. Viruses use complex anti-detection/stealth strategies to evade antivirus software. Motives for creating viruses can inclu ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Polymer Degradation
Polymer degradation is the reduction in the physical properties of a polymer, such as strength, caused by changes in its chemical composition. Polymers and particularly plastics are subject to degradation at all stages of their product life cycle, including during their initial processing, use, disposal into the environment and recycling. The rate of this degradation varies significantly; biodegradation can take decades, whereas some industrial processes can completely decompose a polymer in hours. Technologies have been developed to both inhibit or promote degradation. For instance, polymer stabilizers ensure plastic items are produced with the desired properties, extend their useful lifespans, and facilitate their recycling. Conversely, biodegradable additives accelerate the degradation of plastic waste by improving its biodegradability. Some forms of plastic recycling can involve the complete degradation of a polymer back into monomers or other chemicals. In general, the effec ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Network Science
Network science is an academic field which studies complex networks such as telecommunication networks, computer networks, biological networks, cognitive and semantic networks, and social networks, considering distinct elements or actors represented by ''nodes'' (or ''vertices'') and the connections between the elements or actors as ''links'' (or ''edges''). The field draws on theories and methods including graph theory from mathematics, statistical mechanics from physics, data mining and information visualization from computer science, inferential modeling from statistics, and social structure from sociology. The United States National Research Council defines network science as "the study of network representations of physical, biological, and social phenomena leading to predictive models of these phenomena." Background and history The study of networks has emerged in diverse disciplines as a means of analyzing complex relational data. The earliest known paper in this f ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |