|
Conditional Probability
In probability theory, conditional probability is a measure of the probability of an event occurring, given that another event (by assumption, presumption, assertion or evidence) has already occurred. This particular method relies on event B occurring with some sort of relationship with another event A. In this event, the event B can be analyzed by a conditional probability with respect to A. If the event of interest is and the event is known or assumed to have occurred, "the conditional probability of given ", or "the probability of under the condition ", is usually written as or occasionally . This can also be understood as the fraction of probability B that intersects with A: P(A \mid B) = \frac. For example, the probability that any given person has a cough on any given day may be only 5%. But if we know or assume that the person is sick, then they are much more likely to be coughing. For example, the conditional probability that someone unwell (sick) is coughing might be ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Conditional Expectation
In probability theory, the conditional expectation, conditional expected value, or conditional mean of a random variable is its expected value – the value it would take “on average” over an arbitrarily large number of occurrences – given that a certain set of "conditions" is known to occur. If the random variable can take on only a finite number of values, the “conditions” are that the variable can only take on a subset of those values. More formally, in the case when the random variable is defined over a discrete probability space, the "conditions" are a partition of this probability space. Depending on the context, the conditional expectation can be either a random variable or a function. The random variable is denoted E(X\mid Y) analogously to conditional probability. The function form is either denoted E(X\mid Y=y) or a separate function symbol such as f(y) is introduced with the meaning E(X\mid Y) = f(Y). Examples Example 1: Dice rolling Consider the roll of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Probability Theory
Probability theory is the branch of mathematics concerned with probability. Although there are several different probability interpretations, probability theory treats the concept in a rigorous mathematical manner by expressing it through a set of axioms. Typically these axioms formalise probability in terms of a probability space, which assigns a measure taking values between 0 and 1, termed the probability measure, to a set of outcomes called the sample space. Any specified subset of the sample space is called an event. Central subjects in probability theory include discrete and continuous random variables, probability distributions, and stochastic processes (which provide mathematical abstractions of non-deterministic or uncertain processes or measured quantities that may either be single occurrences or evolve over time in a random fashion). Although it is not possible to perfectly predict random events, much can be said about their behavior. Two major results in probability ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Bruno De Finetti
Bruno de Finetti (13 June 1906 – 20 July 1985) was an Italian probabilist statistician and actuary, noted for the "operational subjective" conception of probability. The classic exposition of his distinctive theory is the 1937 "La prévision: ses lois logiques, ses sources subjectives," which discussed probability founded on the coherence of betting odds and the consequences of exchangeability. Life De Finetti was born in Innsbruck, Austria, and studied mathematics at Politecnico di Milano. He graduated in 1927 writing his thesis under the supervision of Giulio Vivanti. After graduation, he worked as an actuary and a statistician at ''Istituto Nazionale di Statistica'' ( National Institute of Statistics) in Rome and, from 1931, the Trieste insurance company Assicurazioni Generali. In 1936 he won a competition for Chair of Financial Mathematics and Statistics, but was not nominated due to a fascist law barring access to unmarried candidates; he was appointed as ordinary profess ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Radical Probabilism
Radical probabilism is a hypothesis in philosophy, in particular epistemology, and probability theory that holds that no facts are known for certain. That view holds profound implications for statistical inference. The philosophy is particularly associated with Richard Jeffrey who wittily characterised it with the ''dictum'' "It's probabilities all the way down." Background Bayes' theorem states a rule for updating a probability conditioned on other information. In 1967, Ian Hacking argued that in a static form, Bayes' theorem only connects probabilities that are held simultaneously; it does not tell the learner how to update probabilities when new evidence becomes available over time, contrary to what contemporary Bayesians suggested. According to Hacking, adopting Bayes' theorem is a temptation. Suppose that a learner forms probabilities ''P''old(''A'' & ''B'') = ''p'' and ''P''old(''B'') = ''q''. If the learner subsequently learns that ''B'' is ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Law Of Total Probability
In probability theory, the law (or formula) of total probability is a fundamental rule relating marginal probabilities to conditional probabilities. It expresses the total probability of an outcome which can be realized via several distinct events, hence the name. Statement The law of total probability isZwillinger, D., Kokoska, S. (2000) ''CRC Standard Probability and Statistics Tables and Formulae'', CRC Press. page 31. a theorem that states, in its discrete case, if \left\ is a finite or countably infinite partition of a sample space (in other words, a set of pairwise disjoint events whose union is the entire sample space) and each event B_n is measurable, then for any event A of the same probability space: :P(A)=\sum_n P(A\cap B_n) or, alternatively, :P(A)=\sum_n P(A\mid B_n)P(B_n), where, for any n for which P(B_n) = 0 these terms are simply omitted from the summation, because P(A\mid B_n) is finite. The summation can be interpreted as a weighted average, and consequ ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Borel–Kolmogorov Paradox
In probability theory, the Borel–Kolmogorov paradox (sometimes known as Borel's paradox) is a paradox relating to conditional probability with respect to an event of probability zero (also known as a null set). It is named after Émile Borel and Andrey Kolmogorov. A great circle puzzle Suppose that a random variable has a uniform distribution on a unit sphere. What is its conditional distribution on a great circle? Because of the symmetry of the sphere, one might expect that the distribution is uniform and independent of the choice of coordinates. However, two analyses give contradictory results. First, note that choosing a point uniformly on the sphere is equivalent to choosing the longitude \lambda uniformly from \pi,\pi/math> and choosing the latitude \varphi from \frac,\frac/math> with density \frac \cos \varphi. Then we can look at two different great circles: # If the coordinates are chosen so that the great circle is an equator (latitude \varphi = 0), the conditional ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Conditional Probability Distribution
In probability theory and statistics, given two jointly distributed random variables X and Y, the conditional probability distribution of Y given X is the probability distribution of Y when X is known to be a particular value; in some cases the conditional probabilities may be expressed as functions containing the unspecified value x of X as a parameter. When both X and Y are categorical variables, a conditional probability table is typically used to represent the conditional probability. The conditional distribution contrasts with the marginal distribution of a random variable, which is its distribution without reference to the value of the other variable. If the conditional distribution of Y given X is a continuous distribution, then its probability density function is known as the conditional density function. The properties of a conditional distribution, such as the moments, are often referred to by corresponding names such as the conditional mean and conditional variance. Mo ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Leibniz Integral Rule
In calculus, the Leibniz integral rule for differentiation under the integral sign, named after Gottfried Leibniz, states that for an integral of the form \int_^ f(x,t)\,dt, where -\infty < a(x), b(x) < \infty and the integral are functions dependent on the derivative of this integral is expressible as where the partial derivative indicates that inside the integral, only the variation of with is considered in taking the derivative. In the special case where the functions and are constants |
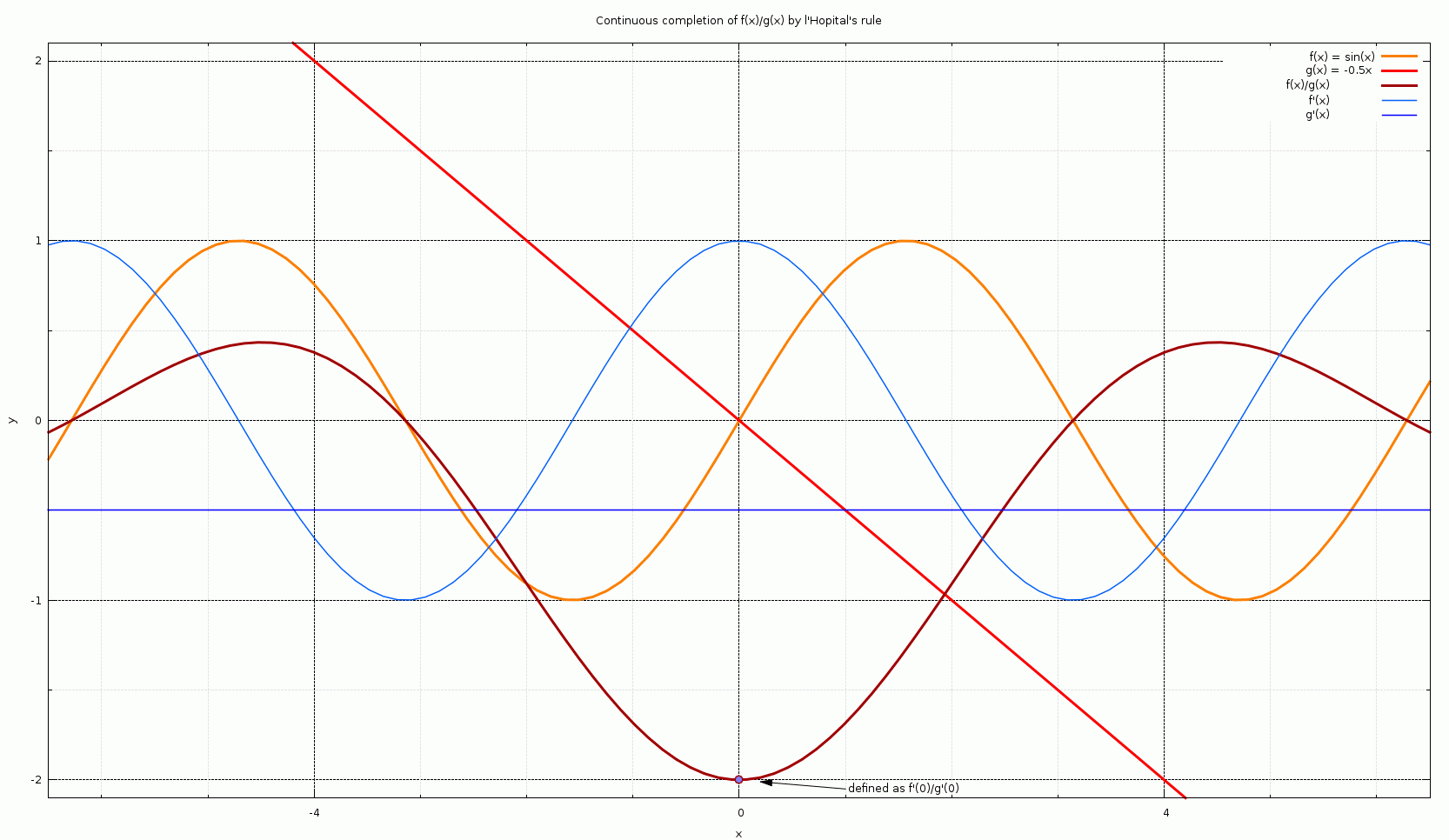
L'Hôpital's Rule
In calculus, l'Hôpital's rule or l'Hospital's rule (, , ), also known as Bernoulli's rule, is a theorem which provides a technique to evaluate limits of indeterminate forms. Application (or repeated application) of the rule often converts an indeterminate form to an expression that can be easily evaluated by substitution. The rule is named after the 17th-century French mathematician Guillaume de l'Hôpital. Although the rule is often attributed to l'Hôpital, the theorem was first introduced to him in 1694 by the Swiss mathematician Johann Bernoulli. L'Hôpital's rule states that for functions and which are differentiable on an open interval except possibly at a point contained in , if \lim_f(x)=\lim_g(x)=0 \text \pm\infty, and g'(x)\ne 0 for all in with , and \lim_\frac exists, then :\lim_\frac = \lim_\frac. The differentiation of the numerator and denominator often simplifies the quotient or converts it to a limit that can be evaluated directly. History Guillaume ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Limit (mathematics)
In mathematics, a limit is the value that a function (or sequence) approaches as the input (or index) approaches some value. Limits are essential to calculus and mathematical analysis, and are used to define continuity, derivatives, and integrals. The concept of a limit of a sequence is further generalized to the concept of a limit of a topological net, and is closely related to limit and direct limit in category theory. In formulas, a limit of a function is usually written as : \lim_ f(x) = L, (although a few authors may use "Lt" instead of "lim") and is read as "the limit of of as approaches equals ". The fact that a function approaches the limit as approaches is sometimes denoted by a right arrow (→ or \rightarrow), as in :f(x) \to L \text x \to c, which reads "f of x tends to L as x tends to c". History Grégoire de Saint-Vincent gave the first definition of limit (terminus) of a geometric series in his work ''Opus Geometricum'' (1647): "The ''terminus'' of a pro ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Defined And Undefined
In mathematics, the term undefined is often used to refer to an expression which is not assigned an interpretation or a value (such as an indeterminate form, which has the propensity of assuming different values). The term can take on several different meanings depending on the context. For example: * In various branches of mathematics, certain concepts are introduced as primitive notions (e.g., the terms "point", "line" and "angle" in geometry). As these terms are not defined in terms of other concepts, they may be referred to as "undefined terms". * A function is said to be "undefined" at points outside of its domainfor example, the real-valued function f(x)=\sqrt is undefined for negative x (i.e., it assigns no value to negative arguments). * In algebra, some arithmetic operations may not assign a meaning to certain values of its operands (e.g., division by zero). In which case, the expressions involving such operands are termed "undefined". Undefined terms In ancient tim ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Goodman–Nguyen–Van Fraassen Algebra
A standard, Boolean algebra of events is a set of events related to one another by the familiar operations ''and'', ''or'', and ''not''. A conditional event algebra (CEA) contains not just ordinary events but also conditional events, which have the form "if ''A'', then ''B''". The usual purpose of a CEA is to enable the defining of a probability function, ''P'', that satisfies the equation ''P''(if ''A'' then ''B'') = ''P''(''A'' and ''B'') / ''P''(''A''). Motivation In standard probability theory, an event is a set of outcomes, any one of which would be an occurrence of the event. ''P''(''A''), the probability of event ''A'', is the sum of the probabilities of all ''A''-outcomes, ''P''(''B'') is the sum of the probabilities of all ''B''-outcomes, and ''P''(''A'' and ''B'') is the sum of the probabilities of all outcomes that are both ''A''-outcomes and ''B''-outcomes. In other words, ''and'', customarily represented by the logical symbol ∧, is interpreted as set intersection: ''P ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |