|
Chromatic (other)
Chromatic, a word ultimately derived from the Greek noun χρῶμα (''khrṓma''), which means "complexion" or "color", and then from the Greek adjective χρωματικός (''khrōmatikós''; "colored"), may refer to: In music *Chromatic scale, the western-tempered twelve-tone scale *Chromatic chord, chords built from tones chromatically altered from the native scale of the musical composition *Chromaticism, the use of chromatic scales, chords, and modulations *Total chromatic, the use of all twelve pitches of the chromatic scale in tonal music * Chromatic fantasia, a specific form of fantasia originating in sixteenth century Europe *The Chromatic button accordion *The chromatic harmonica *Chromatic genus, a genus of divisions of the tetrachord characterized by an upper interval of a minor third *Diatonic and chromatic, as a property of several structures, genres, and other features in music, often contrasted with ''diatonic'' *Chromatics (band), an American electronic music b ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Chromatic Scale
The chromatic scale (or twelve-tone scale) is a set of twelve pitches (more completely, pitch classes) used in tonal music, with notes separated by the interval of a semitone. Chromatic instruments, such as the piano, are made to produce the chromatic scale, while other instruments capable of continuously variable pitch, such as the trombone and violin, can also produce microtones, or notes between those available on a piano. Most music uses subsets of the chromatic scale such as diatonic scales. While the chromatic scale is fundamental in western music theory, it is seldom directly used in its entirety in musical compositions or improvisation. Definition The chromatic scale is a musical scale with twelve pitches, each a semitone, also known as a half-step, above or below its adjacent pitches. As a result, in 12-tone equal temperament (the most common tuning in Western music), the chromatic scale covers all 12 of the available pitches. Thus, there is only one chromatic scale ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
RG Chromaticity
The rg chromaticity space, two dimensions of the ''normalized RGB'', or rgb, space, is a chromaticity space, a two-dimensional color space in which there is no intensity information. In the RGB color space a pixel is identified by the intensity of red, green, and blue primary colors. Therefore, a bright red can be represented as (R,G,B) (255,0,0), while a dark red may be (40,0,0). In the normalized rgb space or rg space, a color is represented by the proportion of red, green, and blue in the color, rather than by the intensity of each. Since these proportions must always add up to a total of 1, we are able to quote just the red and green proportions of the color, and can calculate the blue value if necessary. Conversion between RGB and RG Chromaticity Given a color (R,G,B) where R, G, B = intensity of red, green and blue, this can be converted to color (r,g,b) where r, g, b imply the proportion of red, green and blue in the original color: r = \frac g = \frac b = \f ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Staining
Staining is a technique used to enhance contrast in samples, generally at the microscopic level. Stains and dyes are frequently used in histology (microscopic study of biological tissues), in cytology (microscopic study of cells), and in the medical fields of histopathology, hematology, and cytopathology that focus on the study and diagnoses of diseases at the microscopic level. Stains may be used to define biological tissues (highlighting, for example, muscle fibers or connective tissue), cell populations (classifying different blood cells), or organelles within individual cells. In biochemistry, it involves adding a class-specific ( DNA, proteins, lipids, carbohydrates) dye to a substrate to qualify or quantify the presence of a specific compound. Staining and fluorescent tagging can serve similar purposes. Biological staining is also used to mark cells in flow cytometry, and to flag proteins or nucleic acids in gel electrophoresis. Light microscopes are used for viewin ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Chromatics Inc
Chromatic, a word ultimately derived from the Greek noun χρῶμα (''khrṓma''), which means "complexion" or "color", and then from the Greek adjective χρωματικός (''khrōmatikós''; "colored"), may refer to: In music *Chromatic scale, the western-tempered twelve-tone scale *Chromatic chord, chords built from tones chromatically altered from the native scale of the musical composition *Chromaticism, the use of chromatic scales, chords, and modulations *Total chromatic, the use of all twelve pitches of the chromatic scale in tonal music *Chromatic fantasia, a specific form of fantasia originating in sixteenth century Europe *The Chromatic button accordion *The chromatic harmonica *Chromatic genus, a genus of divisions of the tetrachord characterized by an upper interval of a minor third *Diatonic and chromatic, as a property of several structures, genres, and other features in music, often contrasted with ''diatonic'' *Chromatics (band), an American electronic music ba ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Chromatic (programmer)
Chromatic is a writer and free software programmer best known for his work in the Perl programming language. He lives in Hillsboro, Oregon, United States. He wrote ''Extreme Programming Pocket Guide'', co-wrote ''Perl Testing: A Developer's Notebook'', is the lead author of ''Perl Hacks'', and an uncredited contributor to ''The Art of Agile Development''. He has a music degree. Also, he has contributed to CPAN, Perl 5, Perl 6 Raku is a member of the Perl family of programming languages. Formerly known as Perl 6, it was renamed in October 2019. Raku introduces elements of many modern and historical languages. Compatibility with Perl was not a goal, though a compatibili ..., and Parrot. In 2009, he founded Modern Perl Books, in part to revitalize the world of Perl and to publish materials that other publishers had neglected. In 2010, he released the book ''Modern Perl'' in print and in electronic form, with the latter redistributable freely (though with a suggested don ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
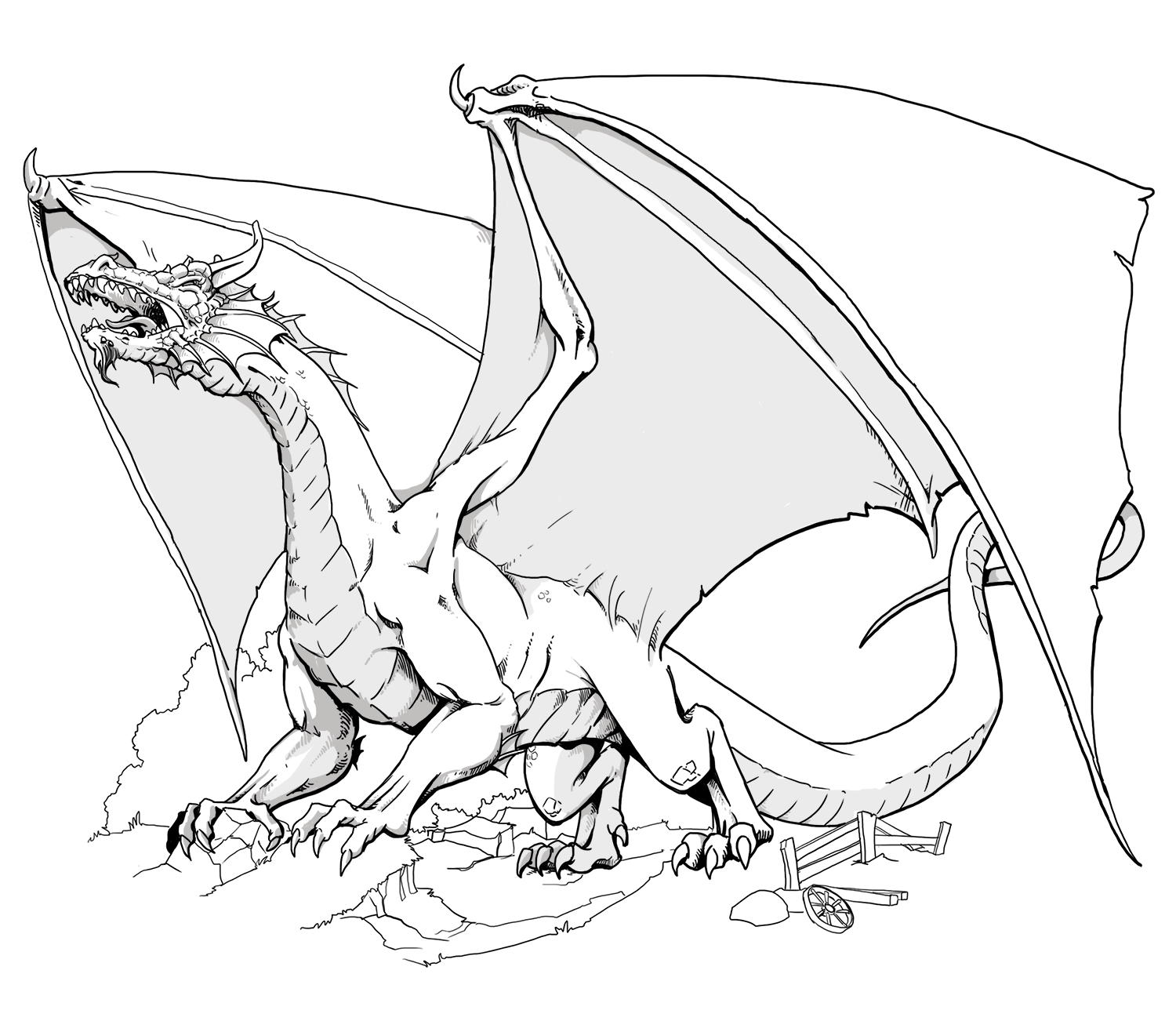
Chromatic Dragon
In the ''Dungeons & Dragons'' (''D&D'') fantasy role-playing game, dragons are an iconic type of monstrous creature. As a group, ''D&D'' dragons are loosely based upon dragons from a wide range of fictional and mythological sources. Dungeons & Dragons allows players to fight its fictional dragons (Tiamat being one of the most notable) and "slay their psychic dragons" as well. These dragons, specifically their "dungeon ecology", have implications for the literary theory of fantasy writing. ''D&D'' dragons also featured as targets of the moral panic surrounding the game. In ''D&D'', dragons are depicted as any of various species of large, intelligent, magical, reptilian beasts, each typically defined by a combination of their demeanor and either the color of their scales or their elemental affinity. For example, a commonly presented species of dragon is the red dragon, which is named for its red scales, and known for its evil and greedy nature, as well as its ability to breathe ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Von Luschan's Chromatic Scale
Von Luschan's chromatic scale (VLS) is a method of classifying skin color. It is also called the von Luschan scale or von Luschan's scale. It is named after its inventor, Felix von Luschan. The equipment consists of 36 opaque glass tiles which were compared to the subject's skin, ideally in a place which would not be exposed to the sun (such as under the arm). The von Luschan scale was used to establish racial classifications of populations according to skin color; in this respect it is in contrast to the Fitzpatrick scale intended for the classification of the skin type of individuals introduced in 1975 by Harvard dermatologist Thomas B. Fitzpatrick to describe sun tanning behavior. The von Luschan scale was used extensively throughout the first half of the 20th century in race studies and anthropometry. However, the results were inconsistent: in many instances, different investigators would give different readings of the same person. The von Luschan scale was largely abando ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Vertex Chromatic Number
In graph theory, graph coloring is a special case of graph labeling; it is an assignment of labels traditionally called "colors" to elements of a Graph (discrete mathematics), graph subject to certain constraints. In its simplest form, it is a way of coloring the Vertex (graph theory), vertices of a graph such that no two adjacent vertices are of the same color; this is called a vertex coloring. Similarly, an edge coloring assigns a color to each edge so that no two adjacent edges are of the same color, and a face coloring of a planar graph assigns a color to each face or region so that no two faces that share a boundary have the same color. Vertex coloring is often used to introduce graph coloring problems, since other coloring problems can be transformed into a vertex coloring instance. For example, an edge coloring of a graph is just a vertex coloring of its line graph, and a face coloring of a plane graph is just a vertex coloring of its dual graph, dual. However, non-vertex ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Fractional Chromatic Number
Fractional coloring is a topic in a young branch of graph theory known as fractional graph theory. It is a generalization of ordinary graph coloring. In a traditional graph coloring, each vertex in a graph is assigned some color, and adjacent vertices — those connected by edges — must be assigned different colors. In a fractional coloring however, a ''set'' of colors is assigned to each vertex of a graph. The requirement about adjacent vertices still holds, so if two vertices are joined by an edge, they must have no colors in common. Fractional graph coloring can be viewed as the linear programming relaxation of traditional graph coloring. Indeed, fractional coloring problems are much more amenable to a linear programming approach than traditional coloring problems. Definitions A ''b''-fold coloring of a graph ''G'' is an assignment of sets of size ''b'' to vertices of a graph such that adjacent vertices receive disjoint sets. An ''a'':''b''-coloring is a ''b''-fold colorin ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Strong Chromatic Number
In graph theory, a strong coloring, with respect to a partition of the vertices into (disjoint) subsets of equal sizes, is a (proper) vertex coloring in which every color appears exactly once in every part. A graph is strongly ''k''-colorable if, for each partition of the vertices into sets of size ''k'', it admits a strong coloring. When the order of the graph ''G'' is not divisible by ''k'', we add isolated vertices to ''G'' just enough to make the order of the new graph ' divisible by ''k''. In that case, a strong coloring of ' minus the previously added isolated vertices is considered a strong coloring of ''G''. The strong chromatic number sχ(''G'') of a graph ''G'' is the least ''k'' such that ''G'' is strongly ''k''-colorable. A graph is strongly ''k''-chromatic if it has strong chromatic number ''k''. Some properties of sχ(''G''): # sχ(''G'') > Δ(''G''). # sχ(''G'') ≤ 3 Δ(''G'') − 1. # Asymptotically, sχ(''G'') ≤ 11 Δ(''G'') / 4 + o(Δ(''G'')). Here, ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Acyclic Chromatic Number
In graph theory, an acyclic coloring is a (proper) vertex coloring in which every 2-chromatic subgraph is acyclic. The acyclic chromatic number of a graph is the fewest colors needed in any acyclic coloring of . Acyclic coloring is often associated with graphs embedded on non-plane surfaces. Upper bounds A(''G'') ≤ 2 if and only if ''G'' is acyclic. Bounds on A(''G'') in terms of Δ(''G''), the maximum degree of ''G'', include the following: * A(''G'') ≤ 4 if Δ(''G'') = 3. * A(''G'') ≤ 5 if Δ(''G'') = 4. * A(''G'') ≤ 7 if Δ(''G'') = 5. * A(''G'') ≤ 12 if Δ(''G'') = 6. A milestone in the study of acyclic coloring is the following affirmative answer to a conjecture of Grünbaum: :Theorem A(''G'') ≤ 5 if ''G'' is planar graph. introduced acyclic coloring and acyclic chromatic number, and conjectured the result in the above theorem. Borodin's proof involved several years of painstaking inspection of 450 reducible configurations. One consequence of t ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Chromatic Index
In graph theory, an edge coloring of a graph is an assignment of "colors" to the edges of the graph so that no two incident edges have the same color. For example, the figure to the right shows an edge coloring of a graph by the colors red, blue, and green. Edge colorings are one of several different types of graph coloring. The edge-coloring problem asks whether it is possible to color the edges of a given graph using at most different colors, for a given value of , or with the fewest possible colors. The minimum required number of colors for the edges of a given graph is called the chromatic index of the graph. For example, the edges of the graph in the illustration can be colored by three colors but cannot be colored by two colors, so the graph shown has chromatic index three. By Vizing's theorem, the number of colors needed to edge color a simple graph is either its maximum degree or . For some graphs, such as bipartite graphs and high-degree planar graphs, the number of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |