|
Curriculum Of The Waldorf Schools
In the curriculum of the Waldorf schools, much of the education in academic subjects takes place in blocks, generally of 3–5 weeks duration. Each pupil generally writes and illustrates a self-created textbook representing the material learned in the block.Todd Oppenheimer, ''Schooling the Imagination'', Atlantic Monthly, Sept. 99 These blocks are supported by on-going classes in subjects such as music, art and crafts, and foreign languages that continue throughout the year. Curricular Approach In a Waldorf elementary school, the curriculum is presented through extended "main lessons" which focus on one subject in depth. This approach differs from other instructional approaches that allot equal time to every subject. In a Waldorf elementary school, the approximately two-hour-long main lesson "ties one topic to as many disciplines as possible".unger The main lesson is not taught from a textbook. The teacher will draw one colored chalk drawing on the board to introduce the them ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Curriculum
In education, a curriculum (; : curricula or curriculums) is broadly defined as the totality of student experiences that occur in the educational process. The term often refers specifically to a planned sequence of instruction, or to a view of the student's experiences in terms of the educator's or school's instructional goals. A curriculum may incorporate the planned interaction of pupils with instructional content, materials, resources, and processes for evaluating the attainment of educational objectives. Curricula are split into several categories: the explicit, the implicit (including the hidden), the excluded, and the extracurricular.Kelly, A. V. (2009). The curriculum: Theory and practice (pp. 1–55). Newbury Park, CA: Sage.Braslavsky, C. (2003). The curriculum. Curricula may be tightly standardized or may include a high level of instructor or learner autonomy. Many countries have national curricula in primary and secondary education, such as the United Kingdom's Na ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Reformation
The Reformation (alternatively named the Protestant Reformation or the European Reformation) was a major movement within Western Christianity in 16th-century Europe that posed a religious and political challenge to the Catholic Church and in particular to papal authority, arising from what were perceived to be errors, abuses, and discrepancies by the Catholic Church. The Reformation was the start of Protestantism and the split of the Western Church into Protestantism and what is now the Roman Catholic Church. It is also considered to be one of the events that signified the end of the Middle Ages and the beginning of the early modern period in Europe.Davies ''Europe'' pp. 291–293 Prior to Martin Luther, there were many earlier reform movements. Although the Reformation is usually considered to have started with the publication of the '' Ninety-five Theses'' by Martin Luther in 1517, he was not excommunicated by Pope Leo X until January 1521. The Diet of Worms of May 152 ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Combinatorics
Combinatorics is an area of mathematics primarily concerned with counting, both as a means and an end in obtaining results, and certain properties of finite structures. It is closely related to many other areas of mathematics and has many applications ranging from logic to statistical physics and from evolutionary biology to computer science. Combinatorics is well known for the breadth of the problems it tackles. Combinatorial problems arise in many areas of pure mathematics, notably in algebra, probability theory, topology, and geometry, as well as in its many application areas. Many combinatorial questions have historically been considered in isolation, giving an ''ad hoc'' solution to a problem arising in some mathematical context. In the later twentieth century, however, powerful and general theoretical methods were developed, making combinatorics into an independent branch of mathematics in its own right. One of the oldest and most accessible parts of combinatorics is gra ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Probability
Probability is the branch of mathematics concerning numerical descriptions of how likely an Event (probability theory), event is to occur, or how likely it is that a proposition is true. The probability of an event is a number between 0 and 1, where, roughly speaking, 0 indicates impossibility of the event and 1 indicates certainty."Kendall's Advanced Theory of Statistics, Volume 1: Distribution Theory", Alan Stuart and Keith Ord, 6th Ed, (2009), .William Feller, ''An Introduction to Probability Theory and Its Applications'', (Vol 1), 3rd Ed, (1968), Wiley, . The higher the probability of an event, the more likely it is that the event will occur. A simple example is the tossing of a fair (unbiased) coin. Since the coin is fair, the two outcomes ("heads" and "tails") are both equally probable; the probability of "heads" equals the probability of "tails"; and since no other outcomes are possible, the probability of either "heads" or "tails" is 1/2 (which could also be written ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Trigonometry
Trigonometry () is a branch of mathematics that studies relationships between side lengths and angles of triangles. The field emerged in the Hellenistic world during the 3rd century BC from applications of geometry to astronomical studies. The Greeks focused on the calculation of chords, while mathematicians in India created the earliest-known tables of values for trigonometric ratios (also called trigonometric functions) such as sine. Throughout history, trigonometry has been applied in areas such as geodesy, surveying, celestial mechanics, and navigation. Trigonometry is known for its many identities. These trigonometric identities are commonly used for rewriting trigonometrical expressions with the aim to simplify an expression, to find a more useful form of an expression, or to solve an equation. History Sumerian astronomers studied angle measure, using a division of circles into 360 degrees. They, and later the Babylonians, studied the ratios of the sides of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
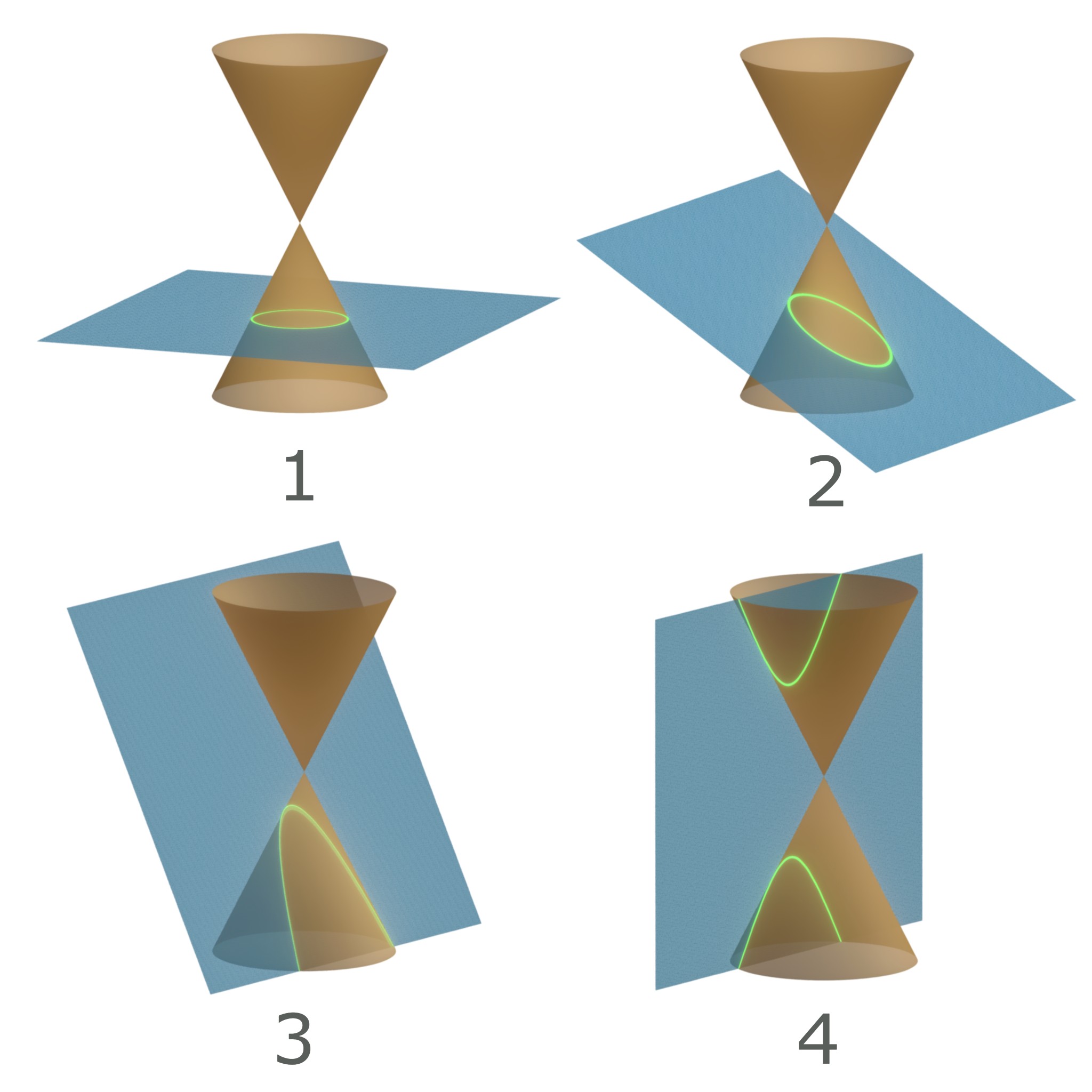
Conic Sections
In mathematics, a conic section, quadratic curve or conic is a curve obtained as the intersection of the surface of a cone with a plane. The three types of conic section are the hyperbola, the parabola, and the ellipse; the circle is a special case of the ellipse, though historically it was sometimes called a fourth type. The ancient Greek mathematicians studied conic sections, culminating around 200 BC with Apollonius of Perga's systematic work on their properties. The conic sections in the Euclidean plane have various distinguishing properties, many of which can be used as alternative definitions. One such property defines a non-circular conic to be the set of those points whose distances to some particular point, called a ''focus'', and some particular line, called a ''directrix'', are in a fixed ratio, called the ''eccentricity''. The type of conic is determined by the value of the eccentricity. In analytic geometry, a conic may be defined as a plane algebraic curve of deg ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Geometry
Geometry (; ) is, with arithmetic, one of the oldest branches of mathematics. It is concerned with properties of space such as the distance, shape, size, and relative position of figures. A mathematician who works in the field of geometry is called a ''geometer''. Until the 19th century, geometry was almost exclusively devoted to Euclidean geometry, which includes the notions of point, line, plane, distance, angle, surface, and curve, as fundamental concepts. During the 19th century several discoveries enlarged dramatically the scope of geometry. One of the oldest such discoveries is Carl Friedrich Gauss' ("remarkable theorem") that asserts roughly that the Gaussian curvature of a surface is independent from any specific embedding in a Euclidean space. This implies that surfaces can be studied ''intrinsically'', that is, as stand-alone spaces, and has been expanded into the theory of manifolds and Riemannian geometry. Later in the 19th century, it appeared that geometries ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Algebra
Algebra () is one of the broad areas of mathematics. Roughly speaking, algebra is the study of mathematical symbols and the rules for manipulating these symbols in formulas; it is a unifying thread of almost all of mathematics. Elementary algebra deals with the manipulation of variables (commonly represented by Roman letters) as if they were numbers and is therefore essential in all applications of mathematics. Abstract algebra is the name given, mostly in education, to the study of algebraic structures such as groups, rings, and fields (the term is no more in common use outside educational context). Linear algebra, which deals with linear equations and linear mappings, is used for modern presentations of geometry, and has many practical applications (in weather forecasting, for example). There are many areas of mathematics that belong to algebra, some having "algebra" in their name, such as commutative algebra, and some not, such as Galois theory. The word ''algebra'' is ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
American Transcendentalism
Transcendentalism is a philosophical movement that developed in the late 1820s and 1830s in New England. "Transcendentalism is an American literary, political, and philosophical movement of the early nineteenth century, centered around Ralph Waldo Emerson." A core belief is in the inherent goodness of people and nature, and while society and its institutions have corrupted the purity of the individual, people are at their best when truly "self-reliant" and independent. Transcendentalists saw divine experience inherent in the everyday, rather than believing in a distant heaven. Transcendentalists saw physical and spiritual phenomena as part of dynamic processes rather than discrete entities. Transcendentalism is one of the first philosophical currents that emerged in the United States;Coviello, Peter. "Transcendentalism" ''The Oxford Encyclopedia of American Literature''. Oxford University Press, 2004. ''Oxford Reference Online''. Web. 23 Oct. 2011 it is therefore a key early point ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Goethe's Faust
''Faust'' is a tragic play in two parts by Johann Wolfgang von Goethe, usually known in English as '' Faust, Part One'' and ''Faust, Part Two''. Nearly all of Part One and the majority of Part Two are written in rhymed verse. Although rarely staged in its entirety, it is the play with the largest audience numbers on German-language stages. ''Faust'' is considered by many to be Goethe's ''magnum opus'' and the greatest work of German literature. The earliest forms of the work, known as the ''Urfaust'', were developed between 1772 and 1775; however, the details of that development are not entirely clear. ''Urfaust'' has twenty-two scenes, one in prose, two largely prose and the remaining 1,441 lines in rhymed verse. The manuscript is lost, but a copy was discovered in 1886. The first appearance of the work in print was ''Faust, a Fragment'', published in 1790. Goethe completed a preliminary version of what is now known as ''Part One'' in 1806. Its publication in 1808 was follow ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Goethe
Johann Wolfgang von Goethe (28 August 1749 – 22 March 1832) was a German poet, playwright, novelist, scientist, statesman, theatre director, and critic. His works include plays, poetry, literature, and aesthetic criticism, as well as treatises on botany, anatomy, and colour. He is widely regarded as the greatest and most influential writer in the German language, his work having a profound and wide-ranging influence on Western literary, political, and philosophical thought from the late 18th century to the present day.. Goethe took up residence in Weimar in November 1775 following the success of his first novel, ''The Sorrows of Young Werther'' (1774). He was ennobled by the Duke of Saxe-Weimar, Karl August, in 1782. Goethe was an early participant in the ''Sturm und Drang'' literary movement. During his first ten years in Weimar, Goethe became a member of the Duke's privy council (1776–1785), sat on the war and highway commissions, oversaw the reopening of silver mines ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Parsifal
''Parsifal'' ( WWV 111) is an opera or a music drama in three acts by the German composer Richard Wagner and his last composition. Wagner's own libretto for the work is loosely based on the 13th-century Middle High German epic poem ''Parzival'' of the ''Minnesänger'' Wolfram von Eschenbach, recounting the story of the Arthurian knight Parzival (Percival) and his quest for the Holy Grail. Wagner conceived the work in April 1857, but did not finish it until 25 years later. In composing it he took advantage of the particular acoustics of his Bayreuth Festspielhaus. ''Parsifal'' was first produced at the second Bayreuth Festival in 1882. The Bayreuth Festival maintained a monopoly on ''Parsifal'' productions until 1903, when the opera was performed at the Metropolitan Opera in New York. Wagner described ''Parsifal'' not as an opera, but as (a festival play for the consecration of the stage). At Bayreuth a tradition has arisen that audiences do not applaud at the end of the first ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |


.jpg)