|
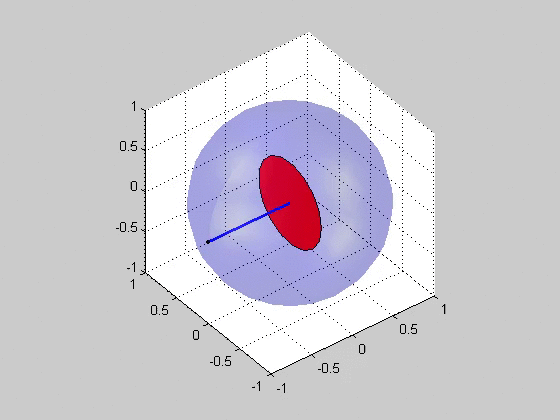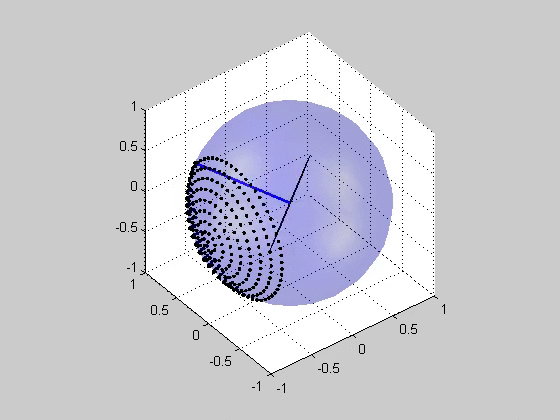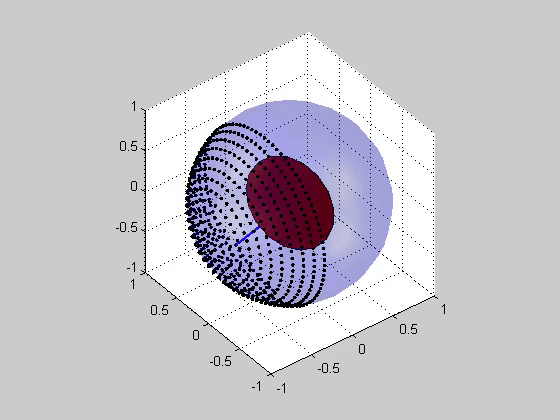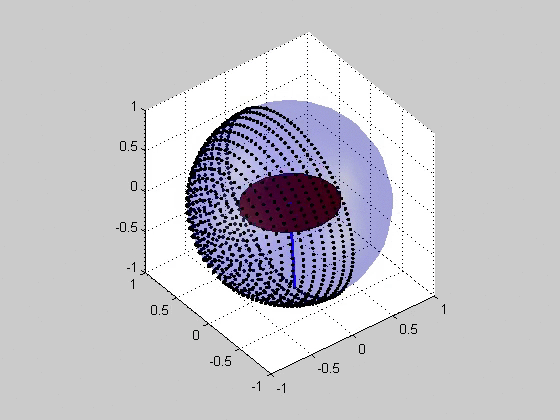
Critical Plane Analysis
Critical plane analysis refers to the analysis of stresses or strains as they are experienced by a particular plane in a material, as well as the identification of which plane is likely to experience the most extreme damage. Critical plane analysis is widely used in engineering to account for the effects of cyclic, multiaxial load histories on the fatigue life of materials and structures. When a structure is under cyclic multiaxial loading, it is necessary to use multiaxial fatigue criteria that account for the multiaxial loading. If the cyclic multiaxial loading is nonproportional it is mandatory to use a proper multiaxial fatigue criteria. The multiaxial criteria based on the Critical Plane Method are the most effective criteria. For the plane stress case, the orientation of the plane may be specified by an angle in the plane, and the stresses and strains acting on this plane may be computed via Mohr's circle Mohr's circle is a two-dimensional graphical representation of t ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Stress (mechanics)
In continuum mechanics, stress is a physical quantity. It is a quantity that describes the magnitude of forces that cause deformation. Stress is defined as ''force per unit area''. When an object is pulled apart by a force it will cause elongation which is also known as deformation, like the stretching of an elastic band, it is called tensile stress. But, when the forces result in the compression of an object, it is called compressive stress. It results when forces like tension or compression act on a body. The greater this force and the smaller the cross-sectional area of the body on which it acts, the greater the stress. Therefore, stress is measured in newton per square meter (N/m2) or pascal (Pa). Stress expresses the internal forces that neighbouring particles of a continuous material exert on each other, while strain is the measure of the deformation of the material. For example, when a solid vertical bar is supporting an overhead weight, each particle in the bar pu ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Deformation (mechanics)
In physics, deformation is the continuum mechanics transformation of a body from a ''reference'' configuration to a ''current'' configuration. A configuration is a set containing the positions of all particles of the body. A deformation can occur because of external loads, intrinsic activity (e.g. muscle contraction), body forces (such as gravity or electromagnetic forces), or changes in temperature, moisture content, or chemical reactions, etc. Strain is related to deformation in terms of ''relative'' displacement of particles in the body that excludes rigid-body motions. Different equivalent choices may be made for the expression of a strain field depending on whether it is defined with respect to the initial or the final configuration of the body and on whether the metric tensor or its dual is considered. In a continuous body, a deformation field results from a stress field due to applied forces or because of some changes in the temperature field of the body. The relat ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Fatigue (material)
In materials science, fatigue is the initiation and propagation of cracks in a material due to cyclic loading. Once a fatigue crack has initiated, it grows a small amount with each loading cycle, typically producing striations on some parts of the fracture surface. The crack will continue to grow until it reaches a critical size, which occurs when the stress intensity factor of the crack exceeds the fracture toughness of the material, producing rapid propagation and typically complete fracture of the structure. Fatigue has traditionally been associated with the failure of metal components which led to the term metal fatigue. In the nineteenth century, the sudden failing of metal railway axles was thought to be caused by the metal ''crystallising'' because of the brittle appearance of the fracture surface, but this has since been disproved. Most materials, such as composites, plastics and ceramics, seem to experience some sort of fatigue-related failure. To aid in predicting ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Mohr's Circle
Mohr's circle is a two-dimensional graphical representation of the transformation law for the Cauchy stress tensor. Mohr's circle is often used in calculations relating to mechanical engineering for materials' strength, geotechnical engineering for strength of soils, and structural engineering for strength of built structures. It is also used for calculating stresses in many planes by reducing them to vertical and horizontal components. These are called principal planes in which principal stresses are calculated; Mohr's circle can also be used to find the principal planes and the principal stresses in a graphical representation, and is one of the easiest ways to do so. After performing a stress analysis on a material body assumed as a continuum, the components of the Cauchy stress tensor at a particular material point are known with respect to a coordinate system. The Mohr circle is then used to determine graphically the stress components acting on a rotated coordinate s ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Cauchy Stress Tensor
In continuum mechanics, the Cauchy stress tensor \boldsymbol\sigma, true stress tensor, or simply called the stress tensor is a second order tensor named after Augustin-Louis Cauchy. The tensor consists of nine components \sigma_ that completely define the state of stress at a point inside a material in the deformed state, placement, or configuration. The tensor relates a unit-length direction vector e to the traction vector T(e) across an imaginary surface perpendicular to e: :\mathbf^ = \mathbf e \cdot\boldsymbol\quad \text \quad T_^= \sigma_e_i, or, :\leftright\leftrightcdot \leftright The SI units of both stress tensor and traction vector are N/m2, corresponding to the stress scalar. The unit vector is dimensionless. The Cauchy stress tensor obeys the tensor transformation law under a change in the system of coordinates. A graphical representation of this transformation law is the Mohr's circle for stress. The Cauchy stress tensor is used for stress analysis of mate ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Critical Plane Analysis
Critical plane analysis refers to the analysis of stresses or strains as they are experienced by a particular plane in a material, as well as the identification of which plane is likely to experience the most extreme damage. Critical plane analysis is widely used in engineering to account for the effects of cyclic, multiaxial load histories on the fatigue life of materials and structures. When a structure is under cyclic multiaxial loading, it is necessary to use multiaxial fatigue criteria that account for the multiaxial loading. If the cyclic multiaxial loading is nonproportional it is mandatory to use a proper multiaxial fatigue criteria. The multiaxial criteria based on the Critical Plane Method are the most effective criteria. For the plane stress case, the orientation of the plane may be specified by an angle in the plane, and the stresses and strains acting on this plane may be computed via Mohr's circle Mohr's circle is a two-dimensional graphical representation of t ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Sines Rule
Sines () is a city and a municipality in Portugal. The municipality, divided into two parishes, has around 14,214 inhabitants (2021) in an area of . Sines holds an important oil refinery and several petrochemical industries. It is also a popular beach spot and the main fishing harbor of Alentejo region. The municipality is bordered to the north and east by the municipality of Santiago do Cacém, south by Odemira and west by the Atlantic Ocean. The coastline of the city, south of São Torpes, is part of the Southwest Alentejo and Vicentine Coast Natural Park. History Vestiges of a few settlements have today been discovered in archaeological sites, such as Palmeirinha and Quitéria, that attest to the age of human settlements in Sines. Arnaldo Soledade (1981) noted that these Visigoths, identified as ''Cinetos'', may have been the original civilization that gave rise to the community, suggesting the local toponymy may have derived from this; ''Cinetos'', to Cines and, finally, ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Cauchy Stress Tensor
In continuum mechanics, the Cauchy stress tensor \boldsymbol\sigma, true stress tensor, or simply called the stress tensor is a second order tensor named after Augustin-Louis Cauchy. The tensor consists of nine components \sigma_ that completely define the state of stress at a point inside a material in the deformed state, placement, or configuration. The tensor relates a unit-length direction vector e to the traction vector T(e) across an imaginary surface perpendicular to e: :\mathbf^ = \mathbf e \cdot\boldsymbol\quad \text \quad T_^= \sigma_e_i, or, :\leftright\leftrightcdot \leftright The SI units of both stress tensor and traction vector are N/m2, corresponding to the stress scalar. The unit vector is dimensionless. The Cauchy stress tensor obeys the tensor transformation law under a change in the system of coordinates. A graphical representation of this transformation law is the Mohr's circle for stress. The Cauchy stress tensor is used for stress analysis of mate ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Strain Energy Density Function
A strain energy density function or stored energy density function is a scalar-valued function that relates the strain energy density of a material to the deformation gradient. : W = \hat(\boldsymbol) = \hat(\boldsymbol^T\cdot\boldsymbol) =\bar(\boldsymbol) = \bar(\boldsymbol^\cdot\boldsymbol)=\tilde(\boldsymbol,\boldsymbol) Equivalently, : W = \hat(\boldsymbol) = \hat(\boldsymbol^T\cdot\boldsymbol\cdot\boldsymbol) =\tilde(\boldsymbol,\boldsymbol) where \boldsymbol is the (two-point) deformation gradient tensor, \boldsymbol is the right Cauchy–Green deformation tensor, \boldsymbol is the left Cauchy–Green deformation tensor, and \boldsymbol is the rotation tensor from the polar decomposition of \boldsymbol. For an anisotropic material, the strain energy density function \hat(\boldsymbol) depends implicitly on reference vectors or tensors (such as the initial orientation of fibers in a composite) that characterize internal material texture. The spatial represe ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Materials Degradation
Material is a substance or mixture of substances that constitutes an object. Materials can be pure or impure, living or non-living matter. Materials can be classified on the basis of their physical and chemical properties, or on their geological origin or biological function. Materials science is the study of materials, their properties and their applications. Raw materials can be processed in different ways to influence their properties, by purification, shaping or the introduction of other materials. New materials can be produced from raw materials by synthesis. In industry, materials are inputs to manufacturing processes to produce products or more complex materials. Historical elements Materials chart the history of humanity. The system of the three prehistoric ages ( Stone Age, Bronze Age, Iron Age) were succeeded by historical ages: steel age in the 19th century, polymer age in the middle of the following century (plastic age) and silicon age in the second half of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Fracture Mechanics
Fracture mechanics is the field of mechanics concerned with the study of the propagation of cracks in materials. It uses methods of analytical solid mechanics to calculate the driving force on a crack and those of experimental solid mechanics to characterize the material's resistance to fracture. Theoretically, the stress ahead of a sharp crack tip becomes infinite and cannot be used to describe the state around a crack. Fracture mechanics is used to characterise the loads on a crack, typically using a single parameter to describe the complete loading state at the crack tip. A number of different parameters have been developed. When the plastic zone at the tip of the crack is small relative to the crack length the stress state at the crack tip is the result of elastic forces within the material and is termed linear elastic fracture mechanics (LEFM) and can be characterised using the stress intensity factor K. Although the load on a crack can be arbitrary, in 1957 G. Irwin f ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |