|
Chinese Mathematics
Mathematics emerged independently in China by the 11th century BCE. The Chinese independently developed a real number system that includes significantly large and negative numbers, more than one numeral system (base 2, binary and base 10, decimal), algebra, geometry, number theory and trigonometry. Since the Han dynasty, as diophantine approximation being a prominent numerical method, the Chinese made substantial progress on polynomial evaluation. Algorithms like regula falsi and expressions like simple continued fractions are widely used and have been well-documented ever since. They deliberately find the principal nth root, ''n''th root of positive numbers and the zero of a function, roots of equations. The major texts from the period, ''The Nine Chapters on the Mathematical Art'' and the ''Book on Numbers and Computation'' gave detailed processes for solving various mathematical problems in daily life. All procedures were computed using a counting board in both texts, and they ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Zero Of A Function
In mathematics, a zero (also sometimes called a root) of a real-, complex-, or generally vector-valued function f, is a member x of the domain of f such that f(x) ''vanishes'' at x; that is, the function f attains the value of 0 at x, or equivalently, x is a solution to the equation f(x) = 0. A "zero" of a function is thus an input value that produces an output of 0. A root of a polynomial is a zero of the corresponding polynomial function. The fundamental theorem of algebra shows that any non-zero polynomial has a number of roots at most equal to its degree, and that the number of roots and the degree are equal when one considers the complex roots (or more generally, the roots in an algebraically closed extension) counted with their multiplicities. For example, the polynomial f of degree two, defined by f(x)=x^2-5x+6=(x-2)(x-3) has the two roots (or zeros) that are 2 and 3. f(2)=2^2-5\times 2+6= 0\textf(3)=3^2-5\times 3+6=0. If the function maps real numbers to real n ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Duke Of Zhou
Dan, Duke Wen of Zhou, commonly known as the Duke of Zhou, was a member of the royal family of the early Zhou dynasty who played a major role in consolidating the kingdom established by his elder brother King Wu. He was renowned for acting as a capable and loyal regent for his young nephew King Cheng, and for successfully suppressing the Rebellion of the Three Guards and establishing firm rule of the Zhou dynasty over eastern China. He is also a Chinese culture hero, with the authorship of the ''I Ching'' and the ''Classic of Poetry'' having traditionally been attributed to him, as well as the establishment of the '' Rites of Zhou''. Life His personal name was Dan (). He was the fourth son of King Wen of Zhou and Queen Tai Si. His eldest brother Bo Yikao predeceased their father (supposedly a victim of cannibalism); the second-eldest defeated the Shang dynasty at the Battle of Muye around 1046 BC, ascending the throne as King Wu. King Wu distributed many fiefs to his r ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Zhoubi Suanjing
The ''Zhoubi Suanjing'', also known by many other names, is an ancient Chinese astronomical and mathematical work. The ''Zhoubi'' is most famous for its presentation of Chinese cosmology and a form of the Pythagorean theorem. It claims to present 246 problems worked out by the Duke of Zhou as well as members of his court, placing its composition during the 11th century BC. However, the present form of the book does not seem to be earlier than the Eastern Han (25–220 AD), with some additions and commentaries continuing to be added for several more centuries. The book was included as part of the '' Ten Computational Canons''. Names The work's original title was simply the ''Zhoubi'': the character is a literary term for the femur or thighbone but in context only refers to one or more gnomons, large sticks whose shadows were used for Chinese calendrical and astronomical calculations. Because of the ambiguous nature of the character , it has been alternatel ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
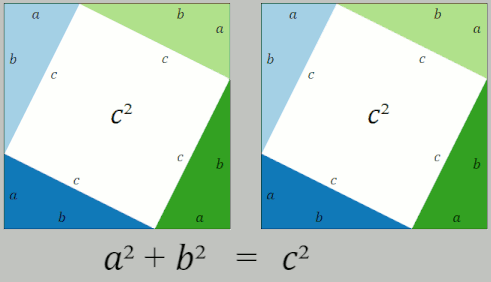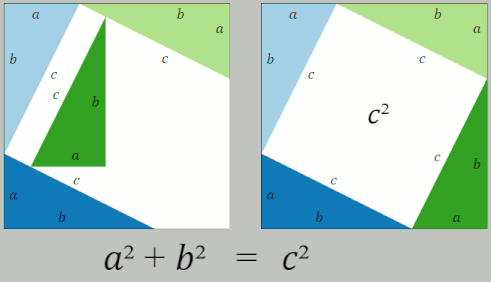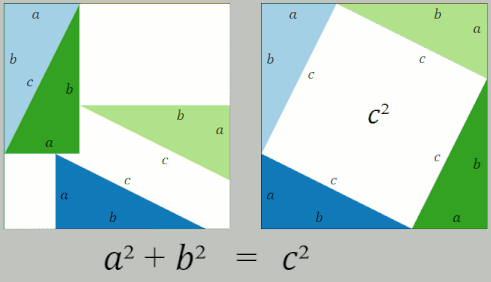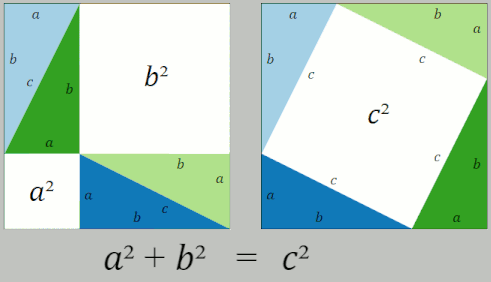
Pythagorean Theorem
In mathematics, the Pythagorean theorem or Pythagoras' theorem is a fundamental relation in Euclidean geometry between the three sides of a right triangle. It states that the area of the square whose side is the hypotenuse (the side opposite the right angle) is equal to the sum of the areas of the squares on the other two sides. The theorem can be written as an equation relating the lengths of the sides , and the hypotenuse , sometimes called the Pythagorean equation: :a^2 + b^2 = c^2 . The theorem is named for the Ancient Greece, Greek philosopher Pythagoras, born around 570 BC. The theorem has been Mathematical proof, proved numerous times by many different methods – possibly the most for any mathematical theorem. The proofs are diverse, including both Geometry, geometric proofs and Algebra, algebraic proofs, with some dating back thousands of years. When Euclidean space is represented by a Cartesian coordinate system in analytic geometry, Euclidean distance satisfies th ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Huainanzi
The ''Huainanzi'' is an ancient Chinese text made up of essays from scholarly debates held at the court of Liu An, Prince of Huainan, before 139 BCE. Compiled as a handbook for an enlightened sovereign and his court, the work attempts to define the conditions for a perfect socio-political order, derived mainly from a perfect ruler. With a notable Zhuangzi (book), Zhuangzi 'Taoist' influence, including Chinese folk religion, Chinese folk theories of yin and yang and Wuxing (Chinese philosophy), Wu Xing, the ''Huainanzi'' draws on Taoist, Legalism (Chinese philosophy), Legalist, Confucian, and Mohist concepts, but subverts the latter three in favor of a wu wei, less active ruler, as prominent in the early Han dynasty before the Emperor Wu of Han, Emperor Wu. The early Han authors of the Huainanzi likely did not yet call themselves Taoist, and differ from Taoism as later understood. But K.C. Hsiao and the work's modern translators still considered it a 'principle' example of Han ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Tian Yuan Shu
''Tian yuan shu'' () is a Chinese system of algebra for polynomial equations. Some of the earliest existing writings were created in the 13th century during the Yuan dynasty. However, the tianyuanshu method was known much earlier, in the Song dynasty and possibly before. History The Tianyuanshu was explained in the writings of Zhu Shijie (''Jade Mirror of the Four Unknowns'') and Li Zhi (mathematician), Li Zhi (''Ceyuan haijing''), two Chinese mathematicians during the Mongol Yuan dynasty. However, after the Ming overthrew the Mongol Yuan, Zhu and Li's mathematical works went into disuse as the Ming literati became suspicious of knowledge imported from Mongol Yuan times. Only recently, with the advent of modern mathematics in China, has the tianyuanshu been re-deciphered. Meanwhile, ''tian yuan shu'' arrived in Japan, where it is called ''tengen-jutsu''. Zhu's text ''Zhu Shijie#Suanxue qimeng, Suanxue qimeng'' was deciphered and was important in the development of Japanese ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Yuan Dynasty
The Yuan dynasty ( ; zh, c=元朝, p=Yuáncháo), officially the Great Yuan (; Mongolian language, Mongolian: , , literally 'Great Yuan State'), was a Mongol-led imperial dynasty of China and a successor state to the Mongol Empire after Division of the Mongol Empire, its division. It was established by Kublai (Emperor Shizu or Setsen Khan), the fifth khagan-emperor of the Mongol Empire from the Borjigin clan, and lasted from 1271 to 1368. In Chinese history, the Yuan dynasty followed the Song dynasty and preceded the Ming dynasty. Although Genghis Khan's enthronement as Khagan in 1206 was described in Chinese language, Chinese as the Han Chinese, Han-style title of Emperor of China, Emperor and the Mongol Empire had ruled territories including modern-day northern China for decades, it was not until 1271 that Kublai Khan officially proclaimed the dynasty in the traditional Han style, and the conquest was not complete until 1279 when the Southern Song dynasty was defeated in t ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Linear Algebra
Linear algebra is the branch of mathematics concerning linear equations such as :a_1x_1+\cdots +a_nx_n=b, linear maps such as :(x_1, \ldots, x_n) \mapsto a_1x_1+\cdots +a_nx_n, and their representations in vector spaces and through matrix (mathematics), matrices. Linear algebra is central to almost all areas of mathematics. For instance, linear algebra is fundamental in modern presentations of geometry, including for defining basic objects such as line (geometry), lines, plane (geometry), planes and rotation (mathematics), rotations. Also, functional analysis, a branch of mathematical analysis, may be viewed as the application of linear algebra to Space of functions, function spaces. Linear algebra is also used in most sciences and fields of engineering because it allows mathematical model, modeling many natural phenomena, and computing efficiently with such models. For nonlinear systems, which cannot be modeled with linear algebra, it is often used for dealing with first-order a ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Horner's Method
In mathematics and computer science, Horner's method (or Horner's scheme) is an algorithm for polynomial evaluation. Although named after William George Horner, this method is much older, as it has been attributed to Joseph-Louis Lagrange by Horner himself, and can be traced back many hundreds of years to Chinese and Persian mathematicians. After the introduction of computers, this algorithm became fundamental for computing efficiently with polynomials. The algorithm is based on Horner's rule, in which a polynomial is written in ''nested form'': \begin &a_0 + a_1x + a_2x^2 + a_3x^3 + \cdots + a_nx^n \\ = &a_0 + x \bigg(a_1 + x \Big(a_2 + x \big(a_3 + \cdots + x(a_ + x \, a_n) \cdots \big) \Big) \bigg). \end This allows the evaluation of a polynomial of degree with only n multiplications and n additions. This is optimal, since there are polynomials of degree that cannot be evaluated with fewer arithmetic operations. Alternatively, Horner's method and also refers to a ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Gaussian Elimination
In mathematics, Gaussian elimination, also known as row reduction, is an algorithm for solving systems of linear equations. It consists of a sequence of row-wise operations performed on the corresponding matrix of coefficients. This method can also be used to compute the rank of a matrix, the determinant of a square matrix, and the inverse of an invertible matrix. The method is named after Carl Friedrich Gauss (1777–1855). To perform row reduction on a matrix, one uses a sequence of elementary row operations to modify the matrix until the lower left-hand corner of the matrix is filled with zeros, as much as possible. There are three types of elementary row operations: * Swapping two rows, * Multiplying a row by a nonzero number, * Adding a multiple of one row to another row. Using these operations, a matrix can always be transformed into an upper triangular matrix (possibly bordered by rows or columns of zeros), and in fact one that is in row echelon form. Once all of the ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Euclidean Division
In arithmetic, Euclidean division – or division with remainder – is the process of dividing one integer (the dividend) by another (the divisor), in a way that produces an integer quotient and a natural number remainder strictly smaller than the absolute value of the divisor. A fundamental property is that the quotient and the remainder exist and are unique, under some conditions. Because of this uniqueness, ''Euclidean division'' is often considered without referring to any method of computation, and without explicitly computing the quotient and the remainder. The methods of computation are called integer division algorithms, the best known of which being long division. Euclidean division, and algorithms to compute it, are fundamental for many questions concerning integers, such as the Euclidean algorithm for finding the greatest common divisor of two integers, and modular arithmetic, for which only remainders are considered. The operation consisting of computing only ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |