|
Bernard Frénicle De Bessy
Bernard Frénicle de Bessy (c. 1604 – 1674), was a French mathematician born in Paris, who wrote numerous mathematical papers, mainly in number theory and combinatorics. He is best remembered for , a treatise on magic squares published posthumously in 1693, in which he described all 880 essentially different normal magic squares of order 4. The Frénicle standard form, a standard representation of magic squares, is named after him. He solved many problems created by Fermat and also discovered the cube property of the number 1729 (Ramanujan number), later referred to as a taxicab number. He is also remembered for his treatise ''Traité des triangles rectangles en nombres'' published (posthumously) in 1676 and reprinted in 1729. Bessy was a member of many of the scientific circles of his day, including the French Academy of Sciences, and corresponded with many prominent mathematicians, such as Mersenne and Pascal. Bessy was also particularly close to Fermat, Descartes and Wallis, ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Mathematician
A mathematician is someone who uses an extensive knowledge of mathematics in their work, typically to solve mathematical problems. Mathematicians are concerned with numbers, data, quantity, structure, space, models, and change. History One of the earliest known mathematicians were Thales of Miletus (c. 624–c.546 BC); he has been hailed as the first true mathematician and the first known individual to whom a mathematical discovery has been attributed. He is credited with the first use of deductive reasoning applied to geometry, by deriving four corollaries to Thales' Theorem. The number of known mathematicians grew when Pythagoras of Samos (c. 582–c. 507 BC) established the Pythagorean School, whose doctrine it was that mathematics ruled the universe and whose motto was "All is number". It was the Pythagoreans who coined the term "mathematics", and with whom the study of mathematics for its own sake begins. The first woman mathematician recorded by history was Hypati ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Théophile Pépin
Jean François Théophile Pépin (14 May 1826 – 3 April 1904) was a French mathematician. Born in Cluses, Haute-Savoie, he became a Jesuit in 1846, and from 1850 to 1856 and from 1862 to 1871 he was Professor of Mathematics at various Jesuit colleges. He was appointed Professor of Canon Law in 1873, moving to Rome in 1880. He died in Lyon at the age of 77. His work centred on number theory. In 1876 he found a new proof of Fermat's Last Theorem for ''n'' = 7, and in 1880 he published the first general solution to Frénicle de Bessy's problem :''x''2 + ''y''2 = ''z''2, ''x''2 = ''u''2 + ''v''2, ''x'' − ''y'' = ''u'' − ''v''. He also gave his name to Pépin's test, a test of primality for Fermat number In mathematics, a Fermat number, named after Pierre de Fermat, who first studied them, is a positive integer of the form :F_ = 2^ + 1, where ''n'' is a non-negative integer. The first few Fermat numbers are: : 3, ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
17th-century French People
The 17th century lasted from January 1, 1601 ( MDCI), to December 31, 1700 ( MDCC). It falls into the early modern period of Europe and in that continent (whose impact on the world was increasing) was characterized by the Baroque cultural movement, the latter part of the Spanish Golden Age, the Dutch Golden Age, the French ''Grand Siècle'' dominated by Louis XIV, the Scientific Revolution, the world's first public company and megacorporation known as the Dutch East India Company, and according to some historians, the General Crisis. From the mid-17th century, European politics were increasingly dominated by the Kingdom of France of Louis XIV, where royal power was solidified domestically in the civil war of the Fronde. The semi-feudal territorial French nobility was weakened and subjugated to the power of an absolute monarchy through the reinvention of the Palace of Versailles from a hunting lodge to a gilded prison, in which a greatly expanded royal court could be more easily k ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
1675 Deaths
Events January–March * January 5 – Franco-Dutch War – Battle of Turckheim: The French defeat Austria and Brandenburg. * January 29 – John Sassamon, an English-educated Native American Christian, dies at Assawampsett Pond, an event which will trigger a year-long war between the English American colonists of New England, and the Algonquian Native American tribes. * February 4 – The Italian opera ''La divisione del mondo'', by Giovanni Legrenzi, is performed for the first time, premiering in Venice at the Teatro San Luca. The new opera, telling the story of the "division of the world" after the battle between the Gods of Olympus and the Titans, becomes known for its elaborate and expensive sets, machinery, and special effects and is revived 325 years later in the year 2000. * February 6 – Nicolò Sagredo is elected as the new Doge of Venice and leader of the Venetian Republic, replacing Domenico II Contarini, who had died 10 days ea ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
1605 Births
Sixteen or 16 may refer to: *16 (number), the natural number following 15 and preceding 17 *one of the years 16 BC, AD 16, 1916, 2016 Films * '' Pathinaaru'' or ''Sixteen'', a 2010 Tamil film * ''Sixteen'' (1943 film), a 1943 Argentine film directed by Carlos Hugo Christensen * ''Sixteen'' (2013 Indian film), a 2013 Hindi film * ''Sixteen'' (2013 British film), a 2013 British film by director Rob Brown Music *The Sixteen, an English choir * 16 (band), a sludge metal band * Sixteen (Polish band), a Polish band Albums * ''16'' (Robin album), a 2014 album by Robin * 16 (Madhouse album), a 1987 album by Madhouse * ''Sixteen'' (album), a 1983 album by Stacy Lattisaw *''Sixteen'' , a 2005 album by Shook Ones * ''16'', a 2020 album by Wejdene Songs * "16" (Sneaky Sound System song), 2009 * "Sixteen" (Thomas Rhett song), 2017 * "Sixteen" (Ellie Goulding song), 2019 *"16", by Craig David from ''Following My Intuition'', 2016 *"16", by Green Day from '' 39/Smooth'', 1990 *"16", ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Rouse History Of Mathematics
Rouse may refer to: Places * Rouse, California, United States, a census-designated place * Rouse, Wisconsin, United States, an unincorporated community * Rouses Point, New York, United States, a village * Rouse Islands, Antarctica * Cape Rouse, Antarctica People * Rouse (surname) * Rouse Simmons (Wisconsin politician) (1832–1897), American politician and businessman Other uses * The Rouse, a military bugle call * Rouse Baronets, an extinct baronetcy in the Baronetage of England * Rouse High School, Leander, Texas, United States * Rouse Ranch, Holt County, Nebraska, United States * The Rouse Company, an American real estate developer See also * Rouse model in polymer physics * Rouse number, a non-dimensional number in fluid dynamics * Rouse Rocks (other) * Rouses, a supermarket chain in Louisiana and Mississippi * Rousse Ruse (also transliterated as Rousse, Russe; bg, Русе ) is the fifth largest city in Bulgaria. Ruse is in the northeastern part of the count ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Public Domain
The public domain (PD) consists of all the creative work A creative work is a manifestation of creative effort including fine artwork (sculpture, paintings, drawing, sketching, performance art), dance, writing (literature), filmmaking, and composition. Legal definitions Creative works require a cre ... to which no exclusive intellectual property rights apply. Those rights may have expired, been forfeited, expressly waived, or may be inapplicable. Because those rights have expired, anyone can legally use or reference those works without permission. As examples, the works of William Shakespeare, Ludwig van Beethoven, Leonardo da Vinci and Georges Méliès are in the public domain either by virtue of their having been created before copyright existed, or by their copyright term having expired. Some works are not covered by a country's copyright laws, and are therefore in the public domain; for example, in the United States, items excluded from copyright include the for ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Deductive Reasoning
Deductive reasoning is the mental process of drawing deductive inferences. An inference is deductively valid if its conclusion follows logically from its premises, i.e. if it is impossible for the premises to be true and the conclusion to be false. For example, the inference from the premises "all men are mortal" and "Socrates is a man" to the conclusion "Socrates is mortal" is deductively valid. An argument is ''sound'' if it is ''valid'' and all its premises are true. Some theorists define deduction in terms of the intentions of the author: they have to intend for the premises to offer deductive support to the conclusion. With the help of this modification, it is possible to distinguish valid from invalid deductive reasoning: it is invalid if the author's belief about the deductive support is false, but even invalid deductive reasoning is a form of deductive reasoning. Psychology is interested in deductive reasoning as a psychological process, i.e. how people ''actually'' draw ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Axioms
An axiom, postulate, or assumption is a statement that is taken to be true, to serve as a premise or starting point for further reasoning and arguments. The word comes from the Ancient Greek word (), meaning 'that which is thought worthy or fit' or 'that which commends itself as evident'. The term has subtle differences in definition when used in the context of different fields of study. As defined in classic philosophy, an axiom is a statement that is so evident or well-established, that it is accepted without controversy or question. As used in modern logic, an axiom is a premise or starting point for reasoning. As used in mathematics, the term ''axiom'' is used in two related but distinguishable senses: "logical axioms" and "non-logical axioms". Logical axioms are usually statements that are taken to be true within the system of logic they define and are often shown in symbolic form (e.g., (''A'' and ''B'') implies ''A''), while non-logical axioms (e.g., ) are actually ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Euclid's Elements
The ''Elements'' ( grc, Στοιχεῖα ''Stoikheîa'') is a mathematical treatise consisting of 13 books attributed to the ancient Greek mathematician Euclid in Alexandria, Ptolemaic Egypt 300 BC. It is a collection of definitions, postulates, propositions (theorems and constructions), and mathematical proofs of the propositions. The books cover plane and solid Euclidean geometry, elementary number theory, and incommensurable lines. ''Elements'' is the oldest extant large-scale deductive treatment of mathematics. It has proven instrumental in the development of logic and modern science, and its logical rigor was not surpassed until the 19th century. Euclid's ''Elements'' has been referred to as the most successful and influential textbook ever written. It was one of the very earliest mathematical works to be printed after the invention of the printing press and has been estimated to be second only to the Bible in the number of editions published since the first printing i ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Perfect Squares
In mathematics, a square number or perfect square is an integer that is the square of an integer; in other words, it is the product of some integer with itself. For example, 9 is a square number, since it equals and can be written as . The usual notation for the square of a number is not the product , but the equivalent exponentiation , usually pronounced as " squared". The name ''square'' number comes from the name of the shape. The unit of area is defined as the area of a unit square (). Hence, a square with side length has area . If a square number is represented by ''n'' points, the points can be arranged in rows as a square each side of which has the same number of points as the square root of ''n''; thus, square numbers are a type of figurate numbers (other examples being cube numbers and triangular numbers). Square numbers are non-negative. A non-negative integer is a square number when its square root is again an integer. For example, \sqrt = 3, so 9 is a squ ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
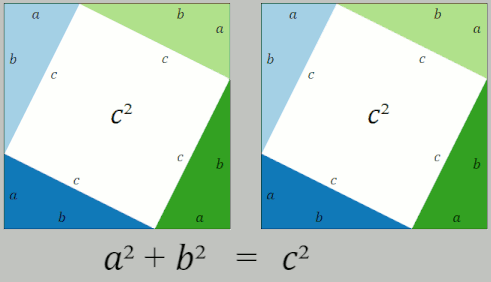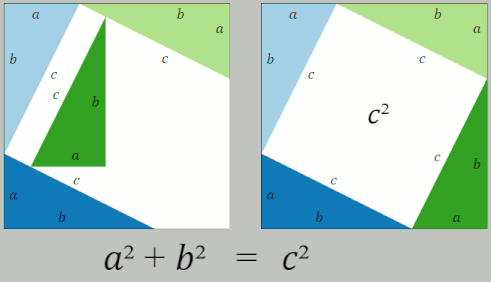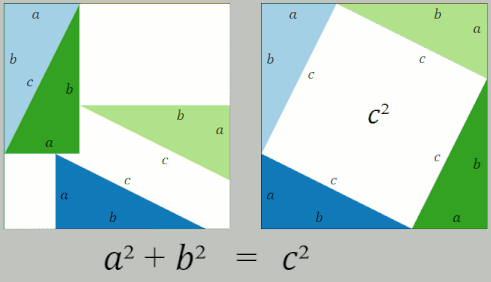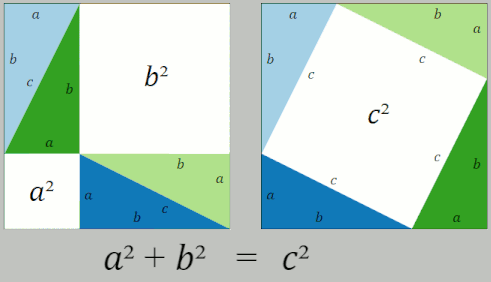
Pythagorean Theorem
In mathematics, the Pythagorean theorem or Pythagoras' theorem is a fundamental relation in Euclidean geometry between the three sides of a right triangle. It states that the area of the square whose side is the hypotenuse (the side opposite the right angle) is equal to the sum of the areas of the squares on the other two sides. This theorem can be written as an equation relating the lengths of the sides ''a'', ''b'' and the hypotenuse ''c'', often called the Pythagorean equation: :a^2 + b^2 = c^2 , The theorem is named for the Greek philosopher Pythagoras, born around 570 BC. The theorem has been proven numerous times by many different methods – possibly the most for any mathematical theorem. The proofs are diverse, including both geometric proofs and algebraic proofs, with some dating back thousands of years. When Euclidean space is represented by a Cartesian coordinate system in analytic geometry, Euclidean distance satisfies the Pythagorean relation: the squared dist ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |