|
Argand Burner
Argand may refer to: * Aimé Argand (1755 – 1803), Swiss physicist and chemist and inventor of the argand lamp * Émile Argand (1879 – 1940), Swiss geologist * Jean-Robert Argand (1768 – 1822), French amateur mathematician ** Argand diagram In mathematics, the complex plane is the plane formed by the complex numbers, with a Cartesian coordinate system such that the -axis, called the real axis, is formed by the real numbers, and the -axis, called the imaginary axis, is formed by the ... ** Argand plane * Luc Argand (1948 - ), Swiss lawyer. {{disambig ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Aimé Argand
François-Pierre-Amédée Argand, known as Ami Argand (5 July 1750 – 14 or 24 October 1803) was a Genevan physicist and chemist. He invented the Argand lamp, a great improvement on the traditional oil lamp. Early years Francois-Pierre-Amédée Argand was born in Geneva, Republic of Geneva, the ninth of ten children. His father was a watchmaker, who intended for him to enter the clergy. However, he had an aptitude more for science, and became a pupil of the noted botanist and meteorologist Horace-Bénédict de Saussure. He published several scientific papers on meteorological subjects while in Paris in his late twenties. He took a teaching post in chemistry and developed some ideas for improving the distillation of wine into brandy, and, with his brother, successfully built a large distillery. The invention During this period, in 1780, he started to invent improvements on the conventional oil lamp. The basic idea was to have a cylindrical wick which air could flow through and ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
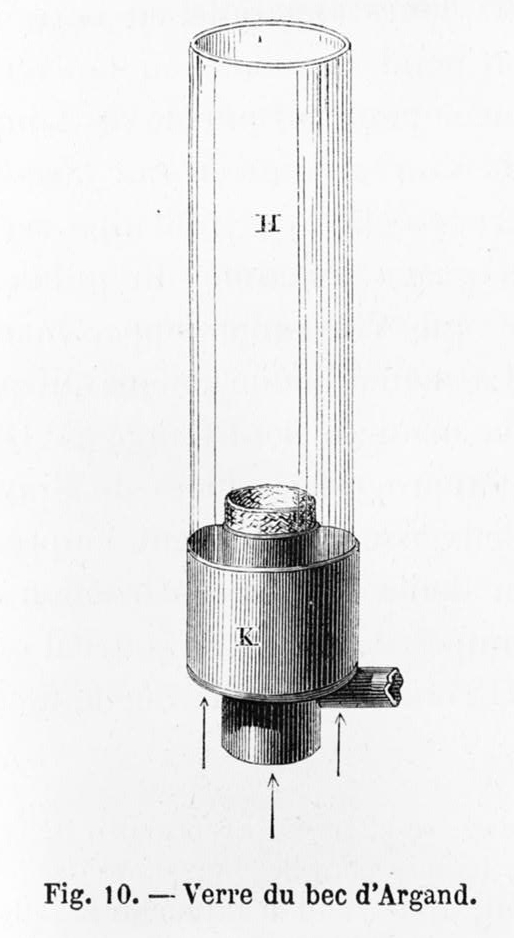
Argand Lamp
The Argand lamp is a type of oil lamp invented in 1780 by Aimé Argand. Its output is 6 to 10 candelas, brighter than that of earlier lamps. Its more complete combustion of the candle wick and oil than in other lamps required much less frequent trimming of the wick. In France, the lamp is called "Quinquet", after Antoine-Arnoult Quinquet, a pharmacist in Paris, who used the idea originated by Argand and popularized it in France. Quinquet sometimes is credited with the addition of the glass chimney to the lamp. Design The Argand lamp had a sleeve-shaped wick mounted so that air can pass both through the center of the wick and also around the outside of the wick before being drawn into a cylindrical chimney which steadies the flame and improves the flow of air. Early models used ground glass which was sometimes tinted around the wick. An Argand lamp used whale oil, seal oil, colza, olive oil or other vegetable oil as fuel which was supplied by a gravity feed from a reservoir mou ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Émile Argand
Émile Argand (6 January 1879 – 14 September 1940) was a Swiss geologist. He was born in Eaux-Vives near Geneva. He attended vocational school in Geneva then worked as a draftsman. He studied anatomy in Paris, but gave up medicine to pursue his interest in geology. He was an early proponent of Alfred Wegener's theory of continental drift, viewing plate tectonics and continental collisions as the best explanation for the formation of the Alps. He is also noted for his application of the theory of tectonics to the continent of Asia. He founded the Geological Institute of Neuchâtel, Switzerland. Awards and honors * 1913 Spendiarov Prize * 1926 Marcel Benoist Prize * A region of wrinkle ridges on the Moon was named ''Dorsa Argand'' after him. * There is a road named "Rue Emile-Argand" at the University of Neuchâtel The University of Neuchâtel (UniNE) is a French-speaking university based in Neuchâtel, Switzerland. The university has four faculties (schools) and more than ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Jean-Robert Argand
Jean-Robert Argand (, , ; July 18, 1768 – August 13, 1822) was an amateur mathematician. In 1806, while managing a bookstore in Paris, he published the idea of geometrical interpretation of complex numbers known as the Argand diagram and is known for the first rigorous mathematical proof, proof of the Fundamental Theorem of Algebra. Life Jean-Robert Argand was born in Geneva, then Republic of Geneva, to Jacques Argand and Eve Carnac. His background and education are mostly unknown. Since his knowledge of mathematics was self-taught and he did not belong to any mathematical organizations, he likely pursued mathematics as a hobby rather than a profession. Argand moved to Paris in 1806 with his family and, when managing a bookshop there, privately published his ''Essai sur une manière de représenter les quantités imaginaires dans les constructions géométriques'' (Essay on a method of representing complex number, imaginary quantities). In 1813, it was republished in the Frenc ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Argand Diagram
In mathematics, the complex plane is the plane formed by the complex numbers, with a Cartesian coordinate system such that the -axis, called the real axis, is formed by the real numbers, and the -axis, called the imaginary axis, is formed by the imaginary numbers. The complex plane allows a geometric interpretation of complex numbers. Under addition, they add like vectors. The multiplication of two complex numbers can be expressed more easily in polar coordinates—the magnitude or ''modulus'' of the product is the product of the two absolute values, or moduli, and the angle or ''argument'' of the product is the sum of the two angles, or arguments. In particular, multiplication by a complex number of modulus 1 acts as a rotation. The complex plane is sometimes known as the Argand plane or Gauss plane. Notational conventions Complex numbers In complex analysis, the complex numbers are customarily represented by the symbol ''z'', which can be separated into its real (''x'') and im ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Complex Plane
In mathematics, the complex plane is the plane formed by the complex numbers, with a Cartesian coordinate system such that the -axis, called the real axis, is formed by the real numbers, and the -axis, called the imaginary axis, is formed by the imaginary numbers. The complex plane allows a geometric interpretation of complex numbers. Under addition, they add like vectors. The multiplication of two complex numbers can be expressed more easily in polar coordinates—the magnitude or ''modulus'' of the product is the product of the two absolute values, or moduli, and the angle or ''argument'' of the product is the sum of the two angles, or arguments. In particular, multiplication by a complex number of modulus 1 acts as a rotation. The complex plane is sometimes known as the Argand plane or Gauss plane. Notational conventions Complex numbers In complex analysis, the complex numbers are customarily represented by the symbol ''z'', which can be separated into its real (''x'') and ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |